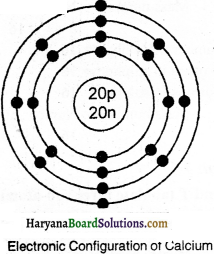
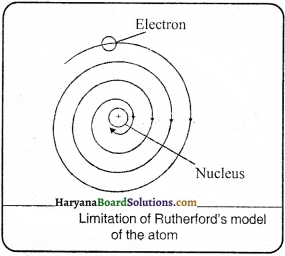
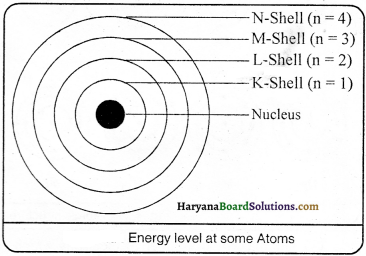
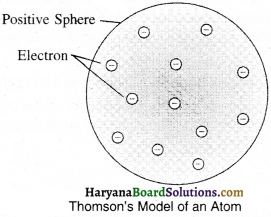
Haryana State Board HBSE 9th Class Hindi Solutions Hindi Rachana Nibanbh-Lekhan निबंध-लेखन Exercise Questions and Answers.
Haryana Board 9th Class Hindi Rachana निबंध-लेखन
निबंध-लेखन
निबंध-लेखन की पद्धति
गद्य अच्छे लेखकों की कसौटी है परंतु निबंध गद्य की कसौटी है। इसका कारण यह है कि निबंध में विचारों और भावों की श्रृंखला होनी चाहिए। निबंध का प्रत्येक वाक्य विषय-वस्तु से संबद्ध हो, यह भी ज़रूरी है। अस्तु निबंध लिखना एक कला है। निबंध-लेखक किसी दिए हुए विषय पर अपने विचारों को सुव्यवस्थित ढंग से प्रस्तुत करता है।
(क) विषय का चयन:
प्रश्न-पत्र में निबंध-लेखन के लिए अनेक विषय होते हैं। अतः सबसे पहली समस्या यह उत्पन्न होती है कि कौन-सा विषय चुना जाए और कौन-सा छोड़ा जाए। हमें केवल वही विषय चुनना चाहिए जिसके बारे में हमें पूरा ज्ञान हो। यदि हम एक बार विषय चुनकर लिखना आरंभ कर दें और फिर उसे काटकर नया विषय चुनेंगे तो इससे दो हानियाँ होंगी। पहली बात तो यह कि हमारा समय नष्ट होगा, दूसरा, हमारे आत्मविश्वास को भी ठेस पहुँचेगी। अतः निबंध का श्रीगणेश करने से पूर्व हमें उसी विषय का चयन करना चाहिए जिसके बारे में हमें पूर्ण जानकारी हो।
(ख) निबंध की रूपरेखा:
प्रायः हम विषय-चयन के पश्चात निबंध लिखना आरंभ कर देते हैं, यह पद्धति सही नहीं है। हमें चुने गए विषय के बारे में दो-चार मिनट सोचकर उसकी अस्थाई रूपरेखा बना लेनी चाहिए। उदाहरण के रूप में, हम ‘आदर्श विद्यार्थी’ का निबंध लेते हैं तो इसके बारे में निम्नोक्त विचार दिए जा सकते हैं-
‘विद्यार्थी’ शब्द का अर्थ क्या है, विद्यार्थी जीवन का महत्त्व क्या है अथवा प्राचीन काल में विद्यार्थी जीवन क्या था ? विद्यार्थी के क्या-क्या गुण होने चाहिएँ ? विद्यार्थी और अनुशासन अथवा विद्यार्थी के जीवन में सादे जीवन और उच्च विचारों का क्या महत्त्व है ? तत्पश्चात, विद्यार्थी के जीवन में परिश्रम, संयम और खेल-कूद का क्या स्थान है ? इन सभी प्रश्नों को पूर्वापर क्रम से रखने पर ही हम ‘आदर्श विद्यार्थी’ विषय के प्रति न्याय कर सकेंगे।
(ग) निबंध का श्रीगणेश:
अगर हम निबंध का आरंभ सुचारु ढंग से करेंगे तो परीक्षक पर हमारा प्रभाव अनुकूल रहेगा। अंग्रेज़ी में कहा भी गया है-Well begun is half done. भूमिका बाँधकर हमें सीधा मूल विषय पर आना चाहिए। इस प्रकार, निबंध का आरंभ आकर्षक एवं प्रभावोत्पादक हो जाएगा।
(घ) निबंध का मध्य भाग:
निबंध के मध्य भाग में संबंधित विषय का सांगोपांग विवेचन होना चाहिए। विषय का प्रतिपादन करते समय सर्वाधिक सावधानी की आवश्यकता है। स्थान और सीमा को ध्यान में रखकर ही विषय का प्रतिपादन हो तो अच्छी बात है। मूल विषय से कभी भी भटकना नहीं चाहिए। विषय से मिलती-जुलती बातों अथवा तथ्यों का प्रयोग उसे अधिक स्पष्ट करता है परंतु यह भी ध्यान में रहे कि विषय का मध्य भाग न अधिक लंबा हो और न ही अधिक छोटा।
(ङ) निबंध का अंतिम भाग:
निबंध के आरंभ की तरह उसका अंत भी महत्त्वपूर्ण होता है। निबंध के अंक तो निबंध के अंत में ही दिए जाते हैं क्योंकि परीक्षक अंतिम भाग को ध्यान से पढ़ता है इसलिए निबंध का अंतिम भाग यदि प्रभावपूर्ण नहीं होगा तो अच्छे अंकों की आशा नहीं की जा सकती।
(च) शुद्ध भाषा का प्रयोग:
भाषा की शुद्धता का निबंध में विशेष महत्त्व है। अशद्धियों के अंक अवश्य कटते हैं। परीक्षक अशुद्धियों पर लाल स्याही के निशान लगाता चलता है। निबंध का मूल्यांकन करते समय इन अशुद्धियों की ओर विशेष ध्यान दिया जाता है। जितनी अधिक अशुद्धियाँ होती हैं, उतने ही अंक कट जाते हैं। फिर अशुद्धियाँ परीक्षक पर बुरा प्रभाव डालती हैं। अतः निबंध लिखने के बाद उसे एक बार पुनः पढ़ लेना चाहिए।
संक्षेप में निबंध-लेखन के समय हमें निम्नोक्त बातों का ध्यान रखना चाहिए-
(i) विचारों में सुसम्बद्धता एवं एकसूत्रता हो,
(ii) एक ही शब्द का बार-बार प्रयोग न करें,
(iii) विराम-चिह्नों का सही एवं उचित प्रयोग होना चाहिए,
(iv) एक ही भाव या विचार की आवृत्ति नहीं होनी चाहिए,
(v) वाक्य छोटे-छोटे और सरल हों,
(vi) कठिन और संस्कृतनिष्ठ शब्दों का अधिक प्रयोग न करें,
(vii) अलंकृत भाषा का यथासंभव प्रयोग न हो,
(viii) नए विचार के लिए नए पैरे का प्रयोग करें,
(ix) यथासंभव उपशीर्षकों का प्रयोग करें, लेकिन ये सर्वथा उचित होने चाहिएँ।

1. मेरे सपनों का भारत
भूमिका: भारत एक महान् देश है। इसकी संस्कृति सबसे प्राचीन है। राजा दुष्यंत और शकुन्तला के पुत्र भरत के नाम पर इस देश का नाम भारत पड़ा। संसार में मेरे देश का बड़ा गौरव है।
1. भौगोलिक स्थिति व जनसंख्या:
भारत एक विशाल देश है। जनसंख्या की दृष्टि से यह विश्व भर में दूसरे स्थान पर है। इसकी वर्तमान जनसंख्या सवा सौ करोड़ से भी अधिक है। भारत के उत्तर में हिमालय, दक्षिण में लंका और हिंद महासागर हैं। इसमें अनेक प्रकार के पर्वत, नदियाँ और मरुस्थल हैं। भौगोलिक दृष्टि से यहाँ अनेक विविधताएँ हैं।
2. सांस्कृतिक एकता:
भारत में विभिन्न धर्मों, जातियों और संप्रदायों के लोग रहते हैं, किंतु फिर भी यहाँ सांस्कृतिक एकता विद्यमान है। कश्मीर से कन्याकुमारी तक हमारा देश एक है। यहाँ अनेक महत्त्वपूर्ण स्थान हैं। हरिद्वार, काशी, कुरुक्षेत्र, मथुरा, गया, जगन्नाथपुरी, द्वारिका आदि यहाँ के प्रसिद्ध तीर्थ हैं। शिमला, मसूरी, नैनीताल, मनाली, दार्जिलिंग आदि सुंदर पर्वतीय स्थान हैं। मुम्बई, कोलकाता, चेन्नई आदि यहाँ के प्रमुख औद्योगिक केंद्र हैं। नई दिल्ली हमारे देश की राजधानी है।।
3. कृषि-प्रधान:
भारत कृषि-प्रधान देश है। भारत में लगभग छह लाख गाँव बसते हैं। इसकी लगभग अस्सी प्रतिशत जनता गाँवों में रहती है। यहाँ गेहूँ, मक्का, बाजरा, ज्वार, चना, धान, गन्ना आदि की फसलें होती हैं। अन्न की दृष्टि से अब हम आत्म-निर्भर हैं।
4. उन्नतिशील:
भारतवर्ष कई सदियों की गुलामी झेलने के बाद सन् 1947 में स्वतंत्र हुआ। स्वतंत्रता मिलने के तुरंत पश्चात् ही यहाँ बहुमुखी उन्नति के लिए प्रयत्न आरंभ हो गए थे। स्वतंत्रता प्राप्ति के बाद यहाँ अनेक प्रकार के कारखाने लगाए गए जिनमें आशातीत उन्नति हुई है। यहाँ अनेक प्रकार की योजनाएँ आरंभ हुईं। पंचवर्षीय योजनाओं के अंतर्गत हर क्षेत्र में भरपूर उन्नति हुई। जिन वस्तुओं को हम विदेशों से मँगवाते थे, आज उन्हीं देशों को अनेक वस्तुएँ बनाकर भेजी जा रही हैं। विज्ञान के क्षेत्र में यहाँ बहुत उन्नति हुई है। परमाणु और उपग्रह के क्षेत्र में भी हम किसी से पीछे नहीं हैं।
5. भारत के महापुरुष:
भारत महापुरुषों की जन्म-स्थली है। राम, कृष्ण, बुद्ध, महावीर, नानक आदि महापुरुष इसी देश में हुए हैं। महाराणा प्रताप, शिवाजी और गुरु गोबिंद सिंह जी इसी देश की शोभा थे। दयानंद, विवेकानंद, रामतीर्थ, तिलक, गांधी, सुभाष, चंद्रशेखर आजाद, भगतसिंह आदि इसी धरती का श्रृंगार थे। वाल्मीकि, व्यास, कालिदास, तुलसीदास, सूरदास, कबीर आदि की वाणी यहीं गूंजती रही। भारत ने ही वेदों के ज्ञान से संसार को मानवता का रास्ता दिखाया।
6. महान्-प्रजातंत्र:
आज भारत में प्रजातंत्र है। यह एक धर्म-निरपेक्ष देश है। भारत संसार का सबसे बड़ा प्रजातंत्र गणराज्य है। हमारा अपना संविधान है। संविधान में प्रत्येक नागरिक को समान अधिकार दिए गए हैं। भारतवासी सदा से शांतिप्रिय रहे हैं, किंतु यदि कोई हमें डराने या धमकाने का प्रयत्न करे तो यहाँ के रणबांकुरे उसके दाँत खट्टे कर डालते हैं।
निष्कर्ष:
तिरंगा हमारे देश का राष्ट्रीय ध्वज है। यहाँ प्रकृति की विशेष छटा देखने को मिलती है। यहाँ हर दो मास बाद ऋतु बदलती रहती है। कविवर जयशंकर प्रसाद ने ठीक ही लिखा है-“अरुण यह मधुमय देश हमारा।”

2. हमारा विद्यालय
भूमिका:
विद्यालय वह मंदिर है जहाँ विद्या बाँटी जाती है। हमारे विद्यालय का नाम राजकीय वरिष्ठ माध्यमिक विद्यालय, करनाल है। यह रेलवे रोड पर स्थित है। इसमें छठी कक्षा से लेकर बारहवीं कक्षा तक शिक्षा दी जाती है। इसमें लगभग बारह सौ विद्यार्थी हैं। हमारे विद्यालय में लड़के-लड़कियाँ साथ-साथ पढ़ते हैं। यह नगर का सबसे बड़ा विद्यालय है।
1. विद्यालय की स्थिति:
हमारे विद्यालय का संपूर्ण भवन नव-निर्मित है। इसमें सभी आधुनिक साधन हैं। इसमें विभिन्न खेलों के क्रीड़ा-स्थल हैं जिसमें हम नित्य-प्रति खेलते हैं। इसमें एक वाटिका भी है जिसमें अनेक प्रकार के फूल खिले रहते हैं। विद्यालय का भवन पूर्णतः हवादार है।
हमारे विद्यालय के भवन में तीस श्रेणी-कक्ष हैं। सभी कमरों में बिजली के पंखे लगे हुए हैं। इनके अतिरिक्त एक सभा भवन भी है। शनिवार को ‘बाल सभा’ एवं अन्य उत्सव इसी सभा भवन में होते हैं। महीने में एक बार फिल्म भी दिखाई जाती है। प्रधानाचार्य महोदय का कमरा, कार्यालय एवं स्टाफ रूम सभी सुसज्जित हैं।
2. अध्यापक गण:
हमारे विद्यालय में 45 अध्यापक/अध्यापिकाएँ हैं। सभी अध्यापक बहुत योग्य हैं। वे हमें अत्यंत परिश्रमं और लगन से पढ़ाते हैं। यही कारण है कि हमारे विद्यालय की वार्षिक परीक्षा का परिणाम प्रत्येक वर्ष अति उत्तम रहता है। पिछले वर्ष हमारे विद्यालय की आठवीं कक्षा का विद्यार्थी राजकुमार राज्य-भर में प्रथम रहा है। इसके साथ-साथ दसवीं तथा बारहवीं कक्षाओं के परीक्षा परिणाम भी प्रतिवर्ष बहुत अच्छे रहते हैं। बारहवीं कक्षा की कुमारी नीलम राज्य भर में प्रथम रही थी।
3. अन्य प्रमुख विशेषताएँ:
हमारे विद्यालय की मुख्य विशेषता यह है कि इसमें किसी भी छात्र को शारीरिक दंड नहीं दिया जाता। अध्यापक बड़े प्यार से पढ़ाते हैं। हमारे विद्यालय में शिक्षा के साथ-साथ खेलों की ओर भी विशेष ध्यान दिया जाता है। गत वर्ष हमारी हॉकी टीम जिले में प्रथम रही थी। नैतिक शिक्षा पर विशेष बल दिया जाता है। हमारे विद्यालय में हर वर्ष पुरस्कार वितरण समारोह का आयोजन भी किया जाता है। इस समारोह में विभिन्न गतिविधियों में योग्यता प्राप्त विद्यार्थियों को पुरस्कार दिए जाते हैं तथा प्रधानाचार्य द्वारा वार्षिक रिपोर्ट पढ़ी जाती है। मुझे अपने विद्यालय से बड़ा प्यार है। यह वह मंदिर है, जहाँ हमें शिक्षा दी जाती है और जीवन का निर्माण किया जाता है।
4. हमारे प्रधानाचार्य:
हमारे विद्यालय के प्रधानाचार्य का नाम डॉ० विश्वराय सेन हैं। वे बड़े ही योग्य एवं दूरदर्शी हैं। वे स्वयं विद्यालय के परिसर की देखभाल करते हैं। वे ग्यारहवीं तथा बारहवीं कक्षाओं के विद्यार्थियों को स्वयं अंग्रेज़ी पढ़ाते हैं। एक योग्य अध्यापक होने के कारण सभी अध्यापक-अध्यापिकाएँ उनका बड़ा आदर करते हैं। नगर भर में हमारा विद्यालय एक महान् शिक्षा केंद्र के रूप में प्रसिद्ध है।
उपसंहार:
वैसे तो हमारे नगर में 20 से अधिक विद्यालय हैं। वे भवन की दृष्टि से भव्य हो सकते हैं। उनमें छात्रों की संख्या अधिक हो सकती है, किंतु हमारा विद्यालय एक आदर्श विद्यालय है। यहाँ विद्यार्थी को व्यक्तित्व के सर्वांगीण विकास के पूर्ण अवसर प्रदान किए जाते हैं। यहाँ का वातावरण सुखद, शांत और शिक्षाप्रद है। मुझे अपने विद्यालय पर गर्व है।

3. मेरे जीवन का लक्ष्य
भमिका:
मानघ का प्रत्येक कार्य उद्देश्यपूर्ण होता है। एक मुर्ख व्यक्ति भी बिना उद्देश्य के काम नहीं करता। अतः प्रत्येक व्यक्ति कोई-न-कोई लक्ष्य सामने रखकर काम करता है। हमारा जीवन एक यात्रा के समान है। यदि यात्री को मालूम हो कि उसे कहाँ जाना है, तो वह उस लक्ष्य की ओर बढ़ना शुरू कर देता है। परंतु अगर उसे अपने गंतव्य का ज्ञान नहीं है तो उसकी यात्रा निरर्थक बन जाती है। इसी प्रकार से अगर एक विद्यार्थी को यह पता हो कि उसे क्या बनना है तो वह उसी दिशा में प्रयत्न करता है और सफलता भी प्राप्त करता है। इसके विपरीत, उद्देश्यहीन जीवन उसे कहीं नहीं ले जाता।
1. बाल्यावस्था की समस्या:
जब बालक आरंभ में विद्यालय में प्रवेश करता है तो उसके सामने अनेक लक्ष्य होते हैं। वह जैसे-जैसे लोगों के संपर्क में आता है, वैसे-वैसे प्रभाव उस पर पड़ते हैं। कभी तो वह सोचता है कि वह अध्यापक बने या डॉक्टर अथवा इंजीनियर बने तो कभी वह सैनिक बनकर देश की सेवा करना चाहता है। माँ-बाप भी बार-बार कहते रहते हैं कि वे अपने बच्चे को यह बनाएँगे या वह बनाएँगे, लेकिन निर्णय तो विद्यार्थी को स्वयं लेना होता है। अतः माँ-बाप के द्वारा कोई भी लक्ष्य बच्चे पर थोपा नहीं जाना चाहिए। विद्यार्थीकाल मानव के लिए सर्वाधिक महत्त्वपूर्ण है, क्योंकि यह मानव-जीवन की आधार-शिला है। अगर विद्यार्थी इस काल में अपने लिए सही लक्ष्य निर्धारित कर लेता है, तो वह अपने जीवन को सुखी बनाता है और अपने देश तथा समाज के लिए उपयोगी सिद्ध होता है।
2. डॉक्टर बनने का उद्देश्य:
हमारे प्राचीन धार्मिक ग्रंथ भी घोषणा करते हैं कि दो व्यक्तियों का समाज पर अत्यधिक उपकार है-पहला है शिक्षक, जो विद्यार्थियों के जीवन में अज्ञान रूपी अंधकार को दूर करके ज्ञान का दीपक जलाता है और दूसरा है डॉक्टर अथवा वैद्य, जो रोगी का उपचार करके उसे नया जीवन देता है। अतः शिक्षा देना और रोगियों का उपचार करना दोनों ही पावन कर्म हैं और मैंने इनमें से एक पावन कार्य को जीवन का लक्ष्य चुना है। हमारे देश में जो लोग डॉक्टरी की परीक्षा पास करते हैं, वे या तो बड़े-बड़े नगरों में अपने क्लीनिक स्थापित करके धन बटोरना शुरू कर देते हैं या फिर विदेशों की ओर भागते हैं। मैं समझता हूँ कि यह मातृ-भूमि के प्रति अन्याय तथा अपने देश और उसके प्रति विश्वासघात है।
3. डॉक्टर का महत्त्व:
मैं चाहता हूँ कि मैं शहर से दूर गाँव में जाकर अपना एक छोटा-सा अस्पताल स्थापित करूँ ताकि गाँव के लोगों को रोगों से मुक्त होने की सुविधा प्राप्त हो। मैं रोगियों से उतनी ही फीस लूँगा, जिससे कि अस्पताल का काम सुचारू रूप से चल सके। गरीब और अभावग्रस्त रोगियों का मैं मुफ्त इलाज करूँगा। मैं स्वयं एक गाँव का निवासी हूँ। मैंने अनेक बार गाँव के रोगियों को दवाई और इलाज के अभाव में मरते देखा है। अतः मैं चाहता हूँ कि डॉक्टरी द्वारा गाँव के उन लोगों की सेवा की जा सके जो न तो बड़े डॉक्टरों की मोटी फीस दे सकते हैं और न ही मरीज को बड़े-बड़े नगरों में ले जा सकते हैं। इसलिए मैं सोचता हूँ कि ग्रामीण क्षेत्र में डॉक्टरों की अधिकाधिक आवश्यकता है। मैं जानता हूँ कि एक अच्छा और सफल डॉक्टर बनना आसान नहीं है। यह बहुत ही कठिन कार्य है। डॉक्टर के हृदय में मरीजों के प्रति दया, सहानुभूति और करुणा की भावना का होना नितांत आवश्यक है। मैंने इस उद्देश्य की पूर्ति के लिए अभी से प्रयत्न शुरू कर दिए हैं।
मेरे माता-पिता का विश्वास भी मुझे प्राप्त है। वे भी यही चाहते हैं कि मैं डॉक्टर बनूँ। वस्तुतः मैं सही अर्थों में डॉक्टर बनकर उन डॉक्टरों के लिए उदाहरण प्रस्तुत करना चाहता हूँ जो मरीजों से रुपया कमाने के लिए इस व्यवसाय को अपनाए हुए हैं। मैं आशा करता हूँ कि डाक्टर बन कर मैं अपने देश और समाज की सच्ची सेवा कर सकूँगा। ईश्वर से प्रार्थना है कि वह मेरी इस मनोकामना को पूर्ण करे।

4. मेरा हरियाणा
अथवा
मेरा राज्य
देसाँ माँ देस हरियाणा।
जित दूध दही का खाणा ॥
भूमिका:
हरियाणा भारतवर्ष का एक प्रमुख राज्य है। हरियाणा की भूमि वीरों की जननी है। सिकंदर से लेकर तैमूर और बाबर जैसे लगभग दो हजार आक्रमणकारियों का मुकाबला इसी धरती के वीरों ने किया। पानीपत की तीन महान् लड़ाइयाँ इसी राज्य की छाती पर लड़ी गईं थीं। शत्रु के खून से नहाई हुई यह धरती स्वभाव से ही वीरता और बलिदान की प्रेरणा प्रदान करती है।
1. ‘हरियाणा’ नाम की उत्पत्ति:
‘हरियाणा’ नाम की उत्पत्ति के बारे में कई विचारधाराएँ हैं। कई विद्वान इस शब्द के दो पदों का अर्थ ‘हरि’ = ‘कृष्ण’, ‘आणा’ = ‘पधारना’ लगाकर भगवान् श्रीकृष्ण के आगमन के साथ जोड़ते हैं। कई इसे ‘हर’ शंकर का प्रदेश = हर का प्रदेश अर्थात् हरयाणा कहते हैं। कुरुक्षेत्र में भगवान् श्रीकृष्ण का यान अर्थात् रथ चल रहा था, अतः इसे हरियाणा कहते हैं। .
2. हरियाणा की भौगोलिक स्थिति और निर्माण:
हरियाणा प्रांत सरस्वती नदी के पावन तट पर बसा है। इसके पूर्व में भारत की राजधानी नई दिल्ली है। दक्षिण में राजस्थान, उत्तर में हिमाचल प्रदेश और पश्चिम में पंजाब स्थित है। यद्यपि हरियाणा प्रांत अति प्राचीन है, परंतु यह 1 नवंबर, 1966 को अस्तित्व में आया। यहाँ की जनसंख्या 2.54 करोड़ के लगभग है। हरियाणा की राजधानी चंडीगढ़ है। इसमें 90 विधानसभा, 10 लोकसभा क्षेत्र तथा 22 जिले हैं। इस प्रदेश की भाषा हिंदी है।
3. कृषि-प्रधान प्रदेश:
हरियाणा एक कृषि-प्रधान प्रदेश है। यहाँ के लोगों का मुख्य व्यवसाय कृषि है। यहाँ गेहूँ, चना, मोठ, चावल, मूंग, कपास, सरसों, गन्ना आदि फसलें उगाई जाती हैं। यहाँ की गेहूँ एवं चावल की मंडियाँ केवल भारत में ही नहीं अपितु एशिया-भर में प्रसिद्ध हैं। करनाल में स्थित ‘राष्ट्रीय डेरी अनुसंधान संस्थान’ विश्व-भर में प्रसिद्ध है।
4. औद्योगिक केंद्र:
उद्योगों के क्षेत्र में भी हरियाणा सबसे आगे है। सूरजपुर में सीमेंट का उद्योग है। पिंजौर में ‘हिंदुस्तान मशीन टूल्ज’ का बहुत बड़ा कारखाना है। फरीदाबाद में विभिन्न प्रकार के कारखाने हैं। सोनीपत की एटलस साइकिल की फैक्टरी विश्व-भर में प्रसिद्ध है। यमुनानगर में पेपर मिल एवं बर्तनों के कारखाने हैं। पानीपत और करनाल चीनी मिलों के लिए प्रसिद्ध हैं। करनाल में जूतों की कम्पनी विश्व-भर में प्रसिद्ध है।
5. हरियाणा के दर्शनीय धार्मिक स्थान:
हरियाणा में अनेक दर्शनीय एवं धार्मिक स्थल हैं जिनमें से कुरुक्षेत्र, करनाल, पिहोवा, नारनौल, भिवानी आदि दर्शनीय स्थल हैं और सोहना का गर्म पानी का स्रोत, बड़खल झील, कुरुक्षेत्र का बिरला मंदिर आदि प्रमुख हैं।
6. साहित्य एवं संस्कृति का केंद्र:
संस्कृत के महाकवि बाणभट्ट एवं पं० छज्जूराम शास्त्री का जन्म इसी पवित्र धरती पर हुआ। हिंदी क्षेत्र के पं० माधव मिश्र और बालमुकुन्द का जन्म भी इसी पवित्र धरती पर हुआ। संतों में कबीर, निश्चलदास तथा गरीबदास का जन्म भी इसी पवित्र धरती पर हुआ। हरियाणा पर्व-प्रधान प्रदेश है। यहाँ प्रसिद्ध त्योहारों के अतिरिक्त तीज, करवाचौथ, मकर संक्रांति, शिवरात्रि, गूगा नवमी, गोपाष्टमी आदि प्रमुख त्योहार मनाए जाते हैं।
निष्कर्ष:
सार रूप में कहा जा सकता है कि हरियाणा एक नवगठित प्रदेश है, किंतु फिर भी इसने विभिन्न क्षेत्रों में अद्भुत उन्नति की है। हमें हरियाणा निवासी होने का गर्व है। कुछ ही समय में हरियाणा एक आदर्श राज्य के रूप में उभरकर संसार के सामने आएगा। अतः हमें हरियाणा से घनिष्ठ प्रेम है।

5. स्वतंत्रता दिवस
आजादी आ, तुझसे आज दो-दो बातें कर लें।
जो मिटे तेरी डगर में, आज उनको याद कर लें।
भूमिका:
भारतवर्ष सदियों तक विदेशी शासकों के अधीन रहा है। यहाँ की जनता ने एक लम्बे संघर्ष के पश्चात् 15 अगस्त, 1947 को स्वतंत्रता प्राप्त की थी। इस स्वतंत्रता-प्राप्ति के लिए लाखों लोगों ने अपना जीवन बलिदान कर दिया। 15 अगस्त को यह राष्ट्रीय पर्व बड़ी धूम-धाम से मनाया जाता है।
1. स्वतंत्रता का इतिहास:
भारत की स्वतंत्रता का इतिहास बड़ा अनोखा है। अंग्रेज़ भारत में व्यापार करने की इच्छा से आए थे, पर भारत की आंतरिक फूट और कलह से लाभ उठाकर देश के शासक बन बैठे। भारतवासियों ने स्वतंत्रता प्राप्त करने के लिए अनेक प्रयत्न किए। अनेक नेताओं और क्रांतिकारियों ने देश की आजादी के लिए अपना बलिदान दिया। अंत में 15 अगस्त, 1947 को देश का विभाजन करके इसे स्वतंत्रता दे दी गई।
2. पराधीनता का अनुभव:
पराधीनता की पीड़ा को पशु-पक्षी भी जानते हैं। पराधीनता के चंगुल में फंसे हुए पशु-पक्षी भी जंजीर, पिंजरा आदि तोड़कर भागना चाहते हैं। यही दशा उन देशों की होती है जो दासता की बेड़ियों में जकड़े हुए होते हैं।
3. स्वतंत्रता दिवस मनाने की विधि:
स्वतंत्रता दिवस का पूरे राष्ट्र के लिए विशेष महत्त्व है। यह पर्व हमारे मन में प्रसन्नता की लहर उत्पन्न कर देता है। इस पर्व को मनाने के लिए भारतीय जनमानस उत्साहपूर्वक अनेक प्रकार के आयोजन करते हैं; जैसे नाटक, संगीत, नृत्य, भाषण आदि। मकानों, सार्वजनिक कार्यालयों, सरकारी भवनों, दुकानों पर तिरंगे फहराए जाते हैं। इस राष्ट्रीय पर्व का महत्त्व भारत के ग्राम, नगर आदि सभी के लिए एक समान है। 15 अगस्त की प्रभात वेला में दिल्ली में प्रधानमंत्री लालकिले में भाषण देकर देश की दशा पर विचार करते हैं और भारत की स्वतंत्रता के लिए प्राणों की आहुति देने वालों को श्रद्धांजलि भेंट करते हैं।
4. राष्ट्रीय पर्व:
अनेक उत्सवों के साथ आकाशवाणी एवं टेलीविज़न से भी कुछ कार्यक्रम प्रसारित होते हैं। यह राष्ट्रीय त्योहार एवं राजकीय त्योहार न होकर घर-घर की खुशी का त्योहार हो जाता है। स्वतंत्रता दिवस हमारे देश का सर्वोच्च पर्व है। जैसे देश-भर में दीपावली और दशहरा मनाया जाता है उसी प्रकार इस राष्ट्रीय पर्व को भी देश-भर की जनता बड़े उत्साह और खुशी से मनाती है। ऐसे पर्यों के अवसर पर विभिन्न प्रकार के आयोजन करने से देश की जनता में राष्ट्रीयता की भावना उत्पन्न होती है तथा अपने देश की स्वतंत्रता की रक्षा के प्रति जागरूकता आती है।
उपसंहार:
इस पर्व को मनाने से देश की भावी पीढ़ियों के हृदय में देश-भक्ति के बीज अंकुरित होते हैं। यह हमारे जीवन व इतिहास के परिवर्तन का दिन है। भारत के सौभाग्य का दिवस है। यह दिवस इस बात का प्रतीक है कि सब भारतवासियों को एकजुट होकर अपने देश की रक्षा के लिए तैयार रहना है। हमारी स्वतंत्रता ही हमारे अस्तित्व का आधार है। अतः हमें इसे मिलजुल कर प्रेमपूर्वक मनाना चाहिए।
6. राष्ट्रीय एकता
है एक ही देश, एक ही है लक्ष्य हमारा; है एक ध्वज, एक ही संगीत हमारा।
हम एक-सी ही राह, हैं सभी मिलकर बनाते; हम एक हैं सब एकता का नाद गुंजाते ॥
भूमिका:
एक परिवार से लेकर पूरे राष्ट्र और विश्व तक के जीवन में एकता का बहुत महत्त्व है। भारतवर्ष कई राज्यों की इकाइयों का संघ है। इसमें अनेक भाषा बोलने वाले, अनेक धर्मों को मानने वाले और अनेक विचारधाराओं के लोग रहते हैं। ऐसी स्थिति में राष्ट्रीय एकता का महत्त्व और भी बढ़ जाता है, किंतु भारतवर्ष की परंपराएँ और संस्कृति एक है। हमारा संविधान एक है। पूर्ण राष्ट्र माला के विभिन्न फूलों की भांति एकता के सूत्र में बँधा हुआ है। भारत कश्मीर से लेकर कन्याकुमारी तक एक है।
1. राष्ट्रीय एकता का महत्त्व:
एकता का मानव-जीवन में बहुत महत्त्व है। एकता के अभाव में मानव-जीवन बिखर जाता है। उसकी उन्नति के सारे मार्ग बंद हो जाते हैं। यही स्थिति एक राष्ट्र की भी होती है। जब तक संपूर्ण राष्ट्र एक है तब तक कोई भी विदेशी शक्ति देश की ओर आँख उठाकर नहीं देख सकती, परंतु जब देश में कलह या एकता का अभाव दृष्टिगोचर होने लगे, तो देश पतन के गर्त में चला जाता है। पृथ्वीराज तथा जयचंद का उदाहरण द्रष्टव्य है। उनकी आपसी फूट के कारण ही मुहम्मद गौरी को भारत पर आक्रमण करने का साहस हुआ। रावण और विभीषण की आपसी फूट के कारण ही सोने की लंका जलकर राख हो गई। राजपूतों की परस्पर शत्रुता के कारण ही भारत में मुगलों के पाँव जम गए।
2. एकता के मार्ग में बाधाएँ:
भारत की राष्ट्रीय एकता में आज अनेक बाधाएँ उत्पन्न हो गई हैं, लेकिन सबसे बड़ी बाधा तो भाषा की भिन्नता है। भाषा की विभिन्नता के कारण एक ही देश के लोग एक-दूसरे के लिए अजनबी बन जाते हैं। भाषा को लेकर आए दिन दंगे होते हैं। राष्ट्रीय एकता के मार्ग में दूसरी बड़ी बाधा है-सांप्रदायिकता की। हर दिन विभिन्न संप्रदायों के लोगों में झगड़े होते रहते हैं। तीसरी बाधा है-प्रांतीयता और चौथी बाधा है-जातीयता की। इन सब कारणों ने भारत की राष्ट्रीय एकता को कमजोर बना दिया है।
3. राष्ट्रीय एकता के उपाय:
इन बाधाओं को दूर करके राष्ट्रीय एकता को पहले की अपेक्षा मजबूत बनाया जा सकता है। देश के स्वतंत्र होने के पश्चात् उन सब बाधाओं को दूर करने के प्रयास किए गए हैं, जो राष्ट्रीयता के मार्ग में बाधा पहुँचा रही हैं। इसमें संदेह नहीं कि स्वतंत्रता-प्राप्ति के पश्चात् प्रांतीयता, सांप्रदायिकता और जातीयता में वृद्धि हुई है। अतः हमें फिर से उन समस्याओं का समाधान करना होगा, जो हमारी राष्ट्रीय एकता के मार्ग में बाधक सिद्ध हो रही हैं।
4. राष्ट्रीय एकता की रक्षा:
देश के हर नागरिक का कर्तव्य है कि वह राष्ट्रीय एकता को स्थिर रखने के लिए तन-मन और धन से कार्य करे। उसे उन भावनाओं और विचारों से दूर रहना चाहिए जो राष्ट्रीय एकता को हानि पहुँचाते हैं। उसे किसी लालच में आकर अपने देश की एकता को ठेस नहीं पहुँचानी चाहिए। यदि राष्ट्र में रहने वाले हम सभी नागरिक एकता के सूत्र में बँधकर रहेंगे तभी सरक्षित रह सकेंगे।
उपसंहार:
हमें यह नहीं भूलना चाहिए कि जैसे माला में पिरोए हुए हर मोती का महत्त्व तब तक ही होता है जब तक वह माला में रहता है। माला के टूट जाने पर मोती बिखर जाते हैं और उनका कोई महत्त्व नहीं रहता। इसी प्रकार हर नागरिक या राज्य का महत्त्व राष्ट्र के साथ जुड़कर रहने में ही है। यदि हम एक हैं तो सुरक्षित हैं, अन्यथा कोई भी शत्रु हमें गुलाम बना सकता है।

7. स्वदेश प्रेम
भूमिका:
देश-प्रेम वास्तव में महान् एवं पवित्र भावना है। यह वह पवित्र गंगा है जिसमें गोता लगाने से हमारा तन ही नहीं, अपितु मन भी पवित्र हो जाता है। जिस देश की धरती की धूल में लोट-लोट कर हम बड़े हुए हैं उस देश की धरती से, वहाँ के कण-कण से प्रेम होना चाहिए। वास्तव में देखा जाए तो देश-प्रेम ही सबसे बड़ा प्रेम है।
1. देश-प्रेम का अर्थ:
देश-प्रेम का अर्थ है-देश से प्यार अर्थात् देश की धरती पर विद्यमान पहाड़, नदियाँ, जंगल, पशु-पक्षी, वस्तुएँ तथा मनुष्य सभी से प्रेम ही देश-प्रेम कहलाता है। इतना ही नहीं, देश की सभ्यता और संस्कृति के प्रति प्रेम भी देश-प्रेम ही होता है।
2. देश-प्रेम एक स्वाभाविक गुण:
देश-प्रेम मानव का एक स्वाभाविक गुण है। यह गुण तो पक्षियों और पशुओं में भी मिलता है। पक्षी सारा दिन दाने-दाने की तलाश में न जाने कहाँ-कहाँ घूमता है परंतु सायंकाल अपने नीड़ में ही पहुँचता है। उसे भी मातृ-भूमि । से प्रेम होता है।
3. देश-प्रेम क्यों?:
देश की उन्नति के लिए स्वदेश प्रेम परमावश्यक है। जिस देश के निवासी अपने देश के हित में अपना हित, अपने देश के कष्ट में अपना कष्ट तथा अपने देश की समृद्धि में अपनी समृद्धि नहीं समझते हैं। वे देश और राष्ट्र कभी उन्नति नहीं कर सकते। जापान, जर्मनी इत्यादि इसीलिए प्रगतिशील राष्ट्र माने जाते हैं क्योंकि वे प्रत्येक कार्य राष्ट्र के हित में करते हैं।
4. भारतीयों के देश-प्रेम के कुछ उदाहरण:
भारत में ऐसे लोगों की कमी नहीं रही जो अपने देश को अपने जीवन से भी बढ़कर समझते थे। सर्वप्रथम जब लक्ष्मण ने श्रीराम को सोने की लंका में रहने के लिए कहा तो श्रीराम ने उत्तर देते हुए कहा था कि यह तो केवल सोने की लंका है। मुझे तो मेरी जन्म-भूमि स्वर्ग से भी अधिक अच्छी लगती है।
चंद्रगुप्त के नेतृत्व में सभी राजाओं ने मिलकर सिकंदर के विश्व-विजय के स्वप्न को चूर-चूर कर दिया था। इस प्रयत्न के पीछे उनका देश-प्रेम और देश-भक्ति ही कार्यरत थी। मुगल शासनकाल में महाराणा प्रताप, छत्रपति शिवाजी, छत्रसाल और गोबिंद सिंह अत्याचारी शासन के विरुद्ध लड़ते रहे। अंग्रेज़ों के शासनकाल में स्वाधीनता-संग्राम के वीरों ने देश-भक्ति के लिए अपना सर्वस्व न्योछावर कर दिया। भारतीय वीरांगना झाँसी की रानी लक्ष्मीबाई, लाला लाजपतराय, भगत सिंह, चंद्रशेखर आजाद, राजगुरु, सुखदेव, महात्मा गांधी, पं० जवाहरलाल नेहरू, नेताजी सुभाषचंद्र बोस आदि वीरों ने देश के लिए तन-मन-धन न्योछावर कर दिया।
5. देश-प्रेम का संकुचित अर्थ:
आज देश का अर्थ कुछ संकुचित होता जा रहा है। कुछ लोग अपने प्रदेश, अपने धर्म और अपनी भाषा तक ही सीमित रहते हैं। वे संपूर्ण देश से प्रेम नहीं करते। अपने ही देशवासियों को अछूत और पतित कहकर उनके प्रति घृणा व्यक्त करते हैं। यह देश-भक्ति नहीं है। उन्हें याद रखना होगा कि भारत कश्मीर से लेकर कन्याकुमारी तक एक देश है। नेहरू जी का कथन है, “यदि भारत ही न रहा तो हममें से कौन जीवित रह सकता है और यदि भारत जीवित रहता है तो हममें से कौन मर सकता है।”
उपसंहार:
देश के प्रति अपने कर्तव्य का भली-भांति पालन करना ही देश के प्रति निष्ठा और देश-भक्ति है। यदि हर नागरिक अपने कर्तव्य का पालन करे तो यही उनका सच्चा देश-प्रेम या सच्ची देश-भक्ति होगी। इसी भाव को एक कवि ने निम्नलिखित पंक्तियों में व्यक्त किया है-
जिस व्यक्ति के जीवन में सदाचार नहीं,
निर्धन के लिए दिल में भरा प्यार नहीं,
आता ही नहीं देश पे मरना जिसको,
उस नीच को जीने का भी अधिकार नहीं।

8. विज्ञान के लाभ तथा हानियाँ
अथवा
विज्ञान-वरदान या अभिशाप
भूमिका:
आज का युग विज्ञान का युग है। गत एक शताब्दी से विज्ञान एक से बढ़कर एक खोजें करता रहा है। जो बातें कभी कल्पना में सोची जाती थीं विज्ञान ने उन्हें साक्षात् कर दिखाया है। मानव चाँद पर पहुँच गया और इससे भी दूर के ग्रहों पर पहुँचने की तैयारी कर रहा है। विज्ञान के सहयोग से मानव-हृदय तक परिवर्तित होने लगा है।
1. आधुनिक आविष्कार:
परमाणु शक्ति एवं विद्युत के अनुसंधान ने मानव को उन्नति के शिखर पर पहुंचा दिया है। तेज चलने वाले वाहन, समुद्र के वक्ष स्थल को रौंदने वाले जहाज और असीम आसमान में वायु की गति से भी तेज चलने वाले विमान, नक्षत्र लोक तक पहुँचाने वाले रॉकेट प्रकृति पर मानव की विजय के उज्ज्वल उदाहरण हैं। आज तार, टेलीफोन, रेडियो, टेलीविज़न, सिनेमा, ग्रामोफोन आदि ने मानव जीवन में अनेक सुख प्रदान किए हैं।
2. समय व दूरी पर नियंत्रण:
विज्ञान ने मानव को अनेक लाभ पहुँचाए हैं जिनको देखकर कहा जा सकता है कि विज्ञान मनुष्य के लिए एक वरदान है। विज्ञान के कारण मनुष्य ने समय और दूरी पर नियंत्रण कर लिया है। लम्बी यात्रा बस, रेलगाड़ी, हवाई जहाज़ आदि द्वारा कुछ घंटों में पूरी की जा सकती है। मित्रों व संबंधियों तक तार व टेलीफोन द्वारा कुछ ही क्षणों में अपना संदेश भेज सकते हैं।
3. विद्युत एवं पानी:
विद्युत के आविष्कार से हमारी महत्त्वपूर्ण आवश्यकताएँ पूरी हो गई हैं। देश में अनेक कारखाने चलाए जाते हैं। विद्युत का प्रयोग हम घरेलू जीवन में भी करते हैं। बटन दबाते ही पंखा चलने लगता है और बल्ब जगमगा उठते हैं। इसी प्रकार से वैज्ञानिक यंत्रों (बिजली) द्वारा हमें पीने के लिए स्वच्छ पानी मिलता है।
4. स्वास्थ्य एवं कृषि के क्षेत्र में उन्नति:
एक्सरे यंत्र के द्वारा शरीर के भीतरी भाग का चित्र लिया जा सकता है। इसकी सहायता से सैकड़ों रोगों की चिकित्सा की जाती है। प्लेग, चेचक, हैज़ा आदि बीमारियों का उपचार विज्ञान की खोजों से ही सम्भव हुआ है। कृषि के क्षेत्र में विज्ञान ने बहुत उन्नति की है। पुराने हल तथा बैल को त्यागकर आज का किसान ट्रैक्टर से खेती करता है। वह नलकूप, पंप आदि से खेती की सिंचाई करता है।
5. परमाणु शक्ति:
परमाणु शक्ति के आविष्कार ने मानव-जाति को आश्चर्यचकित कर दिया है। परमाणु शक्ति से बड़े-बड़े उद्योग चलाए जाते हैं। परमाणु विस्फोट से पर्वतों को तोड़कर सड़कें बनाई जा सकती हैं तथा नहरें खोदी जा सकती हैं। इस प्रकार उपर्युक्त विज्ञान के लाभों को देखते हुए कहा जा सकता है कि विज्ञान मानव जीवन के लिए वरदान है।
6. विज्ञान की हानियाँ:
विज्ञान से जहाँ मानव को इतने लाभ हुए हैं वहाँ अनेक हानियाँ भी हुई हैं। विज्ञान के विनाशक यंत्रों ने मानव की नींद छीन ली है, उसका सुख-चैन समाप्त कर दिया है। विज्ञान ने मानव को अपने यंत्रों का दास बना दिया है। उसके भाग्य में बेकारी लिख दी है। आज का मानव बाह्य रूप या भौतिक रूप से संपन्न एवं समृद्ध होने पर भी हृदय में अशांति लिए हुए भटक रहा है। उसके पास आध्यात्मिक ज्ञान नहीं रहा। वह आज श्रद्धा व सहानुभूति को भूलकर तर्क-वितर्क की छाया में जी रहा है। वह ईश्वर से विमुख होकर विज्ञान के सहारे जीवित है। अतः आज मानव के जीवन में शांति का अभाव होता जा रहा है।
उपसंहार:
सार रूप में कहा जा सकता है कि विज्ञान अपने-आप में न अच्छा है न बुरा है। वह तो मानव के हाथ में एक शक्ति है। इस शक्ति का प्रयोग हम अच्छे या बुरे रूप में कर सकते हैं। विज्ञान के सदुपयोग से इस संसार को उपवन तथा दुरुपयोग से उजाड़ बनाया जा सकता है।
9. सिनेमा के लाभ तथा हानियाँ
भूमिका:
सिनेमा या चलचित्र आधुनिक युग का एक जन-प्रिय आविष्कार है। ध्वनि और चित्र का अद्भुत संगम है। इसे मनोरंजन का सस्ता एवं शक्तिशाली साधन कहा जा सकता है। यह जन-जन की इच्छा का साकार रूप है। सिनेमा के विकास का हमारे जीवन के राजनीतिक, सामाजिक, आर्थिक, सांस्कृतिक आदि सभी पक्षों पर अत्यधिक प्रभाव पड़ता है।
1. आविष्कार:
अमेरिका के प्रसिद्ध वैज्ञानिक एडीसन ने सर्वप्रथम सिनेमा का आविष्कार किया। अमेरिका के पश्चात् इसका इंग्लैण्ड और फ्रांस में प्रचार हुआ। आज संसार के सभी देशों में इसका चलन है। भारत में पहले-पहल मूक चित्र होते थे। भारत का पहला बोलने वाला चलचित्र ‘आलमआरा’ था। हिंदी में सिनेमा को चलचित्र कहते हैं। सिनेमा विज्ञान की अनुपम देन है। इसके अनेक लाभ हैं।
2. ज्ञान एवं शिक्षा का प्रसार:
सिनेमा से हमारे ज्ञान में वृद्धि होती है। वे स्थान जो हम कभी देखने की कल्पना भी नहीं कर सकते वे सिनेमा के द्वारा बड़ी आसानी से देखे जा सकते हैं। अनेक राजनीतिक एवं ऐतिहासिक भवन, नगर, बाग-बगीचे आदि हमें सिनेमा द्वारा देखने को मिलते हैं। सिनेमा जहाँ मनोरंजन का प्रमुख साधन है वहाँ सिनेमा द्वारा शिक्षा का प्रसार भी हुआ है। आज भूगोल, इतिहास, विज्ञान आदि विषयों को भी सिनेमा द्वारा समझाया व सिखाया जाता है।
3. मनोरंजन का साधन:
सिनेमा मनोरंजन का प्रमुख साधन है। आजकल सिनेमा बड़े-बड़े नगरों तक ही सीमित नहीं रहे अपितु छोटे-छोटे कस्बों में भी अनेक सिनेमाघर बन गए हैं। बड़े-बड़े शहरों में सिनेमा घरों की संख्या अधिक हो गई है। बड़े-से-बड़े व्यक्ति से लेकर छोटे-से-छोटे व्यक्तिं तक मनोरंजन के लिए सिनेमा देखते हैं।
4. समाज सुधार:
सिनेमा जहाँ मनोरंजन का उत्तम साधन है वहाँ समाज सुधार का भी श्रेष्ठ साधन सिद्ध हुआ है। समाज में स्वस्थ सहिष्णुता, नैतिकता एवं चरित्र की पवित्रता की स्थापना किस प्रकार की जाए, इस उद्देश्य से हमारे यहाँ अनेक फिल्मों का निर्माण हो चुका है; जैसे पुकार, भगत सिंह, अछूत कन्या, छत्रपति शिवाजी, जागृति, पैगाम, भाभी, धूल का फूल, सुजाता, तपस्या, स्वामी आदि। ये चलचित्र सामाजिक एवं आर्थिक समस्याओं को लेकर निर्मित हुए हैं।
5. व्यापार में वृद्धि:
सिनेमा व्यापार के प्रचार का भी साधन है। सिनेमा व्यापार की बहुत सारी वस्तुओं के प्रचार का प्रमुख साधन है। दैनिक जीवन में काम आने वाली वस्तुओं आदि का प्रचार चलचित्रों द्वारा ही होता है। सिनेमा अपने-आप में भी बहुत बड़ा व्यापार बन गया है। सिनेमा द्वारा हमें विदेशों के विषय में जानकारी बड़ी आसानी से प्राप्त हो जाती है। सिनेमा द्वारा विदेशियों के रहन-सहन, पहनावा, ज्ञान, शिक्षा, संस्कृति, सभ्यता आदि का पता चल सकता है।
6. हानियाँ:
सिनेमा देखने से जहाँ अनेक लाभ होते हैं वहाँ कुछ हानियाँ भी होती हैं। गंदे व अश्लील चित्र व दृश्य देखने से लोगों के विशेषकर बच्चों और युवकों एवं युवतियों के चरित्र पर बुरा असर पड़ता है। अनेक प्रकार के फैशन के दृश्य देखकर लड़के-लड़कियाँ अपने देश की परंपरा और सभ्यता को भूलकर विदेशी सभ्यता और संस्कृति की ओर उन्मुख हो रहे हैं। सिनेमा के अनेक दृश्यों में बैंक लूटना, स्मगलिंग, शराब पीना आदि दिखाया जाता है जिनका दर्शकों के मन पर बुरा प्रभाव पड़ता है।
उपसंहार:
कहने का अभिप्राय यह है कि सिनेमा से जहाँ अनेक लाभ हैं वहाँ अनेक हानियाँ भी उभरकर सामने आई हैं। सरकार द्वारा यद्यपि सिनेमा सैंसर होकर आते हैं, किंतु फिर भी उनमें गंदे दृश्य, भद्दे संवाद और अश्लील गाने रह जाते हैं। यह सब निर्माता और निर्देशक पर निर्भर करता है।
10. खेलों का जीवन में महत्त्व
नियम से व्यायाम को नित कीजिए।
दीर्घ जीवन का सुधा रस पीजिए ॥
भूमिका:
जीवन में स्वास्थ्य का सबसे अधिक महत्त्व होता है। स्वस्थ मानव ही धरती पर विद्यमान् सुखों को भोग सकता है। विजय उसके चरण चूमती है। स्वस्थ व्यक्ति ही साहस एवं धैर्य से पूर्ण जीवन व्यतीत कर सकता है। यह स्वस्थ जीवन प्राप्त होता है खेलों से। नियम से खेलने वाले व्यक्ति में आत्म-विश्वास और कार्य करने की शक्ति विद्यमान रहती है।
1. खेलों के प्रकार:
हमारे देश में खेलों के अंतर्गत फुटबाल, हॉकी, वालीबाल, क्रिकेट, कबड्डी, कुश्ती, खो-खो, पोलो, तैराकी, घुड़सवारी, लम्बी कूद और हर प्रकार की दौड़ें आती हैं। इनके द्वारा शारीरिक एवं मानसिक शक्ति में वृद्धि के साथ-साथ मनोरंजन भी होता है। वास्तव में, देखा जाए तो खेल-कूद और व्यायाम हमारी शक्ति के स्रोत हैं। जो इन स्रोतों के अनुयायी रहते हैं, वे सदैव शक्तिशाली, चुस्त और आरोग्य रहते हैं। आलस्य उनसे कोसों दूर रहता है। खेलों से शरीर में रक्त की गति तीव्र रहती है, जिससे पाचन-शक्ति ठीक रहती है। संपूर्ण शरीर सुडौल और सुदृढ़ हो जाता है। पुढे शक्तिशाली हो जाते हैं, नेत्रों की ज्योति बढ़ जाती है। मन उल्लास से भरा रहता है।
2. खेलों द्वारा चरित्र निर्माण:
खेलों द्वारा न केवल शारीरिक स्वास्थ्य को लाभ प्राप्त होता है, अपितु मानसिक स्वास्थ्य को भी लाभ होता है। खेलों द्वारा हमारे बौद्धिक विकास में भी सहायता मिलती है। इसके साथ-साथ खेलों द्वारा हमारे चरित्र का निर्माण होता है। इनके द्वारा हमारे मन से कुत्सित विचार या भावनाएँ स्वतः ही दूर हो जाती हैं। खेलों द्वारा आपसी सहयोग की भावना उत्पन्न होती है। खिलाड़ी में आत्म-संयम, आत्म-विश्वास, दृढ़ निश्चय, हानि को सहन करने की क्षमता तथा विजयी होने का निश्चय आदि अनेक गुण उत्पन्न होते हैं जिनसे उसके उत्तम चरित्र का परिचय प्राप्त होता है।
3. राष्ट्र के महान् खिलाड़ी पुरुष:
हमारे देश के महान् पुरुष सदैव अच्छे खिलाड़ी रहे हैं। यहाँ ऐसे वीरों की अनेक गाथाएँ प्रचलित हैं। धनुर्धारी राम, हनुमान, परशुराम, भीम, अर्जुन, अभिमन्यु और श्रीकृष्ण का नाम सुनकर किसकी भुजाएँ न फड़क उठेगी ? उन्होंने व्यायाम, ब्रह्मचर्य और शक्ति-पूजा से ही अचल कीर्ति प्राप्त की है। पृथ्वीराज चौहान के शब्द-भेदी बाण, अकबर बादशाह की घुड़सवारी और स्वामी रामतीर्थ की तैराकी तथा व्यायाम की प्रवृत्ति से कौन परिचित नहीं ? स्वामी दयानंद सरस्वती में शक्ति और स्वास्थ्य का चरमोत्कर्ष हुआ था। राष्ट्रपिता गांधी जी को भ्रमण में तथा जवाहरलाल नेहरू की तैरने में रुचि थी।
4. खेलों से प्राप्त शिक्षाएँ:
खेलों से हमें अनेक शिक्षाएँ प्राप्त होती हैं। खेल संघर्ष द्वारा विजय प्राप्त करने की भावना पैदा करते हैं। खेल हँसते-हँसते अनेक कठिनाइयों का सामना करना सिखाते हैं। खेल के मैदान में खिलाड़ी में अनुशासन में रहने की भावना उत्पन्न होती है। एक-दूसरे को सहयोग देने और भाई-चारे की भावना भी खेलों से मिलती है।
उपसंहार:
संक्षेप में कहा जा सकता है कि खेल-कूद मानव-जीवन का अभिन्न अंग है। जो लोग खेलों के महत्त्व को नहीं समझते, वे जीवन के महान् सुख से वंचित रह जाते हैं। विद्यार्थियों को खेलों में अवश्य भाग लेना चाहिए, क्योंकि खेलों में भाग लेने से उनका शारीरिक और मानसिक विकास होगा तथा चरित्र का निर्माण भी होगा। मनुष्य को दीर्घ आयु के लिए व्यायाम या खेलों का अनुसरण अवश्य करना चाहिए।

11. समय का सदुपयोग
भूमिका:
समय अमूल्य धन है। कुछ लोग चरित्र को सबसे बड़ा धन मानते हैं। महाकवि तुलसीदास ने संतोष को सबसे बड़ा धन माना है। अंग्रेज़ी की एक कहावत में कहा गया है ‘टाईम इज़ मनी’ अर्थात् समय ही धन है। इसी प्रकार यदि पूछा जाए कि सबसे बड़ी हानि क्या है तो उत्तर होगा कि समय व्यर्थ गंवा देना ही सबसे बड़ी हानि है। मानव जीवन का एक-एक क्षण महत्त्वपूर्ण है। बीता समय कभी लौटकर नहीं आता। किसी कवि ने ठीक ही कहा है
“उद्योगी को कहाँ नहीं सु-समय मिल जाता।
समय नष्ट कर नहीं सौख्य कोई भी पाता ॥”
1. समय का सदुपयोग:
धन से हर वस्तु खरीदी जा सकती है, परंतु समय नहीं खरीदा जा सकता। समय का सदुपयोग करने के लिए मनुष्य को चाहिए कि वह अपने दैनिक कार्यों की समुचित रूप-रेखा बना ले तथा उसके अनुसार ही अपने कर्म करे। काम करते समय एक मूल मंत्र हमें सदा याद रखना चाहिए कि आज का काम कल पर नहीं छोड़ना चाहिए।
2. छात्रों द्वारा समय का उचित प्रयोग:
विद्यार्थी जीवन का मानव जीवन में बहुत महत्त्व होता है। विद्यार्थी जीवन में किया गया कार्य जीवन में सदा काम आता है। एक आदर्श छात्र कभी भी अपने समय को व्यर्थ नहीं गंवाता। वह प्रतिदिन अपना काम निरंतर करता रहता है। वह अध्यापकों द्वारा दिए गए गृह कार्य को समय पर कर लेता है।
3. समय का सही उपयोग:
समय का उचित प्रयोग करने के लिए बालक को बाल्यावस्था से ही ऐसी आदतें डालनी चाहिएँ, जिससे वह निश्चित समय के अनुसार प्रत्येक कार्य को करे। उसका दैनिक कार्य अनेक भागों में विभाजित होना चाहिए। निश्चित समय पर स्कूल जाना, पढ़ना, खेलना, सैर करना, भोजन करना, सोना आदि समय के अनुसार होना चाहिए। अतः अच्छे गुण एवं संस्कारों के लिए स्वयं समय का सदुपयोग करना चाहिए, क्योंकि संसार में जितने भी महापुरुष एवं मेधावी हुए हैं, उन्होंने समय का सदुपयोग बुद्धिमत्तापूर्वक किया। नेपोलियन तथा पण्डित नेहरू इसका दृष्टांत हैं।
4. समय को खोना अपूर्णीय हानि:
आदि गुरु शंकराचार्य ने कहा है कि समय को व्यर्थ खोना एक ऐसी हानि होती है जो कभी पूर्ण नहीं होती। बुरे कार्यों में, निद्रा, लड़ाई-झगड़ों में समय की बरबादी करना, मूर्खता की पहचान है। ‘समय काटे नहीं कटता’, ‘कोई काम ही नहीं है करने के लिए’ आदि सोचना मन की मूर्खता ही है। खाली रहना विवेकहीनता दर्शाता है।
5. समय सत्य-पथ-प्रदर्शक:
समय सत्य का मार्ग बताने वाला है। समय की पाबंदी सुशीलता का चिहन है। कल का काम आज निपटाना यशस्वी बनने का साधन है। समय का उचित प्रयोग समय की बचत है, सफलता की कुंजी है। जो लोग समय का सही उपयोग करते हैं वे जीवन में सफल होते हैं। इसीलिए कहा भी गया है-
काल करे सो आज कर, आज करे सो अब।
पल में प्रलय होएगी, बहुरि करेगा कब ॥
उपसंहार:
सार रूप में कहा जा सकता है कि समय अमूल्य धन है। समय से एक क्षण देरी से गाड़ी छूट जाती है। किसान द्वारा बोया गया अन्न समय पर ही उगता है। समय चूक जाने पर वह कितनी मेहनत करे अन्न नहीं उगेगा। अतः हर काम समय पर करना चाहिए।

12. राष्ट्रपिता महात्मा गाँधी
अथवा
मेरा प्रिय नेता
भूमिका:
राष्ट्रपिता महात्मा गांधी जी का स्थान सभी महान् नेताओं में सबसे ऊँचा है। उन्होंने सत्य और अहिंसा के बल पर अंग्रेजों जैसी ताकत को भारत को स्वतंत्र करने के लिए विवश कर दिया था। विश्व के इतिहास में उनका नाम सदा आदर से लिया जाएगा। गांधी जी मानवता के उपासक थे।
1. जन्म:
गांधी जी का जन्म गुजरात के काठियावाड़ के पोरबंदर नामक स्थान पर 2 अक्तूबर, 1869 को हुआ था। यह दिन भारतवर्ष के लिए ही नहीं, अपितु समस्त संसार के लिए शुभ माना जाता है। गांधी जी का पूरा नाम मोहनदास कर्मचंद गांधी था। उनके पिता राजकोट राज्य के दीवान थे। उनकी माता श्रीमती पुतलीबाई धार्मिक विचारों वाली साध्वी नारी थीं।
2. शिक्षा:
गांधी जी की शिक्षा पोरबंदर में आरंभ हुई। वे कक्षा के साधारण विद्यार्थी थे। वे अपने सहपाठियों से बहुत कम बातचीत करते थे। पढ़ाई में वे मध्यम छात्र थे। वे अपने अध्यापकों का आदर करते थे। दसवीं की परीक्षा उन्होंने स्थानीय स्कूल से ही प्राप्त की। तेरह वर्ष की आयु में ही उनका विवाह कस्तूरबा बाई से कर दिया गया।
3. माता को दिए गए तीन वचन:
जब महात्मा गांधी वकालत की शिक्षा ग्रहण करने के लिए इंग्लैंड गए तब वे एक पुत्र के पिता भी बन चुके थे। इंग्लैण्ड जाने से पहले उन्होंने अपनी माता को तीन वचन दिए। उन्होंने माँस न खाने, शराब न पीने और पराई स्त्री को बुरी नजर से न देखने इन तीनों वचनों का पालन भली-भाँति किया। गांधी जी वहाँ से एक अच्छे वकील बनकर लौटे।
4. वकालत की शुरूआत:
गांधी जी ने मुम्बई आकर वकालत का कार्य आरंभ किया। किसी विशेष मुकद्दमे की पैरवी करने के लिए वे दक्षिणी अफ्रीका गए। वहाँ भारतीयों के साथ अंग्रेजों का दुर्व्यवहार देखकर उनमें राष्ट्रीय भाव जागृत हुआ। सन् 1915 में जब वे दक्षिणी अफ्रीका से भारत वापस आए तो उस समय अंग्रेजों का दमन-चक्र जोरों पर था। उस समय भारत में रौलेट एक्ट जैसे काले कानून लागू थे। सन् 1919 की जलियाँवाला बाग की नरसंहारी दुर्घटना ने मानवता को नतमस्तक कर दिया था। उस समय अखिल भारतीय काँग्रेस केवल पढ़े-लिखे मध्यवर्गीय लोगों की एक संस्था थी। देश की बागडोर लोकमान्य बाल गंगाधर तिलक के हाथ में थी। उस समय काँग्रेस की स्थिति इतनी अच्छी न थी। वह दो दलों-नरम दल और गरम दल में विभक्त थी। सन् 1920 में महात्मा गाँधी जी ने असहयोग आन्दोलन का सूत्रपात करके भारतीय राजनीति को एक नया मोड़ दिया।
5. भारत छोड़ो आन्दोलन:
सन् 1942 में महात्मा गांधी जी ने संसार की राजनीतिक परिस्थितियों को देखते हुए ‘भारत छोड़ो आन्दोलन’ का बिगुल बजा दिया। उनका कहना था कि “यह हमारी आखिरी लड़ाई है।” वे अपने साथियों के साथ जेल गए। इस प्रकार उन्होंने 15 अगस्त, 1947 के पावन दिन, भारत को अंग्रेजों से आजादी दिलवाई। इस अवसर पर भारत का बँटवारा हो गया। भारत और पाकिस्तान दो अलग देश बन गए।
6. निधन:
अत्यंत दुःख के साथ कहा जा सकता है कि अहिंसा का पुजारी स्वयं हिंसा का शिकार हो गया। 30 जनवरी, 1948 को नाथूराम गोडसे ने उन्हें गोली मार दी।
निष्कर्ष:
सार रूप में कहा जा सकता है कि अहिंसा के पुजारी महात्मा गांधी जी का नाम इतिहास में सदैव स्वर्ण अक्षरों में लिखा जाएगा। अमन के पुजारियों में उनका नाम सम्मान सहित लिया जाता है। भारत की आने वाली पीढ़ियों को उनके जीवन से शिक्षा लेकर भारत की सेवा करनी चाहिए।

13. यदि मैं प्रधानमंत्री होता ?
भूमिका:
यदि मैं भी कल्पना करने लगूं कि काश! मैं देश का प्रधानमंत्री बन जाऊँ तो इसमें गलत ही क्या है। वास्तविक जीवन में न सही कल्पना में तो मैं प्रधानमंत्री बन ही सकता हूँ। ऐसा कुछ नहीं जो मानव इस संसार में रहता हुआ प्राप्त न कर सके। सिर्फ इन्सान के इरादे पक्के, गहरी लगन और संघर्ष करने की ललक होनी चाहिए। इसीलिए मैं भी सोचता हूँ कि मैं प्रधानमंत्री बन जाऊँ।
1. शांति स्थापना का प्रयास:
यदि मैं प्रधानमंत्री बन जाऊँ तो राज्य के शासकीय कार्यों में जरा भी ढील न देता। मैं सदैव देश के भले की ही बात कहता। मैं व्यापक दृष्टिकोण को अपनाकर भारत में शांति-स्थापना के साथ-साथ विश्व-भर में शांति-स्थापना का प्रयास करता। लालबहादुर शास्त्री की भांति बहादुरी से देश की रक्षा करते हुए शत्रुओं के दांत खट्टे कर देता। श्रीमती इंदिरा गांधी की भांति देश की स्थिति मजबूत करके संपूर्ण विश्व में उसकी प्रतिष्ठा को बढ़ाने का प्रयास करता। अटल बिहारी वाजपेयी की भांति अपनी विदेश नीति से संसार के अन्य देशों व राष्ट्रों से मित्रता बनाने का सफल प्रयास करता।
2. आर्थिक विकास पर बल:
आज देश जिन समस्याओं से घिरा हुआ है, उनके घेरे को तोड़कर देश को समस्याओं से मुक्त राष्ट्र का रूप प्रदान करने की कोशिश करता। यदि मैं प्रधानमंत्री बन जाऊँ तो सबसे पहले देश को आर्थिक दृष्टि से मजबूत करूँगा ताकि हमें आर्थिक सहायता के लिए दूसरे देश के सामने हाथ न फैलाने पड़ें। मैं देश की गरीबी को दूर करने का पूरा प्रयास करता।
3. प्राकृतिक संपदा की रक्षा:
प्रधानमंत्री का पद संभालने पर मैं देश की प्राकृतिक संपदा की सुरक्षा की ओर ध्यान दूंगा, क्योंकि किसी भी राष्ट्र के विकास का मुख्य आधार वहाँ की प्राकृतिक संपदा होती है। मैं नदियों के जल का सही प्रयोग करके जहाँ एक ओर विद्युत् उत्पन्न करने की योजनाएँ लागू करूँगा वहाँ नदियों के पानी से अपने देश की धरती को सींचूँगा जिससे उस पर अधिक फसलें उगाई जा सकें और पीने का पानी उपलब्ध हो सके।
4. सीमा सुरक्षा पर बल:
प्रधानमंत्री होने के नाते देश की सीमाओं की सुरक्षा को मजबूत करना मैं अपना कर्त्तव्य समझता हूँ। मैं अपने देश की सेना को मजबूत बनाने के लिए आधुनिकतम हथियार उपलब्ध करवाता। सेना के जवानों का वेतन बढ़ा देता। उनके परिवारजनों के कल्याण की योजनाएँ लागू करता। देश के लिए शहीद होने वाले वीर सैनिकों को राष्ट्रीय सम्मान दिलवाता। अपने देश के सवा सौ करोड़ लोगों के ढाई सौ करोड़ हाथों की सहायता से पाकिस्तान को मुँह तोड़ जवाब देता।
5. भ्रष्टाचार उन्मूलन पर बल:
देश की अन्य बड़ी समस्या जो देश को भीतर-ही-भीतर खाए जा रही है, वह है भ्रष्टाचार। भ्रष्टाचार ने जहाँ देश को कमजोर किया है वहीं हमारे देश की शाखा को विश्व की मार्किट में भी धक्का लगा है। मैं भ्रष्टाचार की समस्या को जड़ से उखाड़ फेंकने का प्रयास करता। भ्रष्टाचारी लोगों से निपटने के लिए कड़ी कानून व्यवस्था की जाती। कानून को तोड़ने वाले लोगों से सख्ती से निपटा जाता, तभी देश से भ्रष्टाचार का बोलबाला समाप्त किया जा सकता।
6. जनसंख्या पर नियंत्रण:
भारतवर्ष में विभिन्न समस्याओं को जन्म देने वाली प्रमुख समस्या है-जनसंख्या की वृद्धि । जनसंख्या की वृद्धि के कारण रोटी, कपड़ा, मकान, बेरोज़गारी, भुखमरी आदि अनेक समस्याएँ उत्पन्न हो गईं हैं। आर्थिक योजनाओं के सफल न होने का कारण भी यह जनसंख्या वृद्धि है। यदि मैं प्रधानमंत्री बन जाऊँ तो सबसे पहले जनसंख्या वृद्धि को रोकने के लिए परिवार कल्याण योजनाओं को सख्ती से लागू करूँगा।
निष्कर्ष:
अंत में मैं कहना चाहूँगा कि ये सब कार्य मैं देशवासियों की सहायता द्वारा या उनके सहयोग से करता, अकेला प्रधानमंत्री कुछ नहीं कर सकता। यदि मैं प्रधानमंत्री होता तो मैं देश की जनता के सहयोग से भारत को उसके विश्व शिरोमणि के पद पर आसीन करवा देता।
14. विद्यार्थी और अनुशासन
भूमिका:
किसी भी भवन की मज़बूती उसकी नींव पर निर्भर करती है। यदि नींव मज़बूत है तो उस पर बना भवन भी सुदृढ़ होगा और लंबे काल तक टिका रहेगा। इसी प्रकार से राष्ट्र की सुदृढ़ता उसके युवकों पर निर्भर करती है। यदि हमारे देश के युवक अनुशासित एवं सुशिक्षित होंगे तो देश का भविष्य सुरक्षित होगा, विद्यार्थी अनुशासित नहीं होंगे तो कभी भी हमारे देश पर विपत्ति के बादल मंडरा सकते हैं।
अनुशासन का अर्थ:
अनुशासन’ शब्द का अर्थ है-शासन के पीछे-पीछे चलना। शासन का अर्थ है-सुव्यवस्था अर्थात् समाज को सुचारु रूप से चलाने के नियमों का पालन करना। जीवन के प्रत्येक क्षेत्र में अनुशासन अनिवार्य है। परिवार हो या समाज, सर्वत्र अनुशासन का पालन करने से मानव जीवन समृद्ध होता है। अनुशासन के अनेक लाभ हैं। इसका पालन करने से आलस्य और कायरता दूर भागते हैं। मनुष्य अपने कर्तव्य का सही पालन करता है। व्यक्ति में सच्चाई और ईमानदारी जैसे सद्गुण विकसित होते
विद्यार्थी और अनुशासन:
विद्यार्थी जीवन में अनुशासन नितांत आवश्यक है। विद्यार्थी एक नन्ही कोंपल के समान होता है। उसे जो भी रूप दिया जाए, वह उसे ग्रहण करता है। उसका मन शीघ्र प्रभावित होता है। अतः बाल्यावस्था से ही विद्यार्थी को अनुशासन की शिक्षा दी जानी चाहिए। हम इस बात का ध्यान रखें कि बालक-बालिकाएँ अपना-अपना काम नियमित रूप से करें। इस दिशा में माता-पिता का दायित्व और भी अधिक महत्त्वपूर्ण है। बच्चे की शिक्षा का प्रथम विद्यालय उसका घर ही है। यदि माता-पिता स्वयं अनुशासित हैं तो बालक भी अनुशासन की भावना ग्रहण करेगा। .
प्राचीनकाल में विद्यार्थी जीवन:
प्राचीन भारत में विद्यार्थी गुरुकुलों में शिक्षा ग्रहण करने जाते थे। 25 वर्ष तक विद्यार्थी ब्रह्मचर्य का पालन करते थे और यह काल शिक्षा ग्रहण करने में व्यतीत करते थे। उन दिनों गुरुकुलों का वातावरण बहुत ही पावन और अनुशासित होता था। प्रत्येक विद्यार्थी अपने गुरुजनों का सम्मान करता था। शिक्षा के साथ-साथ उसे गुरुकुल के सारे काम भी करने पड़ते थे। छोटे-बड़े या अमीर-गरीब सभी एक ही गुरु के चरणों में विद्या ग्रहण करते थे। श्रीकृष्ण और सुदामा ने इकट्ठे संदीपन ऋषि के आश्रम में अनुशासनबद्ध होकर शिक्षा ग्रहण की।।
वर्तमान स्थिति:
आज हमारे देश के विद्यार्थियों में अनुशासन का अभाव है। वे न तो माता-पिता का कहना मानते हैं और न ही गुरुजनों का। प्रतिदिन स्कूलों और कॉलेजों में हड़तालें होती रहती हैं। इस प्रकार के समाचार पढ़ने को मिलते रहते हैं कि विद्यार्थियों ने बस जला डाली या पथराव किया। पुलिस और विद्यार्थियों की मुठभेड़ तो होती ही रहती है। राजनीतिक दल भी समय-समय पर अपने स्वार्थों को पूरा करने के लिए विद्यार्थियों को भड़काते रहते हैं। विद्यार्थियों की अनुशासनहीनता के अनेक दुष्परिणाम हमारे सामने आ रहे हैं। ये विद्यार्थी परीक्षाओं में नकल करते हैं, शिक्षकों को धमकाते हैं और विश्वविद्यालयों के वातावरण को दूषित करते रहते हैं। अनेक विद्यार्थी हिंसात्मक कार्रवाई में भी भाग लेने लगे हैं। इससे राज्य की सुख-शांति में बाधा उत्पन्न होती है। निश्चय ही, आज विद्यार्थियों में अनुशासन की कमी आ चुकी है।
अनुशासनहीनता के कारण:
आज की शिक्षण संस्थाओं का प्रबंध भी विद्यार्थियों को अनुशासनहीन बनाता है। वे विद्यालय के अधिकारियों और शिक्षकों की आज्ञा का उल्लंघन करते हैं। ऐसा भी देखने में आया है कि स्कूलों में छात्र-छात्राओं की भीड़ लगी रहती है। भवन छोटे होते हैं और शिक्षकों की संख्या कम। कॉलेजों में तो एक-एक कक्षा में सौ-सौ विद्यार्थी होते हैं। ऐसी अवस्था में क्या तो शिक्षक पढ़ाएगा और क्या विद्यार्थी पढ़ेंगे। कॉलेजों में छात्रों के दैनिक कार्यों की ओर कोई ध्यान नहीं दिया जाता। रचनात्मक कार्यों के अभाव में छात्र का ध्यान व्यर्थ की बातों की ओर जाता है। यदि प्रतिदिन विद्यार्थी के अध्ययन-अध्यापन की ओर ध्यान दिया जाए तो निश्चित रूप से वह अपना काम अनुशासनपूर्वक करेगा।
नैतिक शिक्षा की आवश्यकता:
छात्रों में अनुशासन की भावना स्थापित करने के लिए यह ज़रूरी है कि वर्तमान शिक्षा प्रणाली में नैतिक और चारित्रिक शिक्षा के लिए कुछ स्थान हो। इससे विद्यार्थी को अपने अधिकारों के साथ-साथ कर्तव्यों का भी ज्ञान होगा। दूसरी बात यह है कि विद्यार्थियों के शारीरिक विकास की ओर उचित ध्यान दिया जाना चाहिए। इसके लिए शिक्षण के साथ-साथ खेल-कूद को भी अनिवार्य घोषित किया जाना चाहिए। इसी प्रकार से एन०सी०सी० और एन०एस०एस० जैसे रचनात्मक कार्यों पर अधिक बल दिया जाना चाहिए।
उपसंहार:
वर्तमान शिक्षण-पद्धति में परिवर्तन करके महापुरुषों की जीवनियों से भी छात्र-छात्राओं को अवगत कराया जाना चाहिए। यथासंभव व्यावसायिक शिक्षा की ओर अधिक ध्यान दिया जाना चाहिए ताकि स्कूल से निकलते ही विद्यार्थी अपने व्यवसाय का शीघ्र चयन करें। प्रतिमाह मासिक परीक्षा अवश्य होनी चाहिए। इस कार्यक्रम को सुचारु ढंग से चलाने के लिए त्रैमासिक और अर्द्धवार्षिक परीक्षाएँ भी आयोजित की जाएँ। सबसे बढ़कर विभिन्न राजनीतिक दलों को शैक्षणिक संस्थाओं में किसी भी प्रकार का हस्तक्षेप नहीं करना चाहिए। सरकार भी यह बात पूरी तरह से जान ले कि छात्रों में अनुशासन स्थापित किए बिना देश सुदृढ़ नहीं हो सकता।
15. प्रदूषण की समस्या
भूमिका:
वर्तमान युग में विश्व के सामने अनेक समस्याएँ चुनौती बनी हुई हैं। प्रदूषण की समस्या उनमें से एक प्रमुख समस्या है। प्रदूषण के कारण आज मानव का जीवन खतरे में पड़ गया है। यह समस्या विज्ञान की देन है। यह महा उद्योगों की समृद्धि का बोनस है। यह मानव को मौत के मुँह में धकेलने की अनाचारी चेष्टा है। यह बीमारियों को निमंत्रण है। यह प्राणिमात्र के अमंगल की अप्रत्यक्ष कामना है।
1. प्रदूषण का अर्थ:
प्रदूषण का अर्थ है-पर्यावरण में असंतुलन आना। हवा, जल, मिट्टी, पौधे, वृक्ष और पशु मिलकर पर्यावरण की रचना करते हैं और पारस्परिक संतुलन रखने के लिए दूसरे को प्रभावित करते हैं। यह प्रतिक्रिया विज्ञान संबंधी संतुलन कहलाती है। कभी-कभी कुछ कारणवश परिवर्तन आ जाता है। यदि इस परिवर्तन की प्रक्रिया का प्रकृति के साथ सामंजस्य न किया जाए तो इससे ऐसी स्थिति पैदा हो जाती है कि मानव-जीवन खतरे में पड़ जाता है। यह असंतुलन ही प्रदूषण का जन्मदाता है।
2. प्रदूषण का मानव:
जीवन पर प्रभाव पर्यावरण-प्रदूषण का मानव-जीवन पर अत्यंत विपरीत प्रभाव पड़ रहा है। वायुमण्डल में कार्बन-डाइ-ऑक्साइड की मात्रा में 16 प्रतिशत की वृद्धि हो गई है। यदि इसकी वृद्धि इसी प्रकार होती रही तो नगरों में रहने वाले लोग श्वास-रोग और नेत्र रोग के शिकार हो जाएँगे। कारखानों में जलाए जाने वाले ईंधन से जो धुआँ और गैस निकलती है उनसे खाँसी और फेफड़ों संबंधी रोग हो जाते हैं। इतना ही नहीं, दूषित पर्यावरण से कैंसर जैसे रोग भी फैलते हैं।
3. जल-प्रदूषण:
वायु-प्रदूषण के अतिरिक्त जल-प्रदूषण भी मानव-जीवन के लिए हानिकारक है। घरेलू गंदा पानी, नालियों में प्रवाहित मल तथा कारखानों से निकलने वाले व्यर्थ पदार्थ नदियों और समुद्रों में प्रवाहित कर दिए जाते हैं। इससे पानी जहरीला बन जाता है। पानी में ऑक्सीजन की मात्रा कम हो जाती है जिससे जल-प्राणी के जीवन को खतरा बढ़ जाता है।
4. रासायनिक पदार्थों द्वारा प्रदूषण:
रासायनिक पदार्थों के अनियमित प्रयोग द्वारा भी पर्यावरण प्रदूषित होता है। प्रायः किसान लोग पैदावार की बढ़ोत्तरी के लिए रसायनों और कीटनाशक दवाइयों का प्रयोग करते हैं, जिनका स्वास्थ्य पर हानिकारक प्रभाव पड़ता है।
5. ध्वनि-प्रदूषण:
वायु एवं जल-प्रदूषण की भाँति ध्वनि-प्रदूषण भी मानव-जीवन के लिए हानिकारक है। वर्तमान यांत्रिक युग में ध्वनि-प्रदूषण भी एक समस्या बनी हुई है। तीव्र ध्वनि का प्रभाव हमारी श्वसन-प्रक्रिया पर पड़ता है। इससे पाचन-क्रिया भी प्रभावित होती है। इसका सीधा प्रभाव अनुकम्पी तंत्रिका तंत्र से नियन्त्रित होने वाले अवयवों पर पड़ता है, जिससे उनमें तनाव बढ़ जाता है और नेत्र-ज्योति मंद पड़ जाती है।
6. प्रदूषण की रोकथाम के उपाय:
वन प्रदूषण की रोकथाम में महत्त्वपूर्ण कार्य करते हैं। वे वायुमंडल में गैसों के अनुपात को समान रखते हैं। बाढ़, भू-स्खलन, भू-क्षरण, रेगिस्तानों के विस्तार, जल-स्रोतों के सूखने तथा वायु-प्रदूषण के रूप में होने वाली तबाही से भी जन-जन की रक्षा करते हैं। इसलिए उनकी रक्षा करना मानव का परम धर्म है। सत्ता का कानून, अनुसंधान संस्थाओं के अनुसंधान, औद्योगिक संस्थाओं के अथक प्रयास और इस समस्या के प्रति जन-जन की जागरूकता ही प्रदूषण की समस्या को दूर कर सकती है। ये समन्वित प्रयास ही इस अदृश्य शत्रु का संहार कर सकेंगे।
निष्कर्ष:
सार रूप में कहा जा सकता है कि शुद्ध जल, शुद्ध वायु, स्वच्छ भोजन तथा शांत वातावरण मानव-जीवन की सुरक्षा के लिए आवश्यक तत्त्व हैं। प्रदूषण एक गम्भीर समस्या है। हमें इसका मुकाबला हिम्मत से करना होगा। इस समस्या को समाप्त करके ही हम प्राणिमात्र के दीर्घ जीवन की कामना कर सकते हैं।

16. बढ़ती हुई महँगाई
भूमिका:
आज संसार के सामने अनेक समस्याएँ हैं। महँगाई उनमें से एक प्रमुख समस्या है। महँगाई के कारण आज समाज के निम्न वर्ग तथा मध्य वर्ग की दशा अत्यंत शोचनीय होती जा रही है। हर वस्तु की कीमत आकाश को छू रही है। सरकार महँगाई को रोकने के लिए अनेक घोषणाएँ कर चुकी है। किंतु वह इसे रोकने में पूर्णतः असफल रही है। महँगाई का बुरा प्रभाव बच्चे से लेकर बूढ़े व्यक्ति तक पर पड़ रहा है। महँगाई के अनेक कारण हैं।
1. जनसंख्या में वृद्धि:
महँगाई का प्रमुख कारण है-जनसंख्या में निरंतर वृद्धि । जनसंख्या में वृद्धि के कारण लोगों के खाने-पीने की वस्तुएँ कम पड़ जाती हैं, जबकि खरीदने वाले अधिक होते हैं। इसलिए उनकी कीमतों में वृद्धि आती है। यही दशा आवास और अन्य वस्तुओं की भी है।
2. उत्पादन में कमी:
भारत एक कृषि-प्रधान देश है। यहाँ की कृषि वर्षा पर निर्भर करती है। यदि समय पर वर्षा नहीं होती तो सूखा पड़ जाने के कारण कृषि के उत्पादन में कमी आ जाती है, जिससे कारखानों में कच्चा माल नहीं पहुंच पाता। इस प्रकार कारखानों में भी उत्पादन कम हो जाएगा। फलस्वरूप वस्तुओं की कीमतें बढ़ने लगेंगी।
3. भ्रष्ट वितरण प्रणाली:
देश में अच्छी वितरण-प्रणाली न होने के कारण भी महँगाई बढ़ती है। कुछ लोग आवश्यकता से अधिक सामान खरीद लेते हैं, जबकि दूसरों के पास अपनी आवश्यकताओं को पूरा करने के लिए भी वस्तुएँ नहीं होतीं। इस प्रकार दोषपूर्ण वितरण प्रणाली के कारण भी महँगाई में वृद्धि हुई है। यदि वस्तुओं की खरीद और वितरण विभाग के कर्मचारी ईमानदारी से काम करें तो कुछ सीमा तक इस समस्या को कम किया जा सकता है।
4. जमाखोरी:
उपज जब मंडियों में आती है, तब अमीर व्यापारी अत्यधिक मात्रा में अनाज एवं अन्य वस्तुएँ खरीदकर अपने गोदाम भर लेता है और इस प्रकार बाजार में वस्तुओं की कमी हो जाती है। व्यापारी अपने गोदामों की वस्तुएँ तभी निकालता है जब उसे कई गुना अधिक कीमत प्राप्त होती है।
5. युद्ध के कारण:
युद्धों के कारण भी महँगाई बढ़ती है। उदाहरणार्थ, भारत को निरंतर कई युद्धों का सामना करना पड़ा इसलिए यहाँ महँगाई भी निरंतर बढ़ी है। विशेषकर बांग्लादेश को आजाद करवाने के संघर्ष का देश को भारी मूल्य चुकाना पड़ा। आज भी पाकिस्तानी उग्रवादियों की घुसपैठ के कारण हमारी सरकार पर आर्थिक बोझ निरंतर बढ़ता जा रहा है।
6. महँगाई के दुष्परिणाम:
कहा जा चुका है कि महँगाई का प्रभाव समाज के प्रत्येक वर्ग पर पड़ता है। लोगों को जीवन-निर्वाह करना कठिन हो जाता है। लोगों का जीवन-स्तर गिर जाता है। बच्चे अच्छी शिक्षा प्राप्त नहीं कर सकते। देश में विकास की योजनाओं में बाधा पड़ती है। आर्थिक विकास की गति कम होने के कारण समाज में जमाखोरी और भ्रष्टाचार का बोलबाला हो जाता है।
उपसंहार:
महँगाई की समस्या संपूर्ण विश्व के लिए एक गम्भीर समस्या है। इससे समाज का हर वर्ग प्रभावित होता है। हम महँगाई पर काबू पाकर ही अपना और आने वाली पीढ़ियों का भविष्य सुरक्षित कर सकते हैं। सरकार को चाहिए कि वह भ्रष्टाचार और जमाखोरी के विरुद्ध कड़े कदम उठाए और ऐसे कार्यों में लगे लोगों को कड़ी सजा दे।
17. जनसंख्या वृद्धि-एक समस्या
भूमिका:
बढ़ती हुई जनसंख्या केवल भारत की ही नहीं, अपितु संसार की समस्या बन चुकी है। भारत जैसे विकासशील देश के लिए यह एक बहुत बड़ी समस्या है। देश की समृद्धि के लिए सरकार द्वारा किए गए विकास के कार्यों में बाधा का प्रमुख कारण बढ़ती हुई जनसंख्या ही है। इसलिए भारत सरकार ने इसकी रोकथाम के लिए ‘परिवार नियोजन’ की योजना बनाई है, जिसमें सरकार को काफी हद तक सफलता भी मिली है।
1. प्राचीन परिस्थिति:
वेदों में दस पुत्रों की कामना की गई है। सावित्री ने यमराज से अपने लिए सौ भाइयों तथा सौ पुत्रों का वर माँगा था। कौरव सौ भाई थे। यह उस समय का वर्णन है जब जनसंख्या इतनी कम थी कि समाज की सुरक्षा और समृद्धि के लिए मानव-शक्ति की बहुत जरूरत थी।
2. वर्तमान स्थिति:
आज की स्थिति इसके विपरीत हो गई है। सन् 1981 की जनगणना के अनुसार भारत की जनसंख्या 78.5 करोड़ थी, किंतु आज यह निरंतर बढ़ती जा रही है। आजादी मिलने के पश्चात् हर मास भारत में दस लाख की आबादी बढ़ती रही है। वर्तमान में भारत की जनसंख्या सवा सौ करोड़ से भी अधिक हो चुकी है। इस स्थिति में भोजन, कपड़ा और आवास का तो अभाव हो ही गया है, इसके अतिरिक्त और भी अनेक समस्याएँ उत्पन्न हो गई हैं।
3. जनसंख्या वृद्धि के प्रभाव:
वस्तुतः देश की जनसंख्या ही उसकी शक्ति का आधार होती है। परंतु जब यह अनियंत्रित गति से बढ़ेगी तो निश्चित ही यह देश के लिए बोझ सिद्ध होगी। निश्चित सीमा से अधिक आबादी किसी देश के लिए गौरव की बात कदापि नहीं कही जा सकती। ऐसी दशा में तो जनसंख्या एक अभिशाप ही कही जाएगी। भारत इस समय की आबादी की दृष्टि से दुनिया का दूसरा बड़ा देश है।
4. जनसंख्या वृद्धि के कारण:
हमारे देश में अनेक कारणों से जनसंख्या में वृद्धि हो रही है। शिक्षा के अभाव में भी संतान उत्पत्ति में वृद्धि होती है। अनपढ़ लोग बच्चों को भगवान की देन मानते हैं। इसके अतिरिक्त कुछ लोग पुत्र प्राप्त करने के लालच में संतान उत्पन्न करते रहते हैं। मजदूर वर्ग की धारणा है कि जितने अधिक हाथ होंगे उतनी मजदूरी अधिक मिलेगी।
5. जनसंख्या वृद्धि कम करने के उपाय:
भारत सरकार ने जनसंख्या की बढ़ोतरी को कम करने के लिए कई कदम उठाए हैं। सरकार ने विवाह की आयु निश्चित कर दी है। कम उम्र में विवाह करना गैर-कानूनी समझा जाता है। सरकार ने जनसंख्या की वृद्धि को कम करने के लिए परिवार कल्याण पर भी बल दिया है। इसके अतिरिक्त सरकार अनेक प्रकार से जनता में जागृति उत्पन्न करने का भी प्रयत्न कर रही है।
6. परिवार कल्याण का महत्त्व:
परिवार कल्याण से मनुष्य को अनेक लाभ होंगे। ‘छोटा परिवार’ निःसन्देह ‘सुखी परिवार’ होता है। परिवार में अधिक संतान होने से बच्चों के पालन-पोषण व शिक्षा-दीक्षा का उचित प्रबंध नहीं हो पाता। बच्चे कुपोषण का शिकार होते हैं, जिससे उन्हें अनेक प्रकार के रोग आ घेरते हैं। अधिक संतान होने से माँ का स्वास्थ्य खराब हो जाता है।
उपसंहार:
सार रूप में कहा जा सकता है कि जनसंख्या की अनियन्त्रित वृद्धि से देश के विकास में बाधा पड़ेगी और अनेक समस्याएँ उत्पन्न होंगी। इसके समाधान का प्रमुख साधन परिवार कल्याण ही है। हमें जनता में परिवार कल्याण के प्रति जागृति लानी होगी। हमें केवल सरकार के भरोसे नहीं रहना होगा। इस कार्य में हर नागरिक का सहयोग प्राप्त करना होगा। तभी हम इस गम्भीर समस्या का हल ढूँढ सकेंगे।
18. गणतंत्र दिवस
अथवा
हमारा राष्ट्रीय पर्व
प्रजातंत्र के दीप जले हैं, आज नई तरुणाई ले।
भारतमाता की जय बोले, देश नई अंगड़ाई ले ॥
भूमिका:
किसी भी देश के जीवन में राष्ट्रीय त्योहारों का बहुत महत्त्व होता है। हमारे राष्ट्रीय त्योहारों में ‘स्वतंत्रता-दिवस’ और ‘गणतंत्र-दिवस’ प्रमुख हैं। 15 अगस्त हमारा स्वतंत्रता दिवस होता है, क्योंकि 15 अगस्त, 1947 को हमारे देश को कई सदियों के बाद स्वतंत्रता मिली थी। 26 जनवरी को हमारे ‘गणतंत्र-दिवस’ का राष्ट्रीय पर्व होता है। इस शुभ (26 जनवरी, 1950) दिन को हमारा देश पूर्ण गणतंत्र राष्ट्र घोषित हुआ था।
1. गणतंत्र दिवस का पूर्व इतिहास:
गणतंत्र दिवस के पीछे एक इतिहास है। 31 दिसम्बर, 1929 को रावी नदी के किनारे लाहौर में काँग्रेस का अधिवेशन हुआ था जिसकी अध्यक्षता पण्डित जवाहरलाल नेहरू ने की थी। उन्होंने प्रस्ताव रखा कि “काँग्रेस का ध्येय पूर्ण स्वराज्य की प्राप्ति है।” 26 जनवरी को तिरंगे की शपथ लेकर लाखों लोगों ने प्रतिज्ञा की कि हमारा लक्ष्य पूर्ण स्वराज्य प्राप्त करना है और इसकी प्राप्ति के लिए हम अपना बलिदान भी दे देंगे।
2. संविधान लागू करना:
26 जनवरी, 1950 को भारतीय संविधान लागू किया गया। इसी दिन भारत को गणतंत्र राज्य की मान्यता मिली। डॉ० राजेन्द्र प्रसाद को देश का प्रथम राष्ट्रपति बनाया गया।
3. गणतंत्र दिवस मनाए जाने की विधि:
भारतीय इतिहास में गणतंत्र-दिवस का बहुत महत्त्व है। इस शुभ दिन को देश-भर में बड़े उत्साह और उमंग से मनाया जाता है। इस दिन लोगों में उत्साह और प्रेरणा जागृत करने के लिए विभिन्न राज्यों में सरकार की ओर से आनंदवर्द्धक कार्यक्रम रखे जाते हैं। प्रातः राष्ट्रीय ध्वज फहराने के साथ-साथ सैनिक परेड, युद्ध-सामग्री का प्रदर्शन, स्कूल तथा कॉलेज के छात्र-छात्राओं के कार्यक्रम, विभिन्न प्रान्तों के पहनावे आदि का रूप प्रदर्शित किया जाता है। संपूर्ण देश एक अतीव उत्साह-उमंग में डूब जाता है।
4. राष्ट्रीय पर्व:
गणतंत्र-दिवस हमारा राष्ट्रीय पर्व है। वैसे तो प्रत्येक नगर, गाँव इस पर्व को बहुत उत्साहपूर्वक मनाता है, लेकिन देश की राजधानी दिल्ली का आलम कुछ और ही होता है। दिल्ली में राष्ट्रपति की राजकीय सवारी निकलती है। विजय चौक पर राष्ट्रपति जल, थल एवं वायु सेना की सलामी लेते हैं। वायुयान आकाश में तथा तोपें धरती पर भारत के राष्ट्रपति का अभिवादन करती हैं। तीनों सेनाओं की टुकड़ियाँ मार्च करती हुईं लालकिले तक पहुँचती हैं। विभिन्न प्रकार की झाँकियाँ 26 जनवरी की शोभा यात्रा में चार चाँद लगा देती हैं। अपने-अपने प्रान्तों की वेश-भूषा से लोक-नर्तक नृत्य-प्रदर्शन से अपनी प्रान्तीय संस्कृति का परिचय देते हैं।
उपसंहार:
सार रूप में कहा जा सकता है कि 26 जनवरी का राष्ट्रीय उत्सव भारतीय नागरिकों के मन में एक अनोखा उत्साह और आत्मचिन्तन की भावना भरने वाला उत्सव है। इस पर्व में हर भारतीय नागरिक को बढ़-चढ़कर भाग लेना चाहिए। इस दिन भारतवासियों को यह विचार करना चाहिए कि हमने क्या खोया और क्या पाया ? हमें अपने द्वारा निश्चित की गई योजनाओं में कहाँ तक सफलता मिली है। जो लक्ष्य हमने निर्धारित किए थे, उन्हें प्राप्त करने में हम कहाँ तक सफल हुए हैं। इस प्रकार हमें इस शुभ अवसर पर आत्म-संकल्प करते हुए आगे बढ़ने का निश्चय दोहराना चाहिए।
19. ऋतुराज बसंत
देखा मधुर बसंत सुहाई, पवन चले सुखदाई।
डाली-डाली डोल रही है, कली-कली विकसाई।
चहक उठे पंछी पेड़ों पर, अरुण धूप है छाई।
अहा शीत है भागा घर को, मीठी मधु ऋतु आई।
भूमिका:
भारतवर्ष ऋतुओं का देश है। संसार के सभी देशों से भारत में अधिक ऋतुएँ आती हैं। संसार में ऐसा कोई भी देश नहीं जहाँ वर्ष में छह ऋतुएँ आती हों, ये ऋतुएँ अपने-अपने समय पर आकर अपना रंग दिखा जाती हैं। हर ऋतु अपने समय पर आकर यहाँ की जनता के मन में नए सिरे से उत्साह और काम करने की प्रेरणा भर जाती है। यहाँ के लोग भी ऋतुओं के अनुसार अपने कार्यक्रम निश्चित करते हैं। बसंत ऋतु सभी ऋतुओं में श्रेष्ठ एवं प्रिय ऋतु मानी जाती है। इसके आगमन पर चारों ओर प्रसन्नता छा जाती है। इसलिए इसे ऋतुराज बसंत कहते हैं।
1. बसंत का आगमन:
बसंत ऋतु के आगमन पर चारों ओर खुशियों की लहर छा जाती है। इस समय सर्दी का अंत और गर्मी का आरंभ हो रहा होता है। इस मौसम में सर्दी से कोई ठिठुरता नहीं और गर्मी किसी का बदन नहीं जलाती। हर एक व्यक्ति बाहर घूमने-फिरने की इच्छुक होता है। यही इस ऋतु की विशेषता है।
2. प्राकृतिक सौंदर्य:
सभी जीव-जंतुओं, पेड़-पौधों आदि में नवजीवन का संचार हो जाता है। वृक्ष नए-नए पत्तों से लद जाते हैं। फूलों का सौंदर्य तथा हरियाली की छटा मन को मुग्ध कर देती है। आम के वृक्षों पर बौर आ जाता है तथा कोयल भी मधुर स्वर में कुहू कुहू करती है। इस सुगन्धित वातावरण में सैर करने से बीमारियाँ भी कोसों दूर भाग जाती हैं। ठण्डी-ठण्डी बसंती पवन मनुष्य की आयु और बल में वृद्धि कर देती है।
3. बसंत ऋतु का आनंद:
बसंत ऋतु में अपूर्व चैतन्य होता है। प्रकृति की अनोखी छटा प्रायः मानव के मन पर बड़ा प्रभाव डालती है। सरसों अपना पीला दुपट्टा ओढ़े प्रकृति-प्रेमियों का स्वागत करती है। पक्षी मधुर कलरव के संगीत की साधना करते प्रतीत होते हैं। मनुष्य ही नहीं, पशु-पक्षी भी इस ऋतु में हर्षोल्लास में उछलते-कूदते और नाचते हैं। इसीलिए कहते हैं कि बसंत ऋतु सब ऋतुओं का राजा है।
4. उत्सव:
बसंत ऋतु के आते ही विभिन्न उत्सव मनाने का सिलसिला आरंभ हो जाता है। बसंत ऋतु में होली का त्योहार प्रमुख रूप से मनाया जाता है। इस अवसर पर लोग नाच-गाकर अपने मन की प्रसन्नता प्रकट करते हैं। इसी ऋतु में बसंत पंचमी का उत्सव भी बड़े चाव से मनाया जाता है। बसंत ऋतु में कवियों की भावना-शक्ति भी उत्तेजक हो उठती है। बंगाल में सरस्वती का पूजन किया जाता है। उत्तरी भारत में नवरात्रों का उत्सव भी इसी समय मनाया जाता है। इस अवसर पर लोग शक्ति की देवी दुर्गा की पूजा करते हैं।
उपसंहार:
निष्कर्ष रूप में कहा जा सकता है कि बसंत ऋतु प्रसन्नता और खुशियों की ऋतु है। मानव-जीवन पर इस ऋतु का विभिन्न प्रकार से प्रभाव पड़ता है। इस ऋतु में प्रातःकाल में भ्रमण करने से मनुष्यों का शारीरिक एवं मानसिक विकास होता है। व्यायाम के लिए भी यह ऋतु अत्यंत उपयोगी है। इस ऋतु में सबसे अधिक त्योहार मनाए जाते हैं जिनसे हमें अनेक शिक्षाएँ एवं प्रेरणाएँ मिलती हैं। अतः इसे ऋतुओं का राजा कहना अतिशयोक्ति नहीं होगी।
20. नर हो न निराश करो मन को
भूमिका:
‘नर’ शब्द का सामान्य अर्थ पुरुष होता है। वस्तुतः नर और पुरुष एक शब्द के व्यंजन हैं। पुरुष का अर्थ भी नर है और नर का अर्थ पुरुष, किंतु ‘नर’ शब्द पर यदि गंभीरता या सूक्ष्मता से सोचा जाए तो स्पष्ट हो जाता है कि ‘नर’ शब्द के लाक्षणिक या व्यंजक प्रयोग से पुरुष की विशिष्टता को व्यक्त किया जाता है। पुरुष के पुरुषत्व तथा श्रेष्ठ वीरत्व. के भाव को अभिव्यक्त करने के लिए ‘नर’ शब्द का प्रयोग किया जाता है। इन अभिव्यंजित तथ्यों के प्रकाश में जब हम इस शीर्षक पंक्ति, ‘नर हो, न निराश करो मन को’ के अर्थ और भाव-विस्तार का विवेचन करते हैं तो मानव-जीवन के अनेक पक्ष स्वतः उजागर होने लगते हैं। मनुष्य के पौरुषत्व और पुरुषार्थ की महिमा जगमगा उठती है।
1. छोटी-सी असफलता से निराश न होना:
इस पंक्ति को पढ़ने से ऐसा प्रतीत होता है जैसे कोई हमें चुनौती भरे शब्दों में या ललकार भरी ध्वनि में कह रहा हो-वाह ! कैसे पुरुष हो तुम ! या अच्छे नर हो। तुम सांसारिक जीवन की छोटी-छोटी कठिनाइयों से ही घबरा उठते हो क्योंकि अभी तक तुमने जीवन की बाधाओं का सामना करना सीखा ही नहीं है। यों चिल्लाने लगे या घबराकर बोलने लगे कि जैसे आसमान टूट पड़ेगा अथवा जमीन फट जाएगी। तुम पुरुष हो और वीरता तुम्हारे पास है। एक बार की असफलता से इतनी निराशा। उठो! और निराशा त्यागकर परिस्थितियों का डटकर सामना करो। हमें एक छोटी-सी चींटी से सबक सीखना चाहिए। जब वह चावल का दाना या कोई और वस्तु अपने मुँह में लेकर अपने बिल की ओर जा रही होती है तो उसका मार्ग रोककर देखो या उसके मुँह का दाना या भोजन छीन कर देखो, वह किस प्रकार छटपटाती है और बार-बार उस दाने को प्राप्त करने का प्रयास करती है। वह तब तक निरंतर संघर्ष करती रहेगी, जब तक दाना प्राप्त करके अपने बिल तक नहीं पहुँच जाती। एक ओर मनुष्य है जो थोड़ी-सी या छोटी-सी असफलता पर निराश होकर बैठ जाता है।
2. प्रकृति से प्रेरणा:
‘नर’ अर्थात बुद्धि और बल से परिपूर्ण मनुष्य, प्राणियों में श्रेष्ठ कहलाने वाला है। वह यदि जीवन में आने वाले संघर्ष या कठिनाइयों से मुँह फेरकर और निराश होकर बैठ जाए तो उसकी श्रेष्ठता के कोई मायने नहीं रह जाएँगे। उसे प्रकृति के हर छोटे-बड़े प्राणी से प्रेरणा लेनी चाहिए। यदि वह संघर्ष करना छोड़ देगा और निराश होकर बैठ जाएगा तो संसार में उसका विकास कैसे होगा? हर प्रकार की उन्नति के मार्ग ही बंद हो जाएँगे। इसलिए मनुष्य को अपने नरत्व को सिद्ध करना होगा। उसे निराशा त्याग कर अपने हृदय में उत्साह और साहस भरकर जीवन-पथ को प्रशस्त करना होगा। यदि निराशा ही जीवन का तथ्य होती तो अब तक संसार में जो विकास हुआ है वह कभी संभव न हो पाता।
नरता का पहला लक्षण ही आगे बढ़ना है, संघर्ष करते रहना है। नदियों की बहती धारा की भाँति मनुष्य का भी यही लक्ष्य होना चाहिए। जिस प्रकार धारा सागर में मिलकर ही विश्राम लेती है, उसी प्रकार नर को भी लक्ष्य प्राप्ति के पश्चात ही दम लेना चाहिए। चट्टान भी धारा के मार्ग में आकर उसका रास्ता रोकने का प्रयास करती है। क्या कभी उस धारा को चट्टान रोक सकी? क्योंकि अपने प्रबल प्रवाह से वह चट्टान को किनारे लगाकर अपना रास्ता स्वयं बना लेती है। वह इस कार्य के लिए किसी से सहायता नहीं माँगा करती। झाड़-झंखाड़ों के रोके भी वह कभी नहीं रुकती। बहुत बड़ी चट्टान के मार्ग में आ जाने पर भले ही धारा
थोड़ी देर के लिए रुकी हुई-सी प्रतीत होती है, किंतु वह कुछ ही क्षणों में अपना दूसरा मार्ग खोज लेती है और फिर उसी प्रवाह से अपने लक्ष्य की ओर बढ़ने लगती है। नर (पुरुष) की भी जीवन-पद्धति या कार्यशैली ऐसी ही होनी चाहिए। नर को भी समस्याओं के आने पर अपनी योजना बनाकर उनका सामना करना चाहिए और उन पर सफलता प्राप्त करनी चाहिए। तभी वह नर कहलाने योग्य बन सकता है। समस्याएँ सामने आने पर माथे पर हाथ रखकर तथा निराश होकर बैठ जाने से उसके जीवन की सार्थकता सिद्ध नहीं होती। वह तभी नर कहलाएगा जब वह समस्याओं पर पैर रखकर आगे बढ़ जाएगा। इसे ही सच्चे अर्थों में जीवन कहते हैं तथा यही नर के नरत्व को व्यक्त करता है।
3. निरंतर संघर्ष करना जीवन है:
नर होकर निराश बैठना, यह उसके लिए शोभनीय नहीं है। निराश होकर बैठ जाने और हिम्मत हार जाने वाले मनुष्य की स्थिति वैसी ही होगी जैसी मणि-विहीन सर्प की होती है। मणि ही सर्प की चमक का कारण होती है। उसी प्रकार हिम्मत एवं उत्साह ही मानव-जीवन को सार्थकता प्रदान करने वाले तत्व हैं। जब मनुष्य उनको त्यागकर बैठ जाता है तब वह ‘नर’ कहलाने का अधिकार भी खो बैठता है। ऐसा होना उसके लिए नरता से पतित होने, अपने आपको कहीं का भी नहीं रहने देने के समान होता है। इसलिए कहा गया है कि नर होकर मन को निराश न करो। मनुष्य को किसी भी स्थिति में निराश नहीं होना चाहिए।
निराशा जीवन में अंधकार भर देती है। इससे मनुष्य को कोई मार्ग नहीं सूझ सकता। इसके विपरीत, निराशा को त्यागकर आशावान और आस्थावान होकर जब मनुष्य समस्याओं से जूझता है तो उसका मार्ग स्वतः ही प्रकाशित हो उठता है और वह आगे बढ़ता हुआ अपने लक्ष्य को प्राप्त करने में सफल हो जाता है। संघर्ष और प्रयास में जो आनंद अनुभव होता है वह अमृत के समान होता है। संघर्ष को त्यागकर यदि हम निराशा का दामन पकड़ लेंगे तो उस अमृत के आनंद से भी हाथ धो बैठेंगे। अतः स्पष्ट है नर की नरता निराशा को त्यागकर संघर्ष करने में ही दिखाई देती है। इस पंक्ति का प्रमुख लक्ष्य ही निरंतर संघर्ष करना, गतिशील बने रहना, उत्साह और आनंद का संदेश देना है। इन्हीं से नर की नरता सार्थक होती है।

21. विजयदशमी या दशहरा
भूमिका:
हमारा भारतवर्ष त्योहारों का देश है। यहाँ प्रत्येक बात में धर्म किसी-न-किसी रूप में समाहित है। वैसे तो भारत में पर्यों की संख्या सैकड़ों में है, परंतु इनमें से चार-रक्षा बंधन, दशहरा, दीपावली तथा होली-बहुत बड़े तथा मुख्य पर्व हैं। दशहरा भारत के मुख्य पर्यों में से एक है। इस दिन भगवान् राम ने रावण पर विजय पाई थी। यह अच्छाई की बुराई पर विजय थी। यह त्योहार समस्त भारत में आश्विन शुक्ल पक्ष की दशमी के दिन बड़े उत्साह से मनाया जाता है।
1. ऐतिहासिक आधार:
मर्यादा पुरुषोत्तम राम की जीवन-कथा को सभी भारतीय भली-भांति जानते हैं। अवध के राजा दशरथ की पत्नी कैकेयी की हठ पर राम, लक्ष्मण और सीता को वन में जाना पड़ा। वहाँ रावण ने धोखे से सीता को उठा लिया। भगवान् राम ने हनुमान और सुग्रीव की वानर सेना को साथ लेकर रावण की लंका पर आक्रमण किया और विजय पाई, इसीलिए यह त्योहार विजयदशमी के नाम से प्रख्यात है।
2. उत्सव मनाने की विधि;
विजयदशमी के त्योहार को भारतीय बड़ी धूमधाम से मनाते हैं। कुछ दिन पूर्व से ही नगरों व गाँवों में रामलीला आरंभ हो जाती है तथा नगर के प्रमुख बाजारों में रामचंद्र जी के जीवन को चित्रित करने वाली सुंदर झाँकियाँ निकाली जाती हैं। विजयदशमी को प्रातःकाल से ही बाजारों को विशेष रूप से सजाया जाता है। उस दिन दूर-दूर के गाँवों से भी लोग नगरों में दशहरा देखने आते हैं। दुकानें सजाई जाती हैं। घरों में स्वादिष्ट भोजन बनाए जाते हैं। इस दिन रावण, कुम्भकरण, मेघनाथ के कागज़ों और बाँसों द्वारा बने हुए बड़े-बड़े पुतलों को आग लगाते हैं। इसी दृश्य को देखने के लिए वहाँ लाखों की संख्या में लोग जमा होते हैं। पुतलों में पटाखे भरे होते हैं। आग लगते ही वे बजने लगते हैं।
3. कुल्लू का दशहरा:
कुल्लू का दशहरा विशेष रूप से प्रसिद्ध है। यहाँ एक सप्ताह पूर्व से ही दशहरे की तैयारियाँ आरंभ हो जाती हैं। नर और नारियाँ तुरहियाँ, बिगुल, ढोल, नगाड़े, बाँसुरी और घन्टियों के तुमुल नाद के मध्य नाच करते हुए कन्धों पर देवगण को उठाकर नगर की परिक्रमा करते हुए कुल्लू नगर के देवता रघुनाथ जी की वन्दना से दशहरे का पर्व आरंभ करते हैं और अन्तिम दिन दोपहर बाद समस्त देवगण रघुनाथ जी के चारों ओर जोशपूर्ण परिक्रमा करते हैं। तत्पश्चात् युद्ध में बाजों के साथ लंका पर चढ़ाई की जाती है और ब्यास नदी के किनारे काँटों के ढेर की लंका जलाकर नष्ट कर दी जाती है।
4. शिक्षा:
हर त्योहार से कोई-न-कोई शिक्षा अवश्य मिलती है। दशहरे से हमें यह शिक्षा मिलती है कि चाहे कोई कितना विद्वान् क्यों न हो अगर उसमें अहंकार आ गया तो उसके सभी गुण फीके पड़ जाते हैं। रावण जैसे विद्वान् लोग भी मर्यादा रहित बन जाते हैं। उनका अंत भी बुरा ही होता है। अतः स्पष्ट है कि हमें कभी घमण्डी नहीं बनना चाहिए और सच्चाई के मार्ग पर चलते हुए कठिनाइयों से घबराना नहीं चाहिए। उपसंहार-दशहरा पर्व हमें बुराई को त्यागकर पुण्य-कार्य करने की प्रेरणा देता है। कवि का कथन है-
पाप तिमिर सब मिट जाता है,
सत्य का होता जब भी प्रकाश।
सुसंदेश दशहरा पर्व का
अन्यायी का होता है नाश ॥

22. दीपावली
भूमिका:
भारत त्योहारों का देश है। प्रत्येक ऋतु में किसी-न-किसी त्योहार को मनाया जाता है। भारत में फसलों के पकने पर भी त्योहार मनाए जाते हैं। महापुरुषों के जन्मदिन को भी त्योहारों की भांति बड़े उत्साह से मनाया जाता है। भारत के मुख्य त्योहारों-रक्षा बंधन, दशहरा, दीपावली और होली आदि में दीपावली सर्वाधिक प्रसिद्ध त्योहार है। इसे बड़े जोश एवं उत्साह के साथ मनाया जाता है। दीपावली का अर्थ है-‘दीपों की पंक्ति’ । यह त्योहार कार्तिक मास की अमावस्या को मनाया जाता है। अमावस्या की रात बिल्कुल अंधेरी होती है किंतु भारतीय घर-घर में दीप जलाकर उसे पूर्णिमा से भी अधिक उजियाली बना देते हैं। इस त्योहार का बड़ा महत्त्व है।
1. ऐतिहासिक आधार:
इस त्योहार का ऐतिहासिक आधार अति महत्त्वपूर्ण एवं धार्मिक है। इस दिन भगवान् राम लंका-विजय करके अपना वनवास समाप्त करके लक्ष्मण और सीता सहित जब अयोध्या आए तो नगरवासियों ने अति हर्षित होकर उनके स्वागत के लिए रात्रि को नगर में दीपमाला करके अपने आनंद और प्रसन्नता को प्रकट किया। दीपावली का त्योहार आर्यसमाजी, जैनी और सिक्ख लोग भी विभिन्न रूपों में बड़े उत्साह से मनाते हैं। इसी दिन आर्यसमाज के संस्थापक स्वामी दयानंद सरस्वती ने महासमाधि ली थी और इसी दिन जैन धर्म के तीर्थंकर महावीर स्वामी ने निर्वाण प्राप्त किया था। इसी प्रकार सिक्ख अपने छठे गुरु की याद में इस त्योहार को मनाते हैं जिन्होंने इसी दिन बंदी गृह से मुक्ति प्राप्त की थी।
2. दीपावली पर्व की तैयारी:
इस पर्व की तैयारी घरों की सफाई से आरंभ होती है जो कि लोग एक-आध मास पूर्व आरंभ कर देते हैं। लोग शरद् ऋतु के आरंभ में घरों की लिपाई-पुताई करवाते हैं और कमरों को चित्रों से अलंकृत करते हैं। इससे मक्खी मच्छर दूर हो जाते हैं। इससे कुछ दिन पूर्व अहोई माता का पूजन किया जाता है। धन त्रयोदशी के दिन लोग पुराने बर्तन बेचते हैं और नए खरीदते हैं। चतुर्दशी को लोग घरों का कूड़ा-करकट बाहर निकालते हैं। लोग कार्तिक मास की अमावस्या को दीपमाला करते हैं।
3. मनाने की विधि:
इस दिन कई लोग अपने इष्ट सम्बन्धियों में मिठाइयाँ बाँटते हैं। बच्चे नए-नए वस्त्र पहनकर बाजार जाते हैं। रात को पटाखे तथा आतिशबाजी चलाते हैं। बहुत-से लोग रात को लक्ष्मी पूजा करते हैं। कई लोगों का विचार है कि इस रात लक्ष्मी अपने श्रद्धालुओं के घर जाती है। इसलिए प्रायः लोग अपने घर के द्वार उस रात बंद नहीं करते ताकि लक्ष्मी लौट न जाए। व्यापारी लोग वर्षभर के खातों की पड़ताल करते हैं और नई बहियाँ लगाते हैं।
4. कुप्रथा:
दीपावली के दिन जहाँ लोग शुभ कार्य एवं पूजन करते हैं वहाँ कुछ लोग जुआ भी खेलते हैं। जुआ खेलने वाले लोगों का विश्वास है कि यदि इस दिन जुए में जीत गए तो फिर वर्ष भर जीतते रहेंगे तथा लक्ष्मी की उन पर कृपा बनी रहेगी। कहीं-कहीं पटाखों को लापरवाही से बजाते समय बच्चों के हाथ-पाँव भी जल जाते हैं और कहीं-कहीं पटाखों के कारण आग लगने की दुर्घटना भी होती है। अतः इस पावन पर्व को हमें सदा सावधानी से. मनाना चाहिए।
उपसंहार:
दीपावली का त्योहार मानव जाति के लिए शुभ काम करने की प्रेरणा देने वाला है। जैसे दीपक जल कर अंधकार को समाप्त करके प्रकाश फैला देता है, वैसे ही दीपावली भी अज्ञानता के अंधकार को हटाकर ज्ञान का प्रकाश हमारे मन में भर . देती है। देश और जाति की समृद्धि का प्रतीक यह त्योहार अत्यंत पावन और मनोरम है।
23. होली अथवा रंगों का त्योहार
भूमिका:
भारत त्योहारों और पर्वो का देश है। यहाँ हर वर्ष अनेक त्योहार मनाए जाते हैं। रक्षाबंधन, दीपावली, दशहरा, होली आदि यहाँ के सुप्रसिद्ध त्योहार हैं। अन्य पर्यों की भांति होली भी भारत का प्रमुख पर्व है। यह भारतीय जीवन का अभिन्न अंग बन चुका है। यह त्योहार फाल्गुन मास की पूर्णिमा के दिन मनाया जाता है। यह त्योहार समाज की एकता, मेल-जोल और प्रेम भावना का प्रतीक माना जाता है।
1. इतिहास:
होली के त्योहार का इतिहास अत्यंत प्राचीन है। इसका संबंध राजा हिरण्यकश्यप से है। हिरण्यकश्यप निर्दयी और नास्तिक राजा था। वह अपने-आपको भगवान मानता था तथा चाहता था कि प्रजा उसे परमात्मा से भी बढ़कर समझे तथा उसकी पूजा करे। उसके पुत्र एवं ईश्वर-भक्त प्रह्लाद ने उसका विरोध किया। इस पर क्रोधित होकर उसने उसे मार डालने का प्रयास किया। हिरण्यकश्यप की एक बहन होलिका थी। उसे वरदान था कि अग्नि उसके शरीर को जला नहीं सकती। लकड़ियों के एक ढेर पर होलिका प्रह्लाद को अपनी गोद में लेकर बैठ गई और फिर लकड़ी के ढेर को आग लगा दी गई। होलिका जलकर राख हो गई पर प्रह्लाद ज्यों-का-त्यों बैठा रहा। इसलिए लोग इस घटना को याद करके होली मनाते हैं।
2. कृषि का पर्व:
होली का त्योहार मनाने का एक और कारण भी है। हमारा देश कृषि प्रधान देश है। फरवरी और मार्च के महीने में गेहूँ और चने के दाने अधपके हो जाते हैं। इनको देखकर किसान खुशी से झूम उठता है। अग्नि देवता को प्रसन्न करने के लिए वह गेहूँ के बालों की अग्नि में आहुति देता है।
3. रंग का त्योहार:
होली को रंगों का त्योहार भी कहा जाता है, क्योंकि इस दिन लोग रंग, गुलाल, अबीर आदि से त्योहार मनाते हैं। लोग एक-दूसरे पर रंग डालते हैं तथा एक-दूसरे के चेहरे पर गुलाल लगाते हैं। इस अवसर पर लोगों के चेहरे एवं वस्त्र रंग-बिरंगे हो जाते हैं। चारों ओर मस्ती का वातावरण छा जाता है। वास्तव में यह त्योहार कई दिन पूर्व ही आरंभ हो जाता है।
यह त्योहार परस्पर भाई-चारे और प्रेम-भाव का त्योहार है। इस अवसर पर कई लोग रात को लकड़ियाँ जलाकर होली की पूजा करते हैं। उसके बाद गाना बजाना करते हैं। कविवर मैथिलीशरण गुप्त का कथन है-
काली-काली कोयल बोली होली, होली, होली,
फूटा यौवन फाड़ प्रकृति की पीली-पीली चोली।
4. कुप्रथा:
इस शुभ त्योहार पर कुछ लोग एक-दूसरे पर मिट्टी, कीचड़, पानी आदि फेंकते हैं। यह उचित काम नहीं है। इससे किसी भी पर्व का महत्त्व कम हो जाता है। ऐसा करने से कभी-कभी लड़ाई-झगड़े तक हो जाते हैं तथा वैर भावना जन्म ले लेती है। कुछ लोग इस अवसर पर शराब आदि नशीले पदार्थों का सेवन करते हैं। इन सब बुराइयों के कारण ऐसे शुभ त्योहार अपने सच्चे महत्त्व एवं अर्थ को खो बैठते हैं। अतः यह आवश्यक है कि हम इस त्योहार को उचित ढंग से मनाएँ तथा हमें उसके महत्त्व को समझने का प्रयास करना चाहिए।
उपसंहार:
सार रूप में कहा जा सकता है कि होली एक आनंदमय एवं मस्ती भरा त्योहार है। यह प्रकृति एवं कृषि का त्योहार है। हमें इसकी पवित्रता एवं महत्त्व को समझना चाहिए तथा उनकी रक्षा करनी चाहिए। इस पर्व पर गोष्ठियों का आयोजन करके हमें समाज में एकता और प्रेम को बढ़ाना चाहिए।
24. रक्षा-बंधन
भूमिका:
रक्षा-बंधन भारत का पवित्र एवं प्रमुख त्योहार है। यह भारतीय लोक जीवन की सुंदर परंपरा है। यह पर्व श्रावण मास की पूर्णिमा को मनाया जाता है। प्राचीन आश्रमों में स्वाध्याय के लिए यज्ञ और ऋषियों के लिए तर्पण कर्म करने के कारण इसका ‘उपाकर्म’ नाम पड़ा। यज्ञ के उपरान्त ‘रक्षा-सूत्र’ बाँधने की प्रथा के कारण इसका नाम ‘रक्षा-बंधन’ लोक-प्रचलित हो गया। संस्कृत शब्द रक्षा का हिंदी रूप ही ‘राखी’ है इसलिए कुछ लोग इसे राखी का त्योहार भी कहते हैं।
1. इतिहास:
रक्षा-बंधन’ पर्व के रूप में कब आरंभ हुआ इस संबंध में कोई निश्चित प्रमाण उपलब्ध नहीं है। कहा जाता है कि एक बार देवताओं और राक्षसों के बीच युद्ध हुआ था। युद्ध में देवताओं का पक्ष कमजोर पड़ता जा रहा था। तब इन्द्र की पत्नी ने अपने पति की मंगल कामना हेतु उन्हें राखी बाँधकर युद्ध में भेजा था। राखी के प्रभाव से युद्ध में इन्द्र की विजय हुई। तब से राखी के त्योहार के महत्त्व को स्वीकार किया जाने लगा।
मुसलमानों के शासनकाल में यवन सुंदर कन्याओं को उठा लेते थे। इस विपत्ति से बचने के लिए कन्याएँ बलवान राजा को एक धागा भेजकर अपना भाई बना लेती थीं। इस प्रकार से वे अपनी मान-मर्यादा की रक्षा करती थीं। मेवाड़ की रानी कर्मवती ने भी हुमायूँ को ऐसे ही राखी भेजकर भाई बनाया था।
2. मनाने की विधि:
आज बहन या धर्म-बहन अपने भाई के माथे पर तिलक लगाकर उसके हाथ पर राखी बाँधती है। भाई बहन से राखी बँधवाकर उसकी रक्षा का भार अपने ऊपर ले लेता है। कुछ लोग इस अवसर पर पूजा-अर्चना भी करते हैं। इस अवसर पर भाई अपनी बहन को स्नेह के रूप में वस्त्र और धन भी देता है।
3. प्रेम का प्रतीक:
रक्षा-बंधन वस्तुतः भाई-बहन के प्रेम का प्रतीक है। यह सामाजिक कल्याण का सूत्र एवं बहन के निश्छल, सरल और पवित्र स्नेह का प्रतीक है
“कच्चे धागों में बहनों का प्यार है।
देखो, राखी का आया त्योहार है ॥”
बहन के अतिरिक्त ब्राह्मण अपने यजमानों को राखी बाँधते हैं। ब्राह्मण अपने यजमान को आशीर्वाद भी देता है। बदले में यजमान ब्राह्मण को दक्षिणा देता है।
4. धार्मिक महत्त्व:
धार्मिक क्षेत्र में रक्षा-बंधन का महत्त्व कई प्रकार से माना जाता है। इसी पवित्र दिन भगवान् विष्णु को वामन अवतार के रूप में दानव राजा बलि को तीन पग भूमि माँगने पर सारा राज्य दान करना पड़ा। इस महान् त्याग की स्मृति बनकर यह शुभ त्योहार आता है। दान-पुण्य के कारण ब्राह्मणों के लिए यह त्योहार और भी महत्त्वपूर्ण बन जाता है। .
उपसंहार:
रक्षा-बंधन’ पर्व की ऐतिहासिक पृष्ठभूमि कुछ भी रही हो किंतु आज यह त्योहार भाई-बहन के पवित्र त्योहार के रूप में मनाया जाता है। इस त्योहार से हमें समाज कल्याण एवं समाज-रक्षा की प्रेरणा मिलती है। हमें न केवल अपनी बहन की रक्षा करनी चाहिए अपितु समाज के हर कमजोर व्यक्ति की रक्षा करना हमारा कर्त्तव्य बनता है।

25. परिश्रम का महत्त्व
भूमिका:
मानव जीवन में परिश्रम का विशेष महत्त्व है। मानव तो क्या, प्रत्येक प्राणी के लिए परिश्रम का महत्त्व है। चींटी का छोटा-सा जीवन भी परिश्रम से पूर्ण है। मानव परिश्रम द्वारा अपने जीवन की प्रत्येक समस्या को सुलझा सकता है। यदि वह चाहे तो पर्वतों को काटकर सड़क निकाल सकता है, नदियों पर पुल बाँध सकता है, काँटेदार मार्गों को सुगम बना सकता है और समुद्रों की छाती को चीरकर आगे बढ़ सकता है। ऐसा कौन-सा कार्य है जो परिश्रम से न हो सके। नेपोलियन ने भी अपनी डायरी में लिखा था-‘असंभव’ जैसा कोई शब्द नहीं है। कर्मवीर तथा दृढ़-प्रतिज्ञ महापुरुषों के लिए संसार का कोई भी कार्य कठिन नहीं होता।
1. भाग्य का सहारा:
कुछ लोग ऐसे भी हैं जो भाग्य पर निर्भर रहकर श्रम को छोड़ देते हैं। वे भाग्य का सहारा लेते हैं परंतु भाग्य जीवन में आलस्य को जन्म देता है और यह आलस्य जीवन को अभिशापमय बना देता है। आलसी व्यक्ति दूसरों पर निर्भर रहता है। ऐसा व्यक्ति हर काम को भाग्य के भरोसे छोड़ देता है। हमारा देश इसी भाग्य पर निर्भर रहकर सदियों तक गुलामी को भोगता रहा। हमारे अंदर हीनता की भावना घर कर गई लेकिन जब हमने परिश्रम के महत्त्व को समझा तब हमने स्वतंत्रता की ज्योति जलाई और पराधीनता की बेड़ियों को तोड़ डाला। संस्कृत के कवि भर्तृहरि ने ठीक ही कहा है-
उद्योगिनं पुरुषसिंहमुपैति लक्ष्मीः देवेन देयमिति का पुरुषा वदन्ति।
दैवं निहत्य करु पौरुषमात्माशक्तया, यत्ने कृते यदि न सिध्यति कोऽत्र दोषः॥
अर्थात् उद्यमी पुरुष को लक्ष्मी प्राप्त होती है। ‘ईश्वर देगा’ ऐसा कायर आदमी कहते हैं। दैव अर्थात् भाग्य को छोड़कर मनुष्य को यथाशक्ति पुरुषार्थ करना चाहिए। यदि परिश्रम करने पर भी कार्य सिद्ध न हों तो सोचना चाहिए कि इसमें हमारी क्या कमी रह गई है।
केवल ईश्वर की इच्छा और भाग्य के सहारे पर चलना कायरता है। यह अकर्मण्यता है। मनुष्य अपने भाग्य का विधाता स्वयं है। अंग्रेज़ी में भी कहावत है-“God helps those who help themselves” अर्थात् ईश्वर भी उन्हीं की सहायता करता है जो अपनी सहायता आप करते हैं। कायर और आलसी व्यक्ति से तो ईश्वर भी घबराता है। कहा भी गया है-‘दैव-दैव आलसी पुकारा’ । संस्कृत की ही उक्ति है-‘श्रमेव जयते’ अर्थात् परिश्रम की ही विजय होती है। वस्तुतः मानव प्रकृति का सर्वश्रेष्ठ प्राणी है। वह स्वयं ईश्वर का प्रतिरूप है। संस्कृत का एक श्लोक है-
उद्यमेन हि सिध्यन्ति कार्याणि न मनोरथैः।
न हि सुप्तस्य सिंहस्य प्रविशन्ति मुखे मृगाः॥ इसका अर्थ यह है कि उद्यम से ही मनुष्य के कार्य सिद्ध होते हैं, केवल इच्छा से नहीं। जिस प्रकार सोए हुए शेर के मुँह में मृग स्वयं नहीं प्रवेश करते, उसी प्रकार से मनुष्य को भी कर्म द्वारा सफलता मिलती है। कर्म से मानव अपना भाग्य स्वयं बनाता है। एक कर्मशील मानव जीवन की सभी बाधाओं और कठिनाइयों पर विजय प्राप्त कर लेता है।
2. परिश्रम के लाभ:
परिश्रम से मनुष्य का हृदय गंगाजल के समान पावन हो जाता है। परिश्रम से मन की सभी वासनाएँ और दूषित भावनाएँ बाहर निकल जाती हैं। परिश्रमी व्यक्ति के पास बेकार की बातों के लिए समय नहीं होता। कहा भी गया है”खाली मस्तिष्क शैतान का घर है।” यही नहीं, परिश्रम से आदमी का शरीर हृष्ट-पुष्ट होता है। उसके शरीर को रोग नहीं सताते। परिश्रम से यश और धन दोनों प्राप्त होते हैं। ऐसे लोग भी देखे गए हैं जो भाग्य के भरोसे न रहकर थोड़े-से धन से काम शुरू करते हैं- और देखते-ही-देखते धनवान बन जाते हैं। परिश्रमी व्यक्ति को जीवित रहते हुए भी यश मिलता है और मरने के उपरांत भी। वस्तुतः परिश्रम द्वारा ही मानव अपने को, अपने समाज को और अपने राष्ट्र को ऊँचा उठा सकता है। जिस राष्ट्र के नागरिक परिश्रमशील हैं, वह निश्चय ही उन्नति के शिखर को स्पर्श करता है लेकिन जिस राष्ट्र के नागरिक आलसी और भाग्यवादी हैं, वह शीघ्र ही गुलाम हो जाता है।
3. महापुरुषों के उदाहरण:
हमारे सामने ऐसे अनेक महापुरुषों के उदाहरण हैं जिन्होंने परिश्रम द्वारा अपना ही नहीं अपितु अपने राष्ट्र का नाम भी उज्ज्वल किया है। अब्राहिम लिंकन का जन्म एक गरीब परिवार में हुआ था लेकिन निरंतर कर्म करते हुए वे झोंपड़ी से निकलकर अमेरिका के राष्ट्रपति भवन तक पहुँचे। भूतपूर्व प्रधानमंत्री श्री लालबहादुर शास्त्री, भूतपूर्व राष्ट्रपति डॉ० सर्वपल्ली राधाकृष्णन, राष्ट्रपिता महात्मा गांधी, महामना मदन मोहन मालवीय, नेता जी सुभाषचंद्र बोस आदि महापुरुष इस बात के साक्षी हैं कि परिश्रम से ही व्यक्ति महान बनता है।
उपसंहार:
यदि हम चाहते हैं कि अपने देश की, अपनी जाति की और अपनी स्वयं की उन्नति करें तो यह आवश्यक है कि हम भाग्य का सहारा छोड़कर परिश्रमी बनें। आज देश के युवाओं में जो बेरोज़गारी और आलस्य व्याप्त है, उसका भी एक ही इलाज है-परिश्रम। संस्कृत में भी ठीक कहा गया है-
श्रमेण विना न किमपि साध्यं ।
26. साहित्य और समाज
भूमिका:
आज अधिकांश विद्वान साहित्य और समाज में गहन संबंध मानते हैं। उनका मत है कि साहित्य और समाज का अविच्छिन्न संबंध है। ये दोनों एक-दूसरे पर आश्रित रहते हैं। समाज यदि शरीर है तो साहित्य उसकी आत्मा है। साहित्य मानव के मस्तिष्क की देन है। मानव भी तो सामाजिक प्राणी है। समाज के बिना तो वह अधूरा है। उसका पालन-पोषण, शिक्षा-दीक्षा आदि सब कुछ समाज में ही होता है। वह समाज में रहता हुआ ही विभिन्न अनुभूतियाँ अर्जित करता है। अतः उसमें एक नैसर्गिक इच्छा होती है कि वह अपनी भावनाओं को संसार के समक्ष अभिव्यक्त करे। उसकी यह लालसा ही साहित्य को जन्म देती है।
1. साहित्य की परिभाषा एवं अर्थ:
संस्कृत में साहित्य शब्द की परिभाषा इस प्रकार की गई है-‘हितेन सहितं साहित्यं’ अथवा ‘सहितस्य भावः साहित्यं । इसके अनुसार साहित्य का अर्थ है-जिसमें हित की भावना हो अर्थात साहित्य समाज के कल्याण या हित के लिए रचा जाता है। अपने हित-अहित का ज्ञान तो पशु को भी होता है। गोस्वामी तुलसीदास ने भी कहा है, ‘हित अनहित पशु पक्षिहुँ जाना’, फिर मनुष्य तो बुद्धिजीवी प्राणी है। उसे तो अपने हित की हमेशा चिंता रहती है। मनुष्य की भाँति ही साहित्य संपूर्ण समाज की चिंता करता है किंतु मनुष्य और साहित्य के हित-चिंतन में पर्याप्त अंतर है। मनुष्य का हित-चिंतन ‘स्व’ पर आधारित है परंतु साहित्य का हित-चिंतन विश्व-कल्याण की भावना पर आधारित है। साहित्य की परिभाषा देते हुए डॉ० महावीर प्रसाद द्विवेदी ने कहा है, “ज्ञान की संचित राशि का नाम साहित्य है।” डॉ० श्यामसुंदर दास का कहना है-सामाजिक मस्तिष्क अपने पोषण के लिए जो भाव सामग्री निकालकर समाज को सौंपता है, उसके संचित भंडार का नाम साहित्य है। मनुष्य की सामाजिक स्थिति के विकास में साहित्य का प्रधान योग रहता है।
2. साहित्य समाज का दर्पण:
साहित्य को सामग्री प्रदान करने वाला तो सामाजिक जीवन ही है। समाज का वातावरण भी साहित्य को प्रभावित करता है। एक साहित्यकार अपने जीवन के चारों ओर के वातावरण, वस्तुओं, घटनाओं आदि को देखता है। उनमें से कुछ उसे प्रभावित करती हैं। साहित्यकार इन बातों को अपने अंतर्मन में संजो लेता है। वे अनुभूतियाँ कवि की निजी अनुभूतियाँ बन जाती हैं। बाद में कवि उन्हीं अनुभूतियों को अपनी रचनाओं में अभिव्यक्त करता है। प्रसिद्ध पाश्चात्य आलोचक टी०एस० इलियट का तो यहाँ तक कहना है, “कवि और उसकी रचना एक-दूसरे को प्रभावित करते हैं। कवि कुछ देता है तो कुछ लेता भी है। कवि जो कुछ लिखता है, वह समाज से लेता है और समाज को वापिस सौंप देता है। समाज के जीवन को उत्साहित करने वाला साहित्य ही सत्साहित्य कहा जाता है।”
3. समाज का साहित्य पर प्रभाव:
सुप्रसिद्ध अंग्रेज़ विद्वान मैथ्यू आर्नोल्ड ने साहित्य को जीवन और समाज का दर्पण कहा है। वस्तुतः साहित्यकार जिस युग या परिवेश में रहता है, उसकी उपलब्धियाँ, चेतनाएँ, स्थितियाँ और समस्याएँ उसके मन-मस्तिष्क को सदैव प्रभावित किए रहती हैं। साहित्य उन्हीं प्रभावों का कलात्मक रूप है। कवि या लेखक अपने युग और उसकी परिस्थितियों का चितेरा होता है। समाज से उसे जैसी सामग्री मिलती है, उसका वह अपने दृष्टिकोण से उपयोग करता है। कवि उस बात को कहता है जिसको सब लोग अनुभव तो करते हैं लेकिन कह नहीं सकते। कवि समाज के दुःख-दर्द और उसकी समस्याओं को सशक्त एवं सजीव रूप में प्रस्तुत करता है।
साहित्य तत्कालीन वातावरण और परिस्थितियों का सच्चा चित्र अथवा प्रतिबिंब प्रस्तुत करता है। समाज के रीति-रिवाजों, आस्था और विश्वास तथा सांस्कृतिक परंपराओं का साहित्य में पर्याप्त वर्णन मिलता है। यदि हम हिंदी साहित्य के इतिहास का सिंहावलोकन करें तो पाएँगे कि आदिकाल में युद्धों का वातावरण था। भक्तिकाल में भक्ति संबंधी धारा प्रवाहित हुई और रीतिकाल में शृंगार रस की प्रधानता थी।
4. साहित्य का समाज पर प्रभाव:
साहित्य समाज का दर्पण है। यह साहित्य के विषय में अधूरा सत्य है। जिस प्रकार साहित्य पर समाज के भावों एवं वातावरण का प्रभाव पड़ता है, उसी प्रकार समाज भी साहित्य द्वारा प्रसारित भावों से प्रभावित होता है। चूंकि कवि और लेखक समाज के प्रतिनिधि होते हैं, अतः वे समाज को नए भाव और नए विचार प्रदान करते हैं। समाज पर जब-जब संकट के बादल छाए हैं, तब-तब साहित्यकारों ने लोगों को प्रेरणा देकर उन्हें कर्तव्यनिष्ठ बनाया है। इतिहास इस बात का साक्षी है कि जब-जब समाज की नाव डगमगाने लगी, तब-तब साहित्यकारों ने उसे संभाला और साहित्य-सजन द्वारा समाज को नई गति तथा नया आलोक प्रदान किया। उदाहरणार्थ, प्रेमचंद के उपन्यासों और कहानियों ने भारत के किसानों के प्रति सहानुभूति जागृत करने में बहुत योगदान दिया है।
उपसंहार:
निष्कर्ष रूप में कहा जा सकता है कि साहित्य और समाज में भिन्नता होते हुए भी एकता है। साहित्यकार समाज को उसका उचित श्रेय प्रदान करता है। एक के कमज़ोर होते ही दूसरे का प्रभाव पड़ना स्वाभाविक है। वस्तुतः ये दोनों परस्पर सापेक्ष हैं। साहित्य का लक्ष्य समाज के दोषों को दूर कर उसे संस्कारित करके पूर्ण बनाना है। अतः समाज के संपर्क में विकसित होने वाले साहित्य का ही अधिक महत्त्व होता है। जो साहित्य समाज से अलग रहकर फलता-फूलता है, उसका कोई महत्त्व नहीं होता। ऐसा साहित्य कुछ समय पश्चात समाप्त हो जाता है। लोक-चेतना के समीप रहने से ही साहित्य में सजीवता, स्फूर्ति एवं गतिशीलता का समावेश हो सकता है। अतः यह स्वतः सिद्ध है कि साहित्य समाज की प्रतिध्वनि होता है क्योंकि किसी देश के साहित्य को पढ़कर वहाँ की संस्कृति, विचारधारा, विश्वास, आचार-विचार, सभ्यता आदि का परिचय मिल सकता है। अतः साहित्य और समाज का परस्पर अत्यंत गहन संबंध है। साहित्य समाज का दर्पण होते हुए भी उसका पथ-प्रदर्शक होता है।
27. समाचार-पत्रों के लाभ तथा हानियाँ
भूमिका:
मनुष्य एक सामाजिक प्राणी है। समाज में मनुष्य अकेला नहीं रह सकता। विज्ञान ने यदि मनुष्य को अपना दास बनाया है तो सारी वसुधा को एक कुटुम्ब भी बना दिया है। समाचार-पत्रों के द्वारा मनुष्य समस्त वसुधा की जानकारी प्राप्त करता है। समाचार-पत्र ज्ञान-वर्द्धन का सबसे सस्ता, सरल और प्रमुख साधन है। भारत जैसे विशाल देश में विचारों के आदान-प्रदान के लिए समाचार-पत्र विशेष भूमिका निभाते हैं। समाचार-पत्र देश की रीढ़ की हड्डी होते हैं। किसी भी देश को कमजोर एवं शक्तिशाली बनाने में समाचार-पत्रों की विशेष भूमिका होती है। वर्तमान युग में समाचार-पत्र प्रजातंत्र का आधार-स्तम्भ हैं, उसके जागरूक प्रहरी हैं। समाचार-पत्र जनजीवन की गतिविधियों का दर्पण हैं। भारत में सर्वप्रथम 1780 में कलकत्ता में एक पत्र प्रकाशित हुआ। उसके पश्चात् राष्ट्रीय आन्दोलन के साथ-साथ भारत में पत्रकारिता का भी प्रचार होता गया।
1. समाचार-पत्र के लाभ:
मानवीय कौतूहल एवं जिज्ञासा को शांत करने में समाचार-पत्र विशेष भूमिका निभाते हैं। देश-विदेश की घटनाओं की जानकारी प्राप्त करके मानव आत्म-तुष्टि अनुभव करता है। शासकीय, व्यापारिक एवं खेलकूद के समाचार भी मनुष्य समाचार-पत्रों से प्राप्त करता है। समाचार-पत्रों से ही मनुष्य सरकारी आज्ञा, निर्देश और सूचनाओं को जानता है। सामाजिक, धार्मिक, राजनीतिक, सांस्कृतिक, आर्थिक तथा सिने-संसार की गतिविधियों की जानकारी भी मानव को समाचार-पत्रों से ही मिलती है।
2. व्यापार वृद्धि में योगदान:
आधुनिक युग में व्यापारिक उन्नति भी समाचार-पत्रों पर ही निर्भर है। व्यापार मंडियों के भाव, शेयरों आदि के उतार-चढ़ाव की जानकारी भी मनुष्य को समाचार-पत्रों से मिलती है। समाचार-पत्र लाखों, करोड़ों के हाथों में पहुँचते हैं और वस्तु के विक्रय में सहायता करते हैं।
3. नौकरी प्राप्त करने में समाचार:
पत्रों की भूमिका-नौकरी के लिए कहाँ-कहाँ स्थान खाली हैं, इसकी जानकारी भी समाचार-पत्र ही देते हैं। इस प्रकार समाचार-पत्र के विज्ञापन बेरोजगार युवाओं को उनके भविष्य की जानकारी करवाते हैं। इसके साथ ही यह लाखों हॉकरों को रोजी-रोटी देते हैं। कहानियाँ, चुटकुले, लेख, व्यंग्य आदि छापकर यह मनुष्य के मनोरंजन का साधन भी बनता है। इसके अतिरिक्त समाचार-पत्र सामाजिक कुरीतियों और धार्मिक अंधविश्वासों को दूर करने में भी विशेष भूमिका निभाते हैं।
4. समाचार-पत्र की हानियाँ:
संसार में लाभ-हानि का चोली-दामन का संबंध है। समाचार-पत्रों से जहाँ लाभ हैं, वहाँ कुछ हानियाँ भी हैं। पीत-पत्रकारिता, मन गढ़न्त और झूठे समाचार, किसी राजनीतिक दल की गलत विचारधारा का प्रचार और अन्धानुकरण आदि देश को विनाश की ओर ले जाते हैं। अश्लील विज्ञापन बच्चों पर बुरा प्रभाव डालते हैं।
उपसंहार:
समाचार-पत्र का मुख्य उद्देश्य मनुष्य के समक्ष सत्य घटनाओं को व्यक्त करना होना चाहिए। विशिष्ट ज्ञानवर्द्धक सामग्री प्रस्तुत करना ही इसका उद्देश्य होना चाहिए। इसे पढ़ने से मनुष्य के संकीर्ण विचार दूर होकर उसे उदार बनाते हैं। सभी देशों की घटनाएँ पढ़ने से मानव में ‘वसुधैव कुटुम्बकम्’ की भावना जागृत होती है। समाचार-पत्र समाज में जागृति लाने, युवकों को देश-भक्ति और बलिदान की प्रेरणा देने एवम् समाज में नारी की स्थिति को सुधारने में महत्त्वपूर्ण भूमिका निभाते हैं।

28. आदर्श अध्यापक
भूमिका:
मानव-जीवन एक यात्रा के समान है। यदि यात्री को यह ज्ञात हो कि उसे कहाँ जाना है तो वह अपनी दिशा की ओर अग्रसर होना शुरू हो जाता है किंतु यदि उसे अपने गंतव्य का ज्ञान नहीं होता तो उसकी यात्रा निरर्थक हो जाती है। इसी प्रकार, यदि एक विद्यार्थी को ज्ञान हो कि उसे क्या करना है तो वह उसी दिशा में प्रयत्न करना आरंभ कर देगा और सफलता भी प्राप्त करेगा। इसके विपरीत, उद्देश्यहीन अध्ययन उसे कहीं नहीं ले जाता।
1. जीवन लक्ष्य का चुनाव अनिवार्य:
ऊपर बताया गया है कि जीवन एक यात्रा के समान है। मैं भी जीवन रूपी चौराहे पर खड़ा हूँ। यहाँ से कई मार्ग अलग-अलग दिशाओं को जा रहे हैं। मेरे समक्ष अनेक सपने हैं और लोगों के अनेक सुझाव भी हैं। कोई कहता है कि अध्यापक का कार्य सर्वोत्तम है क्योंकि अध्यापक राष्ट्र-निर्माता है। कोई कहता है कि सेना में भर्ती होकर देश की सेवा करो। कोई कहता है, नहीं, इंजीनियर बनो, इसी में तुम्हारी भलाई है। कभी-कभी मन में यह भी आता है कि एक सफल व्यापारी बनूँ। फिर सोचता हूँ कि व्यापार में ईमानदारी नहीं। मेरे कुछ साथी भी हैं, वे भी अपने मन में कुछ सपने पाल रहे हैं। कोई डॉक्टर बनकर अधिकाधिक रुपया कमाना चाहता है तो कोई उद्योगपति बनना चाहता है। कुछ ऐसे भी हैं जो राजनीति में प्रवेश करके सत्ता प्राप्त करना चाहते हैं। कोई अभिनेता बनना चाहता है तो कोई लेखक बनकर यश प्राप्त करना चाहता है।
2. मेरा जीवन लक्ष्य अध्यापक बनना:
जीवन में लक्ष्य का चुनाव अपनी इच्छाओं एवं अपने साधनों के अनुरूप करना चाहिए। ध्येय चुनते समय स्वार्थ और परमार्थ में समन्वय रखना चाहिए। अपनी रुचि एवं प्रवृत्ति को भी सामने रखना चाहिए। दूसरों का अंधानुकरण करना उपयुक्त नहीं। मेरे जीवन का भी एक लक्ष्य है। मैं एक आदर्श शिक्षक बनना चाहता हूँ। भले ही लोग इसे एक सामान्य लक्ष्य कहें परंतु मेरे लिए यह एक महान लक्ष्य है जिसकी पूर्ति के लिए मैं भगवान से नित्य प्रार्थना करता हूँ। अध्यापक बनकर भारत का भार उठाने वाले भावी नागरिक तैयार करना मेरी महत्वाकांक्षा है।
3. अध्यापन एक महान कार्य:
आज की शिक्षण व्यवस्था और शिक्षकों को देखकर मेरा मन निराश हो जाता है। आज शिक्षक शिक्षा का सच्चा मूल्य नहीं समझते। वे शिक्षा को एक व्यवसाय समझने लग गए हैं। धन कमाना ही उनके जीवन का उद्देश्य रह गया है। बड़े-बड़े पूँजीपति पब्लिक स्कूलों में इसलिए धन लगा रहे हैं ताकि रुपया कमा सकें। वे यह भूल गए हैं कि अध्यापन पावन एवं महान कार्य है। शिक्षक ही राष्ट्र की भावी उन्नति का जीवन बनाते, सुधारते और सँवारते हैं। इस संसार से अज्ञान का अंधकार दूर करके ज्ञान का दीपक जलाते हैं। छात्र-छात्राओं को नाना प्रकार की विद्याएँ देकर उन्हें विद्वान एवं योग्य नागरिक बनाते हैं। शिक्षक ही निःस्वार्थ भाव से राष्ट्र की सेवा कर सकते हैं। थोड़े धन से संतुष्ट रहकर वे आने वाली पीढ़ी को तैयार करते हैं और उनमें उत्तम गुणों का विकास करते हैं। आज हमारे देश में जो भ्रष्टाचार एवं मूल्यों की गिरावट आ चुकी है, उसके लिए बहुत कुछ हमारे शिक्षक तथा शिक्षा-व्यवस्था दोषी है।
4. आदर्श अध्यापक सच्चा सेवक:
प्राचीन काल में हमारे गुरुकुलों में सच्चे अर्थों में शिक्षा दी जाती थी। प्रत्येक विद्यार्थी के लिए गुरुकुल में रहना अनिवार्य होता था। शिक्षक भी गुरुकुल में ही रहता था। उसका समूचा जीवन अपने विद्यार्थियों के लिए होता था। यही कारण था कि बड़े-बड़े राजा भी अपने पुत्रों को वहाँ पर शिक्षा ग्रहण करने के लिए भेजते थे। शिक्षक अपने जीवन के अनुभवों द्वारा विद्यार्थियों को ज्ञान देता था। आदर्श शिक्षक समाज का सच्चा सेवक होता है। उसके सामने राष्ट्र-सेवा का आदर्श होता है। मैं ऐसा ही शिक्षक बनकर विद्यार्थियों को स्वावलंबन, सेवा, सादगी, स्वाभिमान, अनुशासन और प्रामाणिकता का पाठ पढ़ाऊँगा।
5. विद्यार्थियों की शिक्षा में रुचि उत्पन्न करना शिक्षक का कर्तव्य:
आज का विद्यार्थी विद्या को बोझ समझकर उससे दूर भागता है। परिणामस्वरूप, ग्रामीण क्षेत्र के स्कूलों में विद्यार्थी स्कूल जाना बंद कर देते हैं और उनकी शिक्षा अधूरी रह जाती है। मैं विद्यार्थियों में शिक्षा के प्रति रुचि उत्पन्न करूँगा और यह प्रयास करूँगा कि जो विद्यार्थी स्कूल में आ गया है, वह स्कूल छोड़कर न जाए और अपनी शिक्षा को पूरा करे। छात्रों में अकसर अनुशासनहीनता देखी जा सकती है। उधर कुछ विद्यार्थी राजनीति में भाग लेकर अपनी शिक्षा को बीच में ही छोड़ देते हैं। इसके लिए अध्यापक ही दोषी हैं। यदि अध्यापक सही ढंग से पढ़ाएँ तो विद्यार्थी का मन अन्यत्र कहीं नहीं लगेगा। मैं इस बात का प्रयत्न करूँगा कि विद्यार्थी अपना मन पढ़ाई में लगाएँ और एक अनुशासनबद्ध व्यक्ति की तरह जीवनयापन करें।
उपसंहार:
मैं अपनी कक्षा को परिवार के समान समशृंगा। अनुशासन का पूरा ध्यान रखूगा। पढ़ाई में कमज़ोर छात्रों का विशेष ध्यान रखूगा। उनको अतिरिक्त समय देकर भी पढ़ाऊँगा। मैं मनोविज्ञान के आधार पर अध्यापन करूँगा ताकि विद्यार्थियों की कमज़ोरी का सही कारण जान सकूँ। होनहार छात्रों को भी मैं विशेष रूप से प्रोत्साहित करूँगा ताकि वे आगे चलकर जीवन में विशेष उपलब्धि प्राप्त कर सकें। शिक्षा और खेलकूद का उचित समन्वय स्थापित करूँगा। अभिनय, वाद-विवाद, सामान्य ज्ञान तथा निबंध प्रतियोगिता में भाग लेने की प्रेरणा अपने विद्यार्थियों को दूंगा। मैं स्वयं सादा.रहूँगा और अपनी सादगी, सरलता, विनम्रता तथा सहृदयता से विद्यार्थियों को भी प्रभावित करूँगा। सक्षेप में, मैं एक आदर्श अध्यापक बनने का प्रयास करूँगा क्योंकि एक आदर्श एवं चरित्रवान अध्यापक ही विद्यार्थियों को सही दिशा दे सकता है। ईश्वर करे मेरा उद्देश्य पूर्ण हो और मैं अपने देश की सेवा करूँ।
29. वर्तमान शिक्षा प्रणाली
भूमिका:
भारत की वर्तमान शिक्षा-प्रणाली परतंत्रता काल की शिक्षा प्रणाली है। यह ब्रिटिश शासन की देन है। इस प्रणाली के जन्मदाता लॉर्ड मैकाले थे। उन्होंने कहा था-“मुझे पूरा विश्वास है कि इस शिक्षा-प्रणाली से भारत में एक ऐसा शिक्षित वर्ग पैदा होगा जो रक्त और रंग से ही भारतीय होगा परंतु रुचि, विचार, वाणी और मन-मस्तिष्क से अंग्रेज़।” यह शिक्षा-प्रणाली आज भी सफेद कालरों वाले बाबू और लिपिक ही पैदा कर रही है। इस शिक्षा-प्रणाली के कारण विद्यार्थियों की मानसिक, शारीरिक और आत्मिक शक्तियों का पूर्ण विकास नहीं हो पाता।
1. प्राचीन भारत में शिक्षा का महत्त्व:
भारत में प्राचीनकाल से ही शिक्षा का महत्त्व रहा है, बल्कि हम यह कह सकते हैं कि सभ्यता, संस्कृति तथा शिक्षा का उदय सबसे पहले भारत में ही हुआ। प्राचीन काल में शिक्षा के केंद्र गुरुकुल, नगरों के कोलाहल और शोरगुल से दूर वन-प्रदेशों में होते थे। महान ऋषि-मुनि इन गुरुकुलों का संचालन करते थे। प्रायः विद्यार्थी ब्रह्मचर्य का पालन करते हुए विज्ञान, चिकित्सा, नीति, युद्ध-कला तथा धर्म-शास्त्रों की शिक्षा गुरु चरणों में बैठकर प्राप्त करते थे। तक्षशिला, नालंदा, वाराणसी और मिथिला आदि में इसी प्रकार के विश्वविद्यालय थे। यहाँ विदेशी भी शिक्षा प्राप्त करने के लिए आते थे। फिर आया मध्य युग और भारत को लंबे काल तक परतंत्रता भोगनी पड़ी। मुसलमानों के शासनकाल में अरबी-फारसी की शिक्षा का प्रसार हुआ। 18-19वीं शताब्दी तक आते-आते शिक्षा केवल अमीरों और सामंतों तक सिमटकर रह गई। स्त्री शिक्षा तो लगभग समाप्त ही हो गई।
2. नवीन शिक्षा प्रणाली की आवश्यकता:
15 अगस्त, 1947 को भारत स्वतंत्र हुआ। हमारे कर्णधारों का ध्यान नई शिक्षा-प्रणाली की ओर गया क्योंकि ब्रिटिश शिक्षा-प्रणाली हमारी आवश्यकताओं के अनुकूल नहीं थी। राष्ट्रपिता महात्मा गांधी ने तो यहाँ तक कहा था, “शिक्षा से मेरा मतलब है, बच्चे या मनुष्य की तमाम शारीरिक, मानसिक और नैतिक शक्तियों का सर्वांगीण विकास।” अतः शिक्षा-प्रणाली में परिवर्तन लाने के लिए शिक्षा-शास्त्रियों ने अनेक समितियों का गठन किया। केंद्रीय विकास समिति की वयस्क शिक्षा कमेटी ने एक विशाल योजना बनाई जो तीन वर्ष के अंदर पचास प्रतिशत शिक्षा का प्रसार कर देना चाहती थी। सैकेंडरी शिक्षा की योजना का भी निर्माण हुआ। विश्वविद्यालयों के माध्यम से भी इस समस्या को सुलझाने के प्रयास चलते रहे। फिर सन् 1948 में ‘बेसिक शिक्षा समिति’ का गठन हुआ जिसका उद्देश्य पूरे भारत में बेसिक शिक्षा का व्यापक प्रसार करना था। अखिल भारतीय शिक्षा समिति की सिफारिश पर 6 वर्ष से 11 वर्ष की आयु वर्ग के सभी बच्चों के लिए बेसिक शिक्षा को अनिवार्य कर दिया गया।
3. कोठारी आयोग की स्थापना:
1964-66 के वर्षों में शिक्षा के क्षेत्र में परिवर्तन लाने के लिए सरकार ने कोठारी आयोग की स्थापना की। इस आयोग ने बड़ी सूझ-बूझ से राष्ट्रीय स्तर पर एक नई शिक्षा-प्रणाली-10 + 2 + 3 को लागू करने की सिफारिश की। वस्तुतः यह 15 वर्षीय नया पाठ्यक्रम है। लंबे काल तक इस नई पद्धति पर चर्चा-परिचर्चा चलती रही। अंततः देश के विभिन्न राज्यों में इस प्रणाली को लागू किया गया। इस प्रणाली में 10 वर्ष तक दसवीं कक्षा तक हाई स्कूलों में सामान्य शिक्षा होगी। इसमें सभी छात्र-छात्राएँ एक जैसे विषय पढ़ेंगे। इस पाठ्यक्रम में दो भाषाएँ, गणित, विज्ञान एवं सामाजिक अध्ययन पाँच विषय रखे गए हैं। इसके अतिरिक्त, किसी एक क्राफ्ट तथा शारीरिक शिक्षा का भी ज्ञान प्राप्त करना प्रत्येक छात्र के लिए अनिवार्य होगा। 10 वर्ष की हाई स्कूली शिक्षा के पश्चात विद्यार्थी अलग-अलग विषयों का अध्ययन करेगा। वह चाहे तो विज्ञान (मेडिकल या नॉन मेडिकल) ले सकता है, वह चाहे तो कॉमर्स और औद्योगिक कार्यों से संबंधित क्राफ्ट भी ले सकता है। उद्योग से संबंधित पाठ्यक्रम में विद्यार्थी चालीस से भी अधिक क्राफ्टों में रुचि के अनुसार प्रशिक्षण ले सकता है। ऐसा प्रशिक्षण प्राप्त विद्यार्थी देश के असंख्य लघु उद्योगों में काम कर सकता है।
4. नवीन शिक्षा नीति के लाभ:
वस्तुतः नवीन शिक्षा-प्रणाली रोज़गार को सामने रखकर बनाई गई है। प्रायः देखने में आता है कि अयोग्य विद्यार्थी महाविद्यालयों और विश्वविद्यालयों में प्रवेश ले लेते हैं लेकिन पढ़ने में उनकी रुचि नहीं होती। वे शिक्षण संस्थानों में अनुशासनहीनता और अराजकता उत्पन्न करने लगते हैं। नई शिक्षा नीति का सबसे बड़ा लाभ यह है कि ऐसे विद्यार्थी 10 +2 तक ही रह जाएंगे और उनको महाविद्यालयों में प्रवेश ही नहीं मिलेगा। केवल योग्य विद्यार्थी ही कॉलेजों में प्रवेश ले सकेंगे। 10 + 2 करने के पश्चात अधिकांश विद्यार्थी विभिन्न डिप्लोमा पाठ्यक्रमों में प्रवेश लेकर रोज़गार प्राप्त कर सकेंगे लेकिन इस नवीन शिक्षा-पद्धति को सफल बनाने के लिए यह भी आवश्यक है कि स्थान-स्थान पर डिप्लोमा पाठ्यक्रम भी आरंभ किए जाएँ ताकि 10 + 2 करने के पश्चात विद्यार्थी कॉलेजों की ओर न भागें।
उपसंहार:
इससे शिक्षित बेरोज़गारों में कमी आएगी और शिक्षित लोगों का समाज में मान-सम्मान होगा। यह नवीन शिक्षा प्रणाली विद्यार्थियों के सर्वांगीण विकास में सहायक होगी और भविष्य के निर्माण के लिए उपयुक्त होगी। इस प्रणाली को सफल बनाने का भार हमारे शिक्षकों पर भी है। सरकार इस ओर भी ध्यान रखे कि केवल योग्य विद्यार्थी ही आगे चलकर शिक्षक बनें क्योंकि योग्य शिक्षक ही उत्तम शिक्षा दे सकते हैं। नई शिक्षा नीति में इसी बात पर बल दिया गया है कि सुयोग्य शिक्षक ही शिक्षा जगत में प्रवेश करें। साथ ही, इस बात का भी ध्यान रखा गया है कि इस शिक्षा नीति के फलस्वरूप विद्यार्थियों को रोज़गार के अधिकाधिक अवसर मिल सकें।

30. पुस्तकालय का महत्त्व
भूमिका:
पुस्तकालय ‘पुस्तक + आलय’ के योग से बना है। इसका अर्थ है पुस्तकों का घर । यदि पुस्तकालय का अर्थ पुस्तकों का घर मानें तो प्रकाशकों का गोदाम, पुस्तकों की दुकान या पुस्तकों का निर्माण करने का स्थान भी पुस्तकालय कहा जा सकता है, परंतु ऐसा नहीं है। पुस्तकालय का सही अर्थ है-वह भवन, जहाँ अध्ययन के लिए पुस्तकें रखी गई हों या वह भवन, स्थान जहाँ से सर्वसाधारण को पढ़ने के लिए पुस्तकें मिलती हों। . पुस्तकालय ज्ञान का आगार होता है। यह ज्ञान-अर्जन के साथ-साथ मनोरंजन का स्वस्थ साधन है। इसे हम व्यक्ति की जिज्ञासा की शांति का स्थान तथा मानसिक विकास का साधन कह सकते हैं। पुस्तकालय शिक्षा, ज्ञान एवं विद्या का प्रचार-प्रसार करने का सबल साधन है। पुस्तकालय एक मंदिर की भाँति से होता है जहाँ उच्चकोटि के संत-महापुरुषों के अनुभवों को सुरक्षित रखा जाता है। एडीसन के अनुसार, “पुस्तकें महती प्रतिभाओं के द्वारा मानव-जाति के लिए छोड़ी गई पैतृक संपत्ति हैं, जो पीढ़ी-दर-पीढ़ी को सौंपी जाने के लिए हैं, मानों वे अभी अजन्मे व्यक्तियों के लिए दिए गए ज्ञान का उपहार हों।” अतः पुस्तकालय संचित ज्ञान को सरल ढंग से प्राप्त करने में सबकी सहायता करता है।
ज्ञान प्राप्त करने का साधन-पुस्तकालय ज्ञान प्राप्त करने में अत्यधिक सहायता करता है। ज्ञान-प्राप्ति के प्रमुख दो साधन हैं-(1) विद्यालय और (2) पुस्तकालय। विद्यालय में अध्यापकों के मुख से सुनकर विद्या प्राप्त की जाती है और पुस्तकालयों में अध्ययन और चिंतन के द्वारा ज्ञान प्राप्त किया जाता है। विद्यालय स्तर पर विद्यार्थी में एकाग्रता का अभाव संभव हो सकता है, परंतु पुस्तकालय में प्रत्येक व्यक्ति श्रद्धा एवं एकाग्रता से ही ज्ञान प्राप्त करता है। पुस्तकालयों को दो भागों में बाँटा जा सकता है(1) वाचनालय तथा (2) पुस्तकालय।
वाचनालयों में विभिन्न प्रदेशों से प्रकाशित दैनिक, साप्ताहिक, पाक्षिक व मासिक पत्र-पत्रिकाओं का अध्ययन किया जाता है। पुस्तकालय में विभिन्न विषयों की पुस्तकों का भंडार होता है। प्राचीन, दुर्लभ पुस्तकों को पढ़ने का अवसर प्राप्त होता है।
पुस्तकालय के प्रकार-पुस्तकालय चार प्रकार के होते हैं-
1. निजी पुस्तकालय: इसमें व्यक्ति अपनी पसंद की अथवा विशेष प्रकार की पुस्तकें रखता है। इसका उपयोग सीमित होता है।
2. विद्यालय, महाविद्यालय तथा विश्वविद्यालय स्तर का पुस्तकालय: इस पुस्तकालय का प्रयोग उसके विद्यार्थी एवं अध्यापक करते हैं। विश्वविद्यालय स्तर के पुस्तकालय में अनेक प्रकाशित व अप्रकाशित शोध होते हैं। नवीन ज्ञान का विशाल भंडार भी इसे कह सकते हैं। यहाँ विभिन्न विषयों की पुस्तकों का प्रबंध रहता है।
3. संस्थागत पुस्तकालय: बड़ी कंपनियाँ, उद्योग, बैंक, क्लब, सरकारी कार्यालय अपनी-अपनी आवश्यकता के अनुसार अनेक प्रकार की पुस्तकों को इस पुस्तकालय में रखते हैं।
4. सार्वजनिक पुस्तकालय: इन पुस्तकालयों में जाकर कोई भी व्यक्ति ज्ञान का विस्तार और मनोरंजन कर सकता है।
हमारे देश में पुस्तकालयों की परंपरा बहुत पुरानी है। नालंदा, तक्षशिला और वल्लभी पुस्तकालय विश्व में प्रसिद्ध थे। स्वतंत्र भारत में पुस्तकालयों का पुनः विकास आरंभ हुआ।
उपसंहार:
पुस्तकालय विद्यार्थी और अध्यापक दोनों के लिए उपयोगी हैं। पुस्तकालय में सभी विषयों पर अलग-अलग पुस्तकें उपलब्ध होती हैं। अतः बिना धन खर्च किए विद्यार्थी और अध्यापक विषय की जानकारी प्राप्त कर सकते हैं। पुस्तकालय का वातावरण विद्यार्थी को पढ़ने में भरपूर योगदान देता है। पुस्तकालय में ज्ञान के साथ-साथ मनोरंजन की पुस्तकें भी होती हैं। पुस्तकों का अध्ययन करने से समय का सदुपयोग तो होता ही है, साथ-साथ ज्ञान में वृद्धि भी होती है। पुस्तकालय हमें अज्ञान के अंधेरे से निकालता है। हमारे देश में पुस्तकालयों का पूर्ण विकास नहीं हुआ है। अतः सरकार को पुस्तकालयों की उपयोगिता को समझकर इस ओर ध्यान देना चाहिए।
31. विद्यार्थी और फैशन
भूमिका:
शब्दकोश में फैशन का अर्थ है ढंग या शैली परंतु लोकव्यवहार में फैशन से अभिप्राय है-परिधान-शैली अर्थात् वस्त्र पहनने की कला। व्यक्ति अपने आपको आकर्षक दिखाने के लिए फैशन करता है। गोरा या काला, दुर्बल या मोटा, नवयुवक या प्रौढ़ सभी व्यक्ति अपने-अपने ढंग से वस्त्र पहनते हैं। व्यक्ति अपने शरीर की बनावट, रंग-रूप और आयु के अनुसार फैशन करता है। यहाँ तक फैशन के विषय में कोई विशेष विवाद नहीं है। विद्यार्थी हो या अध्यापक, लड़का हो या लड़की, पुरुष हो या स्त्री सभी को फैशन करने का जन्मसिद्ध अधिकार है।
1. फैशन पर विवाद:
फैशन के विषय पर विवाद तब उठता है, जब हम उसका गूढ़ अर्थ लेते हैं बनाव-सिंगार। फैशन के इस गूढ़ अर्थ से अर्थात् बनाव-सिंगार से दूल्हा-दुल्हन का तो संबंध हो सकता है किंतु छात्र और छात्राओं का फैशन से कोई संबंध नहीं होना चाहिए। छात्र और छात्राएँ अभी विद्यार्थी हैं और विद्यार्थी शब्द का अर्थ है विद्या चाहने वाले। यदि विद्या चाहने वाले विद्यार्थी विद्या के स्थान पर फैशन को चाहने लगेंगे तो वे अपने लक्ष्य से बहुत दूर हट जाएँगे। इस स्थिति में तो वही बात होगी, ‘आए थे हरिभजन को ओटन लगे कपास।’ इस प्रकार, फैशन में सीमा पार करने पर विद्यार्थी से विद्या रूठ जाएगी।
2. प्राचीनकाल में विद्यार्थियों में फैशन की भावना:
प्राचीन काल में विद्यार्थियों में बनावटी बनाव-सिंगार की इच्छा इतनी प्रबल नहीं थी जितनी आज के युग के विद्यार्थियों में विद्यमान है। प्राचीन काल में विद्यार्थी ‘सादा जीवन उच्च विचार’ में विश्वास करते थे। उनमें फैशन की अपेक्षा विद्या को चाहने की तीव्र इच्छा थी। आज के छात्र-छात्राओं में फैशनेबल दिखने की इच्छा अधिक तीव्र है। अधिकांश छात्र गली-मुहल्ले में जिस प्रकार का फैशन देखते हैं, वैसे ही वस्त्रों की मांग वे अपने माता-पिता से करते हैं। ऐसा इसलिए होता है कि वे अपने रहन-सहन से स्वयं को औरों की तुलना में धनी दिखाना चाहते हैं जबकि वास्तव में वे नहीं होते। धनी दिखाने के साथ-साथ आज का विद्यार्थी अपने आपको दूसरों से अधिक सुंदर दिखाना चाहता है जो वास्तव में वह नहीं है। इस प्रकार, बनाव-सिंगार में वे अपना इतना समय व्यर्थ करते हैं कि दूसरे महत्त्वपूर्ण कार्यों के लिए उनके पास न तो समय ही रहता है, न रुचि, न धन और न ही सामर्थ्य । ऐसी स्थिति में कौन उन्हें बुद्धिमान कहेगा और कौन उन्हें समझाए कि यह धन का अपव्यय तो है ही, साथ ही, समय की बरबादी भी है।
3. सौंदर्य के लिए धन की आवश्यकता:
जब विद्यार्थियों में सुंदर दिखने की भावना प्रबल होती है तो उनमें विलासिता भी बढ़ती है। विलासमय जीवन में धन की अति आवश्यकता होती है। जब विद्यार्थियों को विलासी जीवन बिताने के लिए धन सरलता से नहीं मिलता तो वे झूठ और छल का सहारा लेते हैं। यहाँ तक की चोरी की नौबत आ जाती है। तत्पश्चात, जुआ आदि व्यसन भी उनसे दूर नहीं रहते। इस प्रकार, विद्यार्थी की मौलिकता समाप्त हो जाती है और वह कृत्रिमता के वातावरण में जीने लगता है। ऐसे में सिनेमा के अभिनेता उसके आदर्श बनकर रह जाते हैं तथा गुरुजनों एवं परिवार से उनका संबंध टूट जाता है। विद्यालय की अपेक्षा फिल्मों में उनकी रुचि अधिक हो जाती है तथा वे अपने मार्ग से भटक जाते हैं। फैशन के पीछे भागने वाले विद्यार्थी कभी भी अपने जीवन में सफल नहीं होते। अतः आगे चलकर उनको निश्चय ही पछताना पड़ता है।
4. सिनेमा का कुप्रभाव:
आज सिनेमा हमारे जीवन का महत्त्वपूर्ण अंग बन चुका है। विशेषकर, छात्र-छात्राएँ फिल्मों से अत्यधिक प्रभावित हो रहे हैं। अमीर परिवार तो फिल्मी ढंग के वस्त्र पहन सकते हैं और अपने बच्चों को भी फैशनेबल वस्त्र पहना सकते हैं लेकिन गरीब आदमी के लिए ऐसा कर पाना संभव नहीं है। फिर देखा-देखी विद्यार्थियों में फैशन की होड़ बढ़ती जा रही है। टी०वी० की संस्कृति ने हमारे जीवन के लिए अनेक समस्याएँ उत्पन्न कर दी हैं। फैशनपरस्ती इन्हीं फिल्मों की ही देन है।
5. फैशन के दुष्परिणाम:
आज विद्यार्थियों में फैशन की बढ़ती हुई प्रवृत्ति न केवल उनके माता-पिता के लिए भी बल्कि समाज के लिए भी घातक सिद्ध हो रही है। निम्न मध्यवर्गीय परिवार अपने बच्चों की मांगों की पूर्ति नहीं कर पाते। जिसके फलस्वरूप उनके बेटे-बेटियाँ घर में असहज वातावरण उत्पन्न कर देते हैं। फैशन के पीछे भागने वाले विद्यार्थी सिनेमा हालों में जाकर अशोभनीय व्यवहार करते हैं, गली-मोहल्लों में हल्ला मचाते हैं और हिंसात्मक गतिविधियों में भाग लेते हैं। फैशनपरस्ती विद्यार्थी जीवन के विकास में निश्चय से एक बाधा का काम करती है। जो विद्यार्थी केवल फैशनपरस्ती के पीछे भागते हैं, वे अपनी पढ़ाई की ओर ध्यान नहीं दे पाते। इस प्रकार ऐसे विद्यार्थी अपने माता-पिता की आशाओं पर पानी फेर देते हैं।
उपसंहार:
सार रूप में यह कहा जा सकता है कि फैशन विद्यार्थियों के लिए उचित नहीं है। विद्यार्थियों का आदर्श सादा जीवन एवं उच्च विचार ही होना चाहिए। फैशन के चक्कर में पड़कर विद्यार्थी को अपना जीवन नष्ट नहीं करना चाहिए। उसका लक्ष्य तो उचित शिक्षा प्राप्त करके अपने भावी जीवन का निर्माण ही होना चाहिए। जो विद्यार्थी फैशन की ओर ध्यान न देकर पढ़ाई की ओर ध्यान देते हैं, वे निश्चय से अपने जीवन में सफल होते हैं। केवल फैशन के पीछे भागने वाले अंततः पछताते रह जाते हैं।
32. व्यायाम के लाभ
भूमिका:
एक स्वस्थ व्यक्ति ही जीवन का पूर्ण आनंद ले सकता है। अगर आदमी का शरीर स्वस्थ नहीं है तो जीवन की सभी प्रकार की सुविधाएँ उसके लिए व्यर्थ हैं। कालिदास ने भी अपने महाकाव्य ‘कुमारसंभव’ में कहा है
“शरीरमाचं खलु धर्म साधनं” अर्थात् शरीर ही धर्म का मुख्य साधन है। स्वास्थ्य ही जीवन है और अस्वास्थ्य मृत्यु है। अस्वस्थ व्यक्ति का किसी भी कार्य में मन नहीं लगता। बढ़िया-से-बढ़िया भोजन भी उसे विष के समान लगता है। यही नहीं, उसमें किसी भी काम को करने की क्षमता भी नहीं होती। यद्यपि स्वास्थ्य के लिए पौष्टिक और सात्विक भोजन की आवश्यकता होती है तथापि स्वास्थ्य-रक्षा के लिए व्यायाम की सर्वाधिक आवश्यकता है। व्यायाम से बढ़कर और कोई अच्छी औषधि नहीं है।
1. व्यायाम की आवश्यकता:
मानव-शरीर पाँच तत्त्वों से बना है। यही पाँच तत्त्व मानव-शरीर के लिए आवश्यक हैं। जब इनमें से किसी तत्त्व की कमी होती है तो शरीर अस्वस्थ हो जाता है। अतः शरीर को स्वस्थ बनाए रखने के लिए व्यायाम नितांत आवश्यक है। अंग्रेज़ी की उक्ति भी है-‘A sound mind dwells in sound body’ अर्थात् स्वस्थ शरीर में स्वस्थ मस्तिष्क का निवास होता है। अगर व्यक्ति प्रतिदिन व्यायाम करता रहता है तो उसका शरीर निरोग रहता है। जो लोग व्यायाम नहीं करते, उनका पेट बढ़ जाता है अथवा गैस की समस्या उत्पन्न हो जाती है या रक्तचाप में विकार आ जाता है।
2. व्यायाम के लाभ:
इसमें कोई संदेह नहीं कि व्यायाम स्वास्थ्य-रक्षा का साधन है परंतु व्यायाम नियमित रूप से करना चाहिए। जो लोग कुछ देर व्यायाम करके पुनः त्याग देते हैं, उनको लाभ की अपेक्षा हानि ही होती है। सबसे बड़ी बात तो यह है कि व्यायाम आयु और शक्ति के सामर्थ्य के अनुसार ही करना चाहिए।
व्यायाम से शरीर बलिष्ठ और सुडौल होता है। शारीरिक शक्ति बढ़ती है। शरीर में चुस्ती और फुर्ती आती है। व्यायाम करने वाले व्यक्ति का उत्साह बढ़ता है। यही नहीं, वह उद्यमी भी होता है। प्रतिदिन व्यायाम करने से खूब भूख लगती है और पाचन-शक्ति भी बढ़ती है। शरीर में रक्त का संचरण सही होता है। शरीर की मांसपेशियाँ और हड्डियाँ भी मज़बूत बनती हैं। इसके विपरीत, जो लोग व्यायाम नहीं करते, उनका शरीर रोगी हो जाता है। वे अकसर डॉक्टरों और हकीमों के यहाँ चक्कर लगाते रहते हैं। ऐसे विद्यार्थियों का पढ़ाई में मन भी नहीं लगता। व्यायाम न करने वालों का शरीर दुबला-पतला रहता है।
3. व्यायाम के प्रकार:
व्यायाम के अनेक प्रकार हैं। कुछ लोग व्यायाम का अर्थ दंड-बैठक लगाना ही लेते हैं परंतु शारीरिक व्यायाम में वे सभी क्रियाएँ आ जाती हैं जिनसे शरीर के अंग पुष्ट होते हैं। प्रातःकाल में खुली हवा में दौड़ लगाना या सैर करना भी व्यायाम है। इसी प्रकार से कुछ लोग घोड़े पर सवार होकर खुली हवा में सपाटे भरना पसंद करते हैं। कुछ लोग नदी में तैरते हैं। कुछ लोगों का दावा है कि नदी में तैरना अच्छा व्यायाम है। इसी प्रकार से अखाड़े में कुश्ती करना या मुग्दर घुमाना भी व्यायाम है। आज बड़े-बड़े नगरों में व्यायाम करने के लिए जिम खुल गए हैं। यहाँ पर युवक-युवतियाँ विभिन्न प्रकार के शारीरिक अभ्यास करते हैं। ग्रामीण क्षेत्रों में भी तरह-तरह के खेल-कूद होते रहते हैं। इन सबका एक ही उद्देश्य है-शरीर को स्वस्थ रखना।
4. व्यायाम और खेल-कूद:
व्यायाम का सबसे अच्छा साधन खेल-कूद है। फुटबाल, वॉलीबाल, बास्केटबाल, खो-खो, कबड्डी, क्रिकेट, बैडमिंटन, जिमनास्टिक आदि असंख्य ऐसे खेल हैं जो व्यायाम के सर्वश्रेष्ठ साधन हैं। जो व्यक्ति नियमित रूप में किसी-न-किसी खेल में भाग लेता है, उसका शरीर भी स्वस्थ रहता है। इसीलिए तो स्कूलों और कॉलेजों में खेल-कूद की ओर अधिक ध्यान दिया जाता है।
5. व्यायाम और योगाभ्यास:
व्यायाम का एक अन्य अच्छा और सस्ता साधन है-योगाभ्यास। इसमें कोई अधिक खर्च नहीं आता। घर में किसी स्थान पर पाँच-सात आसन अगर नियमित रूप से किए जाएँ तो शरीर काफी स्वस्थ रहता है। उदाहरण के रूप में, प्राणायाम, पद्मासन, सर्वांगासन, गोमुखासन, शवासन, सूर्य नमस्कार आदि कुछ ऐसे आसन हैं जिनके द्वारा शरीर को स्वस्थ रखा जा सकता है परंतु इन आसनों को किसी शिक्षक से सीखने के बाद ही करना चाहिए, तभी लाभ होगा, नहीं तो हानि भी हो सकती है। व्यायाम का सर्वाधिक अनुकूल समय प्रातःकाल है। इस समय वायु शुद्ध और स्वच्छ होती है। व्यायाम करने के बाद पौष्टिक और सात्विक भोजन करना चाहिए। बूढ़ों और रोगियों के लिए प्रातः और सायं का भ्रमण ही उचित है।
उपसंहार:
स्वास्थ्य ही मनुष्य का सच्चा धन है। अतः उसे बनाए रखने के लिए प्रतिदिन व्यायाम करना आवश्यक है परंतु अधिक व्यायाम करने से लाभ की अपेक्षा हानि होती है। अतः उचित मात्रा में ही व्यायाम करना चाहिए लेकिन इतना निश्चित है कि व्यायाम मानव के मस्तिष्क एवं शरीर के लिए अत्यधिक लाभकारी है।

33. भ्रष्टाचार-कारण व निवारण
अथवा
भ्रष्टाचार के बढ़ते चरण
भूमिका:
किसी भी देश के विकास के मार्ग में उस देश की विभिन्न समस्याएँ बहुत बड़ी बाधा हैं। इन समस्याओं में प्रमुख समस्या है-भ्रष्टाचार की समस्या। जिस समाज व राष्ट्र को भ्रष्टाचार का कीड़ा ग्रसने लगता है, उसका भविष्य फिर निश्चित रूप से अंधकारमय बन जाता है। भारतवर्ष का यह दुर्भाग्य है कि उस पर यह समस्या पूर्ण रूप से छाई हुई है। यदि समय रहते इसका समाधान न ढूँढा गया तो इसके भयंकर परिणाम सामने आएँगे।
1. भ्रष्टाचार का अर्थ:
भ्रष्टाचार का शाब्दिक अर्थ है-आचार से भ्रष्ट या अलग होना। अतः कहा जा सकता है कि समाज स्वीकृत आचार-सहिंता की अवहेलना करके दूसरों को कष्ट पहुँचाकर निजी स्वार्थों अथवा इच्छाओं की पूर्ति करना ही भ्रष्टाचार है। दूसरे शब्दों में, भ्रष्टाचार वह निंदनीय आचरण है, जिसके वशीभूत होकर मनुष्य अपने कर्तव्य को भूलकर अनुचित रूप से लाभ प्राप्त करने का प्रयास करता है। भाई-भतीजावाद, बेरोज़गारी, गरीबी आदि इसके दुष्परिणाम हैं जो हमारे राष्ट्र को भोगने पड़ रहे हैं।
2. भ्रष्टाचार के मूल कारण:
प्रत्येक विकराल समस्या के पीछे कोई-न-कोई कारण अवश्य रहता है। इसी प्रकार, भ्रष्टाचार के विकास के पीछे भी विभिन्न कारण हैं। वस्तुतः सुख एवं ऐश्वर्य के पनपने के साथ-साथ भ्रष्टाचार का जन्म हुआ। भ्रष्टाचार के मूल में मनुष्य का कुंठित अहंभाव, स्वार्थपरता, भौतिकता के प्रति आकर्षण, अर्थ एवं काम की प्राप्ति की लिप्सा छिपी हुई है। मनुष्य की अंतहीन इच्छाएँ भी भ्रष्टाचार का प्रमुख कारण है। इसके अतिरिक्त, अपनों का पक्ष लेना भ्रष्टाचार का सबसे बड़ा कारण है। कवि हर्ष ने अपनी रचना ‘नैषधचरितं’ में लिखा है कि अपने प्रियजनों के लिए पक्षपात होता ही है। यही पक्षपात भ्रष्टाचार का मूल कारण है।
3. भ्रष्टाचार की व्यापकता एवं प्रभाव:
भ्रष्टाचार का प्रभाव एवं क्षेत्र अत्यंत व्यापक है। आज ऐसा कोई भी क्षेत्र नहीं है, जहाँ भ्रष्टाचार ने अपनी जड़ें न फैला रखी हों। व्यक्ति, समाज, राष्ट्र यहाँ तक कि अंतर्राष्ट्रीय जगत इसकी गिरफ्त में आए हुए हैं। यह घट-घट, कण-कण में राम की भाँति समाया हुआ है। ऐसा लगता है कि संसार का कोई कार्य इसके बिना पूरा नहीं होता। राजनीतिक क्षेत्र तो भ्रष्टाचार का घर ही बन चुका है। आज विधायक हो या सांसद बाज़ार में रखी वस्तुओं की तरह बिक रहे हैं। भ्रष्टाचार के बल पर सरकारें बनाई और गिराई जाती हैं।
धार्मिक स्थलों पर जाकर देखा जा सकता है कि धर्मात्मा कहलाने वाले लोग ही भ्रष्टाचार की गंगा में डुबकियाँ लगा रहे हैं। आर्थिक क्षेत्र में तो भ्रष्टाचार ने कमाल ही कर दिखाया है। करोड़ों की रिश्वत लेने वाला व्यक्ति सीना तानकर चलता है। अरबों रुपयों का घोटाला करने वाला नेता जनता में सम्माननीय बना रहता है। चुरहट कांड, बोफोर्स कांड, मंदिर कांड, मंडल कांड, चारा कांड आदि सब भ्रष्टाचार के ही भाई-बंधु हैं। शिक्षा के क्षेत्र में भी भ्रष्टाचार अपने जौहर दिखा रहा है। योग्य छात्र उच्च कक्षाओं में प्रवेश नहीं पा सकते किंतु अयोग्य छात्र भ्रष्टाचार का सहारा लेकर कहीं-से-कहीं जा पहुँचते हैं और योग्य विद्यार्थी हाथ मलता रह जाता है।
4. भ्रष्टाचार के निवारण के उपाय:
भ्रष्टाचार को रोकना अत्यंत कठिन कार्य है क्योंकि जो-जो कदम इसे रोकने के लिए उठाए जाते हैं, वे कुछ दूर चलकर डगमगाने लगते हैं और भ्रष्टाचार की लपेट में आ जाते हैं। यह संक्रामक रोग की भाँति है। यह व्यक्ति से आरंभ होकर पूर्ण समाज को ग्रस लेता है। भ्रष्टाचार को रोकने के लिए समाज में पुनः नैतिक मूल्यों की स्थापना करनी होगी। जब तक हमारे जीवन में नैतिकता का समावेश नहीं होगा, तब तक हमारा भौतिकवादी दृष्टिकोण नहीं बदल सकता। नैतिकता के अभाव में सारी बातें कोरी कल्पना ही बनकर रह जाएँगी। इसलिए हमें प्रत्येक व्यक्ति के हृदय में नैतिकता के प्रति श्रद्धा एवं सम्मान उत्पन्न करना होगा।
समाज में फैले भ्रष्टाचार को रोकने के लिए ईमानदारी से काम करने वाले लोगों को प्रोत्साहन देना चाहिए ताकि उन्हें देखकर दूसरे उनका अनुकरण करने लगे और ईमानदारी के प्रति लोगों के मन में आस्था उत्पन्न हो। भ्रष्टाचार को रोकने के लिए कठोर दंड की व्यवस्था की जानी चाहिए। आज दंड व्यवस्था इस तरह कमज़ोर है कि यदि कोई व्यक्ति रिश्वत लेता हुआ पकड़ा जाए तो रिश्वत देकर बच निकलता है। ऐसी स्थिति में भ्रष्टाचार को बढ़ावा मिलता है।
उपसंहार:
भ्रष्टाचार को समाप्त करना केवल सरकार का ही कर्तव्य नहीं है। यह प्रत्येक व्यक्ति, समाज और संस्था का भी कर्तव्य है। आज हम सबको मिलकर भ्रष्टाचार को समाप्त करने का प्रयास करना होगा। प्रत्येक व्यक्ति को केवल अपने स्वार्थो तक ही सीमित नहीं रहना चाहिए अपितु ‘बहुजन हिताय बहुजन सुखाय’ के सिद्धांत को अपनाकर चलना चाहिए, तभी हम भ्रष्टाचार को समाप्त कर सकेंगे। यह बात दो टूक कही जा सकती है कि भ्रष्टाचार के दानव को नष्ट किए बिना हमारा राष्ट्र उन्नति नहीं कर सकता। इसका सबसे बड़ा दुष्परिणाम यह है कि आज भ्रष्टाचार के कारण अमीर और अमीर होता जा रहा है और गरीब और गरीब होता जा रहा है। यह आर्थिक विषय कभी भी देश की स्वतंत्रता के लिए खतरा बन सकती है।
34. दहेज-प्रथा-एक अभिशाप
भूमिका:
भारतीय समाज में अनेक कुप्रथाएँ फैली हुई हैं। दहेज प्रथा, सती प्रथा, बाल-विवाह जैसी कुप्रथाओं ने आज संसार के उन्नत देशों के सामने भारत का सिर झुका दिया है। दहेज प्रथा भारतीय समाज की एक प्रमुख कुरीति है। वैसे तो विवाह के अवसर पर दहेज लेने तथा देने की प्रथा हमारे समाज में सदियों से चली आ रही है परंतु इसका जो आदर्श रूप हमारे प्राचीन समाज में प्रचलित था, वह न जाने कब और कहाँ लुप्त हो गया। प्राचीनकाल में दहेज माता-पिता के प्रेम का प्रतीक था, जिसे वे कन्या के विवाह के अवसर पर उपहार के रूप में देते थे परंतु अब यह प्रथा एक सामाजिक अभिशाप का रूप धारण कर चुकी है।
1. ‘दहेज’ शब्द का अर्थ:
‘दहेज’ शब्द अरबी के शब्द ‘जहेज’ से रूपांतरित होकर बना है, जिसका अर्थ है-‘सौगात’। यह प्रथा भारतीय समाज में कब शुरू हुई, यह कहना तो कठिन है। वेदों में भी इस प्रथा का परिचय मिलता है। दहेज प्रथा हमारे देश में प्राचीनकाल से चली आ रही है। वैदिक काल में माता-पिता कन्या के विवाह के पश्चात उसको अपनी गृहस्थी बसाने के लिए कुछ वस्तुएँ दिया करते थे; जैसे चूल्हा, कुछ बर्तन, चारपाई, गाय, चावल तथा कुछ घरेलू वस्तुएँ। यह माता-पिता की ओर से एक प्रकार का उपहार होता था परंतु वर पक्ष के लोग कन्या पक्ष के सामने अपनी कोई माँग नहीं रखते थे।
2. नारी का सम्मान:
प्राचीन भारतीय समाज में नारी का स्थान बहुत ऊँचा था। ‘यत्र नार्यस्तु पूज्यन्ते रमन्ते तत्र देवता’ अर्थात जहाँ नारी की पूजा की जाती है, वहाँ देवता निवास करते हैं। परिवार में स्त्री को बहुत सम्मान की दृष्टि से देखा जाता था। कोई भी धार्मिक कार्य स्त्री के बिना पूरा नहीं हो सकता था। उसे अपनी योग्यतानुसार वर चुनने का भी अधिकार प्राप्त था। शकुंतला, मैत्रेयी, सीता, अनुसूइया आदि इसके उदाहरण हैं परंतु देश के गुलाम होने के साथ-साथ स्त्री की स्वतंत्रता भी खत्म हो गई। उससे शिक्षा का अधिकार भी छीन लिया गया। वह केवल विलासिता की वस्तु बनकर रह गई।
3. प्रदर्शन की भावना:
स्वतंत्रता-प्राप्ति के बाद देश में दहेज-प्रथा को बढ़ावा मिला है। आज समाज में प्रदर्शन की भावना बढ़ती जा रही है। अमीर लोग बेटी के विवाह में बढ़-चढ़ कर दहेज देते हैं परंतु गरीब लोग ज़्यादा दहेज न दे सकने के कारण अपनी बेटी के लिए अच्छा वर नहीं खरीद पाते। आजकल वर पक्ष के लोग भी बढ़-चढ़कर दहेज माँगते हैं। काले धन के प्रसार से भी दहेज-प्रथा को बढ़ावा मिला है। यद्यपि आजकल स्त्रियाँ अपने पैरों पर खड़ी होकर पुरुषों के साथ कंधे-से-कंधा मिलाकर काम कर रही हैं तथापि आजकल माँ-बाप लड़की के जन्म पर दुःख मनाते हैं। सामर्थ्य से अधिक दहेज देने के बाद भी माता-पिता निश्चित नहीं हो पाते कि ससुराल में उनकी बेटी का स्वागत होगा या नहीं ?
4. एक सामाजिक बुराई:
दहेज-प्रथा भारतीय समाज के लिए कलंक है। यह एक ऐसी सामाजिक बुराई है जो भारतीय समाज को खोखला करती जा रही है। यह प्रथा परिवार की आर्थिक दशा को दीमक की तरह खाए जा रही है। प्रायः माता-पिता विवाह के समय दूसरों से ऋण लेकर अपनी शक्ति से अधिक दहेज देते हैं। फिर भी न जाने कितनी युवतियाँ दहेज की बलिवेदी पर चढ़ जाती हैं। दहेज रूपी दैत्य के आतंक के कारण न जाने कितनी युवतियाँ कुँवारी रह जाती हैं। अनमेल-विवाह, असफल प्रेम-विवाह तथा विवाह से पूर्व चारित्रिक पतन इसी प्रथा के दुष्परिणाम हैं।
5. दहेज:
प्रथा उन्मूलन विधेयक-दहेज-प्रथा को समाप्त करने के लिए सन 1961 में कानून बनाया गया। इसके अनुसार, वर पक्ष के लोग दो हज़ार रुपए से अधिक का दहेज नहीं ले सकते। इस कानून में दहेज लेने वालों के लिए दंड की व्यवस्था भी की गई थी। सन 1975 में इस कानून को और अधिक कठोर बनाया गया। सन 1976 में एक और कानून बनाया गया जिसके अनुसार किसी भी सरकारी कर्मचारी के दहेज लेने पर उसके विरुद्ध कार्रवाई की जाएगी परंतु जनता के सक्रिय सहयोग के अभाव में ये सब कानून केवल कानून की पुस्तकों तक ही सीमित रह गए।
6. युवा वर्ग में जागृति:
दहेज-प्रथा के उन्मूलन के लिए देश में युवा वर्ग की जागृति अति आवश्यक है। शिक्षित युवक-युवतियों को यह दृढ़ प्रतिज्ञा करनी चाहिए कि न हम दहेज लेंगे और न हम दहेज देंगे। दहेज-विरोधी कानून का सख्ती से पालन करवाया जाए तथा दहेज लेने वालों को कड़े-से-कड़ा दंड दिया जाए। माता-पिता को भी कन्या की शिक्षा पर अधिक ध्यान देना चाहिए ताकि वह आत्मनिर्भर हो सके। कन्या के आत्मनिर्भर होने पर उसे ससुराल में सम्मान की दृष्टि से देखा जाएगा। अंतर्जातीय विवाह को प्रोत्साहन दिया जाए, इससे योग्य वर मिलने में कठिनाई नहीं होगी।
उपसंहार:
माता-पिता की सुख-शांति पर वज्रपात करने वाला, बालिकाओं के सुख पर तुषारापात करने वाला, आए दिन वधू हत्याओं तथा आत्मदाहों को प्रोत्साहन देने वाला, घोड़े-बैलों की तरह क्रय-विक्रय का यह व्यवसाय निश्चय ही आज भारत के सामने एक राष्ट्रीय समस्या के रूप में मुँह खोले खड़ा है। यदि शिक्षित युवक-युवतियाँ रूढ़िवादी मनोवृत्ति वाले माता-पिता की अवहेलना करके सरकार को सहयोग दें तो भारतीय संस्कृति पर लगा हुआ यह कलंक निश्चय ही धुल जाएगा।

35. मेरा प्रिय कवि
अथवा
महाकवि तुलसीदास
भूमिका:
साहित्य समाज का दर्पण है और लेखक युगद्रष्टा होता है। वह अपने आस-पास की परिस्थितियों से प्रेरित होकर साहित्य की रचना करता है। महाकवि ही श्रेष्ठ साहित्य की रचना कर सकते हैं। श्रेष्ठ साहित्य के तीन उद्देश्य होते हैं-सत्यं, शिवं एवं सुंदरं। भक्ति काल के महाकवि गोस्वामी तुलसीदास ऐसे ही युग प्रवर्तक एवं समन्वयवादी कवि थे जिन्होंने अपने साहित्य द्वारा समाज में आदर्शों की स्थापना की और हिंदू धर्म तथा जाति का उद्धार किया। मेरे प्रिय कवि महाकवि एवं रामभक्त तुलसीदास ही हैं। भारत में महात्मा बुद्ध के पश्चात यदि कोई दूसरा समन्वयकारी एवं युगद्रष्टा व्यक्ति हुआ है तो वह महाकवि तुलसीदास ही हैं। आज बड़े-बड़े राजाओं का साम्राज्य नष्ट हो चुका है लेकिन उस महाकवि का साम्राज्य आज भी विद्यमान है।
1. जन्म एवं काल:
तुलसीदास का जन्म संवत 1554 में श्रावण सप्तमी के दिन बांदा जिले के राजापुर ग्राम में हुआ। इनके पिता का नाम आत्माराम दूबे और माता का नाम हुलसी था। अभुक्त मूल नक्षत्र में उत्पन्न होने के कारण माता-पिता ने इनको त्याग दिया और पालन-पोषण के लिए एक दासी को दे दिया। तत्पश्चात, इनकी भेंट स्वामी नरहरिदास से हुई। वे इन्हें अयोध्या ले गए और उन्होंने इनका नाम ‘रामबोला’ रखा। बालक तुलसीदास दीक्षित होकर विद्याध्ययन करने लगे। काशी में 15 वर्षों तक रहकर इन्होंने वेदों आदि का समुचित अध्ययन किया। गोस्वामी की पत्नी का नाम रत्नावली था।
2. प्रमुख रचनाएँ:
गोस्वामी द्वारा रचित ग्रंथों की संख्या 37 मानी गई है लेकिन नागरी प्रचारिणी सभा, काशी द्वारा प्रकाशित ‘तुलसी ग्रंथावली’ के अनुसार इनके बारह ग्रंथ ही प्रामाणिक हैं। ये हैं-रामचरितमानस, विनय पत्रिका, दोहावली, गीतावली, कवितावली, रामाज्ञा प्रश्न, बरवै रामायण, रामलला नहछू, कृष्ण गीतावली, वैराग्य संदीपनी, पार्वती मंगल, जानकी मंगल। गोस्वामी जी की कीर्ति का आधार स्तंभ तो ‘रामचरितमानस’ ही है। इस महाकाव्य का आधार ‘वाल्मीकि रामायण’ है।
3. विषय की व्यापकता एवं प्रबंध योजना:
तुलसीदास ने व्यापक काव्य-विषय ग्रहण किए हैं। कृष्णभक्त कवियों के समान उन्होंने जीवन के किसी अंग विशेष का वर्णन न करके उसके समग्र रूप को ग्रहण किया। उनकी रचनाओं में शृंगार, शांत, वीर आदि सभी रसों का परिपाक है। उनके द्वारा रचित ‘रामचरितमानस’ में धर्म, संस्कृति तथा काव्य सभी का आश्चर्यजनक समन्वय है। उनके काव्य की सबसे बड़ी विशेषता प्रबंध योजना है। उनकी लगभग सभी रचनाओं में कथासूत्र पाए जाते हैं। ‘रामचरितमानस’ की प्रबंध-पटुता तो सर्वश्रेष्ठ है। चार-चार वक्ताओं द्वारा वर्णित होने पर भी उसमें कहीं पर भी अस्पष्टता नहीं आने पाई। समूची कथा मार्मिक प्रसंगों से भरी पड़ी है। कथानक, वस्तु-वर्णन, व्यापार-वर्णन, भाव प्रवणता और संवाद सभी में पूरा संतुलन है। राम-सीता का परस्पर प्रथम दर्शन, राम वन-गमन, दशरथ की मृत्यु, भरत का पश्चाताप, सीता हरण, लक्ष्मण मूर्छा आदि अनेक ऐसे मार्मिक स्थल हैं जिनके कारण ‘रामचरितमानस’ की प्रबंध योजना काफी प्रभावोत्पादक बन पड़ी है।
4. महान समन्वयवादी चिंतक:
गोस्वामी तुलसीदास एक महान समन्वयवादी चिंतक भी थे। उन्होंने दर्शनशास्त्र के शुष्क एवं नीरस सिद्धांतों को भावमय बना दिया। उनके काव्य की महान उपलब्धि उनकी समन्वय भावना है। उन्होंने समाज में व्याप्त विषमता को मिटाकर उसमें एकरूपता उत्पन्न करने का प्रयास किया। धर्म, समाज, राजनीति, साहित्य प्रत्येक क्षेत्र में उन्होंने समन्वयवादी प्रवृत्ति को अपनाया। डॉ० हज़ारीप्रसाद द्विवेदी ने उनके बारे में उचित ही कहा है-“तुलसी का सारा काव्य समन्वय की विराट् चेष्टा है। रामचरितमानस शुरू से आखिर तक समन्वय का काव्य है।”
5. सच्चे रामभक्त:
तुलसीदास राम काव्यधारा के प्रधान कवि हैं। वस्तुतः उनकी रचनाओं के कारण ही राम काव्यधारा श्रेष्ठ काव्यधारा मानी जाती है। वे कवि होने के साथ-साथ सच्चे रामभक्त भी थे। उन्होंने मर्यादा पुरुषोत्तम भगवान राम की भक्ति की स्थापना की। वे अपने आपको दीन और राम को दयालु, स्वयं को भिखारी और भगवान को दानी घोषित करते हैं। तुलसी की भक्ति के कारण ही आज ‘रामचरितमानस’ प्रत्येक हिंदू घर में उपलब्ध है और स्थान-स्थान पर रामायण का पाठ होता है।
6. कला-पक्ष:
तुलसी के काव्य का आंतरिक पक्ष जितना उज्ज्वल है, उसका बाह्य पक्ष भी उतना ही श्रेष्ठ है। भाषा पर उनका असाधारण अधिकार था। उन्होंने अपने समय में प्रचलित अवधी और ब्रज दोनों भाषाओं में काव्य रचनाएँ लिखीं। ‘रामचरितमानस’ में यदि अवधी का सुंदर प्रयोग है तो विनय पत्रिका में साहित्यिक ब्रज भाषा का। कहीं-कहीं अरबी और फारसी शब्दों का भी सुंदर मिश्रण है। इनकी भाषा सरल, सहज और प्रवाहमयी है। कुछ स्थानों पर प्रचलित लोकोक्तियों और मुहावरों का भी सुंदर प्रयोग है। पदों की दृष्टि से तुलसी का क्षेत्र व्यापक है। ‘रामचरितमानस’ में अगर दोहा-चौपाई का प्रयोग है तो ‘कवितावली’ में छंदों की भरमार है। कवित्त, सवैया, दोहा, गीति आदि छंदों का सुंदर प्रयोग है। तुलसी के काव्य में अलंकारों का प्रयोग भी पर्याप्त मात्रा में है परंतु यह अलंकार प्रयोग पूर्णतया स्वाभाविक रूप में हुआ है।

36. वर्षा ऋतु
देखो नभ में बादल छाए, दिन में हो गई रात।
आई है बरसात सुहानी आई है बरसात ॥
भूमिका:
भारतवर्ष ऋतुओं का देश है। इसमें छह ऋतुएँ प्रतिवर्ष समय पर आती हैं। प्रत्येक दो मास के बाद यहाँ नई ऋतु का आगमन होता है। गर्मी के बाद वर्षा शुरू हो जाती है। जब हम तेज़ गर्मी से झुलस जाते हैं तो वर्षा ऋतु आकर हमें राहत पहँचाती है। यह सावन और भादों के महीने में आती है। वर्षा ऋतु हमारे देश की सर्वाधिक महत्त्वपूर्ण ऋतु है। कारण यह है कि हमारा देश कृषि प्रधान देश है और कृषि का संबंध वर्षा से है। हमारे देश का विकास वर्षा पर ही निर्भर करता है। यदि वर्षा अच्छी होती है तो किसानों के खलिहान अनाज से भर जाते हैं और देश में समृद्धि व्याप्त हो जाती है।
1. ऋतुओं की रानी:
वसंत को यदि ‘ऋतुराज’ कहा जाता है तो वर्षा ‘ऋतुओं की रानी’ है। राजा और रानी का यह जोड़ा अत्यंत सुंदर एवं मनमोहक है। जेठ और आषाढ़ की लू से जब जन-जीवन तो क्या, वनस्पति भी झुलस रही होती है तो ऐसे ही समय पर आकर ऋतुओं की रानी हर प्रकार के जीव-जंतुओं तथा वनस्पतियों को राहत पहुँचाती है। वर्षा के आगमन से चारों ओर हरियाली छा जाती है तथा जन-जीवन प्रसन्नता से झूम उठता है। वर्षा को देखकर किसान का सीना चौड़ा हो जाता है और वह खेत जोतने की तैयारी करने लगता है।
2. किसानों के लिए वरदान:
भारत में मई और जून की कड़कती गर्मी के बाद जुलाई और अगस्त में वर्षा का आगमन होता है। वर्षा का स्वागत किसान नाच-गाकर करता है, स्त्रियाँ झूला झूलती हैं तथा बच्चे नंग-धडंग होकर वर्षा में गलियों में घूमते हैं। चारों ओर हरियाली छा जाती है। जल ग्रहण कर पौधे और वृक्ष प्रसन्नता से झूम उठते हैं। ताल-तलैया पानी से भर जाते हैं। जगह-जगह मेंढकों के तराने शुरू हो जाते हैं। नदी-नालों में बरसात का पानी भर जाने से नव-यौवन का संचार हो जाता है। समय पर यदि अच्छी वर्षा हो जाए तो देश में अन्न का संकट टल जाता है। किसान के लिए अच्छी वर्षा वरदान सिद्ध होती है।
3. वर्षा से उत्पन्न कष्ट:
वर्षा ऋतु जहाँ प्राणी मात्र के लिए वरदान सिद्ध होती है, वहाँ वर्षा के कारण मनुष्यों को कुछ हानियाँ अथवा कष्ट भी उठाने पड़ते हैं। कई बार वर्षा के कारण नदियों में बाढ़ आ जाती है। अधिक वर्षा के कारण आस-पास के गाँवों तथा नगरों में बाढ़ का पानी भर जाता है। जीवन अस्त-व्यस्त हो जाता है। गलियों में पानी भर जाता है। यातायात ठप्प हो जाता है। व्यापार में रुकावट आ जाती है। अधिक वर्षा के कारण फसलों को भी हानि पहुँचती है। चारों ओर पानी भर जाने के कारण मच्छर उत्पन्न हो जाते हैं और गंदगी के कारण अनेक रोग फैल जाते हैं।
4. वर्षा ऋतु में प्रकृति की शोभा:
वर्षा ऋतु में प्रकृति की शोभा देखने योग्य होती है। आकाश में छाए हुए बादल देखकर मोर नाच उठते हैं। बादलों की गर्जन और उनमें चमकती बिजली की प्राकृतिक छवि मन को मोह लेती है। वर्षा के कारण सब जगह पानी-ही-पानी दिखाई देता है। नदियाँ और नाले उमड़ने लगते हैं। वर्षा ऋतु में बादलों से भरे आकाश में सात रंगों का इंद्रधनुष देखकर चित्त प्रसन्न हो उठता है। पोखरों तथा तालाबों में मेंढक टर्राते हैं, मोर नाचते हैं और सभी पशु-पक्षी प्रसन्न दिखाई देते हैं। असंख्य कवियों ने वर्षा ऋतु का सुंदर वर्णन किया है। वर्षाकाल में काले-काले बादल उमड़कर जीव-जंतुओं को सुख प्रदान करते हैं। कविवर पंत ने एक स्थल पर लिखा है-
झम-झम-झम-झम मेघ बरसते हैं सावन के,
छम-छम-छम-छम गिरती बूंदें तरुओं से छन के।
चम-चम बिजली चमक रही रे उर में घन के,
थम-थम दिन के तम में सपने जगते मन के।
उपसंहार:
सार रूप में कहा जा सकता है कि वर्षा ऋतु का भारतवर्ष में अत्यंत महत्त्व है। भारतवर्ष में कृषि का सारा काम वर्षा पर निर्भर करता है। अच्छी वर्षा से किसान को अत्यंत लाभ होता है। देश के नागरिकों के लिए खाद्यान्न तथा अन्य फसलें अच्छी होती हैं तथा देश में समृद्धि होती है। दूसरी ओर गर्मी से छुटकारा मिलता है तथा चारों ओर हरियाली छा जाती है। हाँ, कभी-कभी अधिक वर्षा के कारण मनुष्य को अनेक हानियाँ भी उठानी पड़ती हैं, फिर भी वर्षा ऋतु का सर्वत्र स्वागत ही होता है। वर्षा ऋतु में अनेक त्योहार मनाए जाते हैं। तीज इस ऋतु का प्रसिद्ध त्योहार है। इस अवसर पर युवतियाँ एवं स्त्रियाँ गीत गाती हैं तथा झूले झूलती हैं। वर्षा ऋतु भारत की समृद्धि का प्रतीक है।
37. आदर्श विद्यार्थी
भूमिका:
विद्यार्थी काल मानव के भावी जीवन की आधारशिला है। यदि यह आधारशिला मज़बूत है तो उसका जीवन निरंतर विकास करेगा, नहीं तो आने वाले कल की बाधाओं के सामने वह टूट जाएगा। यदि छात्र ने विद्यार्थी जीवन में परिश्रम, अनुशासन, संयम और नियम का अच्छी प्रकार से पालन किया है तो उसका भावी जीवन सुखद होगा। सभ्य नागरिक के लिए जिन गुणों का होना ज़रूरी है, वे गुण तो बाल्यकाल में विद्यार्थी के रूप में ही ग्रहण किए जाते हैं। जिस विद्यार्थी ने मन लगाकर विद्या का अध्ययन किया है; माता-पिता और गुरुजनों की विनम्रतापूर्वक सेवा की है; वह अवश्य ही एक सफल नागरिक बनेगा।
1. विद्यार्थी शब्द का अर्थ:
‘विद्यार्थी’ संस्कृत भाषा का शब्द है। यह दो शब्दों के मेल से बना है-‘विद्या’ और ‘अर्थी’ । इसका अर्थ है-विद्या चाहने वाला। विद्यार्थी विद्या प्राप्त करने के लिए ही विद्यालय में जाता है और विद्या से प्रेम करता है। वस्तुतः ‘विद्यार्थी’ शब्द में ही आदर्श विद्यार्थी के सभी गुण निवास करते हैं। अतः जिसके जीवन का लक्ष्य विद्या-प्राप्ति है, जो विद्या का अनुरागी है और जो निरंतर विद्यार्जन में ही अपना समय व्यतीत करता है, वही आदर्श विद्यार्थी है।
2. प्राचीनकाल में विद्यार्थी जीवन:
हमारे ऋषि-मुनियों ने मानव-जीवन को चार आश्रमों में बाँटा है। वे चार आश्रम हैं-ब्रह्मचर्य, गृहस्थ, संन्यास और वानप्रस्थ । ब्रह्मचर्य आश्रम ही विद्यार्थी जीवन है। उस काल में विद्याध्ययन के लिए बालक घर-परिवार से दूर आश्रम में जाते थे। आश्रम में तपस्या, ब्रह्मचर्य, अनुशासन और गुरु-सेवा करते हुए वे विद्या प्राप्त करते थे। स्वयं भगवान कृष्ण सुदामा के साथ संदीपन ऋषि के आश्रम में विद्या प्राप्त करने गए। विद्यार्थी काल में जो लोग एकाग्र मन से विद्या का अध्ययन करते रहे हैं, वही आगे चलकर महान बने हैं। अर्जुन, अभिमन्यु, एकलव्य, श्रीकृष्ण आदि के अनेक उदाहरण हमारे सामने हैं।
3. आदर्श विद्यार्थी के गुण:
संसार में गुणों से ही मनुष्य की पूजा होती है। संस्कृत में कहा भी गया है-“गुणैः हि सर्वत्र पदं निधीयते” अर्थात् गुणों द्वारा ही मनुष्य सर्वत्र ऊँचा स्थान प्राप्त कर सकता है। विद्यार्जन में ही सारे गुण निवास करते हैं। विद्यार्थी का जीवन साधना की अवस्था है। इस काल में वह ऐसे गुणों को ग्रहण कर सकता है जो आने वाले काल में न केवल उसके लिए अपितु समूचे समाज के लिए उपयोगी हों। गुरुजनों से ज्ञान प्राप्त करने के लिए विनम्रता परम आवश्यक है। यदि विद्यार्थी उदंड और उपद्रवी अथवा कटुभाषी है तो वह कभी भी आदर्श विद्यार्थी नहीं बन सकता। विद्या का लक्ष्य विनय की प्राप्ति है-विद्या ददाति विनयं। एक अच्छे गुरु से विद्या प्राप्त करने के तीन उपाय हैं-नम्रता, जिज्ञासा और सेवा। अतः एक सच्चे विद्यार्थी को विनम्र होना चाहिए।
अनुशासन का भी विद्यार्थी जीवन में उतना ही महत्त्व है, जितना कि विनम्रता का। जो विद्यार्थी अनुशासनप्रिय हैं, वे ही मेधावी छात्र बनते हैं लेकिन जो विद्यार्थी अनुशासनहीन होते हैं, वे अपने देश, अपनी जाति और अपने माता-पिता का अहित करते हैं। अनुशासन का पालन करने से ही विद्यार्थी का सही अर्थों में बौद्धिक विकास होता है। .
4. सादा जीवन और उच्च विचार;
आदर्श विद्यार्थी का मूल मंत्र है-सादा जीवन और उच्च विचार। जो बालक विद्यार्थी काल में सादगीपूर्ण जीवन व्यतीत करते हैं, उनका मन विद्या में लगा रहता है लेकिन आजकल पश्चिमी सभ्यता के दुष्प्रभाव के कारण विद्यार्थी फैशनपरस्ती के शिकार बन रहे हैं। माता-पिता भी इस ओर ध्यान नहीं दे रहे हैं। सादगी के साथ ही उच्च विचार जुड़े हुए हैं। जिस विद्यार्थी का ध्यान बाहरी दिखावे की ओर रहेगा, वह अपने अंदर उच्च विचारों का विकास कैसे कर पाएगा ? इसके साथ-साथ विद्यार्थी को सदाचार और स्वावलंबन का भी पालन करना चाहिए।
5. संयम और परिश्रम:
एक आदर्श विद्यार्थी के लिए संयम और परिश्रम दोनों ही आवश्यक हैं। विद्यार्थी जीवन संयमित और नियमित होना चाहिए। जीवन में उचित रीति का पालन करने वाले विद्यार्थी कभी असफल नहीं होते। एक आदर्श विद्यार्थी को अपनी इंद्रियों और मन पर पूर्ण संयम रखना चाहिए। समय पर सोना, समय पर उठना, समय पर भोजन करना, ब्रह्मचर्य का पालन करना और निरंतर परिश्रम करना एक अच्छे विद्यार्थी के गुण हैं। संयम-नियम का पालन करने से ही विद्यार्थी का मन अध्ययन में लगता है। परिश्रम के बिना विद्या नहीं आती। संस्कृत में कहा भी गया है-
सुखार्थी वा त्यजेत् विद्याम, विद्यार्थी वा त्यजेत् सुखं ।
सुखार्थिनः कुतो विद्या, विद्यार्थिनः कुतः सुखं ॥
6. विद्यार्थी और खेल-कूद:
आज के विद्यार्थी के लिए खेल-कूद में भाग लेना भी अनिवार्य है। इससे शरीर और मन दोनों स्वस्थ रहते हैं। जो विद्यार्थी खेल-कूद में भाग न लेकर केवल किताबी कीड़े बने रहते हैं, वे शीघ्र ही रोगी बन जाते हैं। खेल-कूद में भाग लेने से विद्यार्थी संयम और परिश्रम के नियमों को भी सीखता है। आज के स्कूलों और कॉलेजों में खेलों के लिए समुचित व्यवस्था है। अतः विद्यार्थियों को विद्याध्ययन के साथ-साथ खेलों में भी भाग लेना चाहिए।
उपसंहार:
इस प्रकार से आज का विद्यार्थी यदि देश का सुयोग्य नागरिक बनना चाहता है, अपना विकास करना चाहता है, अपने माता-पिता के प्रति अपने कर्तव्य का उचित निर्वाह करना चाहता है तो उसे वे सब गुण अपनाने होंगे जो उसके विकास के लिए आवश्यक हैं।
38. कुसंगति के दुष्परिणाम
भूमिका:
कुसंगति का शाब्दिक अर्थ है-बुरी संगति। अच्छे व्यक्तियों की संगति से बुद्धि की जड़ता दूर होती है, वाणी तथा आचरण में सच्चाई आती है, पापों का नाश होता है और चित्त निर्मल होता है लेकिन कुसंगति मनुष्य में बुराइयों को उत्पन्न करती है। यह मनुष्य को कुमार्ग पर ले जाती है। जो कुछ भी सत्संगति के विपरीत है, वह कुसंगति सिखलाती है। एक कवि ने कहा भी है-
काजल की कोठरी में कैसे हु सयानो जाय,
एक लीक काजल की लागि हैं, पै लागि हैं।
यह कभी नहीं हो सकता कि परिस्थितियों का प्रभाव हम पर न पड़े। दुष्ट और दुराचारी व्यक्ति के साथ रहने से सज्जन व्यक्ति का चित्त भी दूषित हो जाता है।
1. कुसंगति के दुष्परिणाम:
कुसंगति का बड़ा प्रभाव पड़ता है। एक कहावत है-अच्छाई चले एक कोस, बुराई चले दस कोस। अच्छी बातें सीखने में समय लगता है। जो जैसे व्यक्तियों के साथ बैठेगा, वह वैसा ही बन जाएगा। बुरे लोगों के साथ उठने-बैठने से अच्छे लोग भी बुरे बन जाते हैं। यदि हमें किसी व्यक्ति के चरित्र का पता लगाना हो तो पहले हम उसके साथियों से बातचीत करते हैं। उनके आचरण और व्यवहार से ही उस व्यक्ति के चरित्र का सही ज्ञान हो जाता है।
कुसंगति की अनेक हानियाँ हैं । दोष और गुण सभी संसर्ग से उत्पन्न होते हैं। मनुष्य में जितना दुराचार, पापाचार, दुष्चरित्रता और दुर्व्यसन होते हैं, वे सभी कुसंगति के फलस्वरूप होते हैं। श्रेष्ठ विद्यार्थियों को कुसंगति के प्रभाव से बिगड़ते हुए देखा जा सकता है। जो विद्यार्थी कभी कक्षा में प्रथम आते थे, वही नीच लोगों की संगति पाकर बरबाद हो जाते हैं। कुसंगति के कारण बड़े-बड़े घराने नष्ट-भ्रष्ट हो जाते हैं। बुद्धिमान-से-बुद्धिमान व्यक्ति पर भी कुसंगति का प्रभाव पड़ता है।
कवि रहीम ने भी एक स्थल पर लिखा है
बसि कुसंग चाहत कुसल, यह रहीम जिय सोच ।
महिमा घटी समुद्र की, रावन बस्यौ पड़ोस ॥
2. सज्जन और दुर्जन का संग:
सज्जन और दुर्जन का संग हमेशा अनुचित है बल्कि यह विषमता को ही जन्म देता है। बुरा व्यक्ति तो बुराई छोड़ नहीं सकता, अच्छा व्यक्ति ज़रूर बुराई ग्रहण कर लेता है। अन्यत्र रहीम कवि लिखते हैं
कह रहीम कैसे निभै, बेर केर को संग।
वे डोलत रस आपने, उनके फाटत अंग ॥
अर्थात बेरी और केले की संगति कैसे निभ सकती है ? बेरी तो अपनी मस्ती में झूमती है लेकिन केले के पौधे के अंग कट जाते हैं। बेरी में काँटे होते हैं और केले के पौधे में कोमलता। अतः दुर्जन व्यक्ति का साथ सज्जन के लिए हानिकारक ही होता है।
3. कुसंगति से बचने के उपाय:
हम अपने जीवन को सफल बनाना चाहते हैं तो हमें दुर्जनों की संगति छोड़कर सत्संगति करनी होगी। सत्संगति का अर्थ है-श्रेष्ठ पुरुषों की संगति। मनुष्य जब अपने से अधिक बुद्धिमान, विद्वान और गुणवान लोगों के संपर्क में आता है तो उसमें अच्छे गुणों का उदय होता है। उसके दुर्गुण नष्ट हो जाते हैं। सत्संगति से मनुष्य की बुराइयाँ दूर होती हैं और मन पावन हो जाता है। कबीरदास ने भी लिखा है
कबीरा संगति साधु की, हरे और की व्याधि ।
संगति बुरी असाधु की, आठों पहर उपाधि ॥
4. सत्संगति के प्रकार:
सत्संगति दो प्रकार से हो सकती है। पहले तो आदमी श्रेष्ठ, सज्जन और गुणवान व्यक्तियों के साथ रहकर उनसे शिक्षा ग्रहण करे। दूसरे प्रकार का सत्संग हमें श्रेष्ठ पुस्तकों के अध्ययन से प्राप्त होता है। सत्संगति से मनुष्यों की ज्ञान-वृद्धि होती है। संस्कृत में भी कहा गया है- सत्संगति : कथय किं न करोति पुंसाम्। रहीम ने पुनः एक स्थान पर कहा है
जो रहीम उत्तम प्रकृति, का करि सकत कुसंग।
चंदन विष व्यापत नहीं, लिपटे रहत भुजंग ॥
अर्थात यदि आदमी उत्तम स्वभाव का हो तो कुसंगति उस पर प्रभाव नहीं डाल सकती। यद्यपि चंदन के पेड़ के चारों ओर साँप लिपटे रहते हैं तथापि उसमें विष व्याप्त नहीं होता। उपसंहार-महाकवि सूरदास ने भी कुसंगति से बचने की प्रेरणा देते हुए कहा है-
तज मन हरि-विमुखनि को संग।
जाकै संग कुबुधि उपजति है, परत भजन में भंग ॥
बुरा व्यक्ति विद्वान होकर भी उसी प्रकार दुःखदायी है, जिस प्रकार मणिधारी साँप। मनुष्य बुरी संगति से ही बुरी आदतें सीखता है। विद्यार्थियों को तो बुरी संगति से विशेष रूप से सावधान रहना चाहिए। सिगरेट-बीड़ी, शराब, जुआ आदि बुरी आदतें व्यक्ति कुसंगति से ही सीखता है। अस्तु, कुसंगति से बचने में ही मनुष्य की भलाई है।

39. फुटबॉल मैच
अथवा
मेरा प्रिय खेल
भूमिका:
शिक्षा में खेलों का विशेष महत्त्व है। विद्यार्थियों के लिए पढ़ाई तथा खेल बराबर की महत्ता रखते हैं। खेल शिक्षा का एक आवश्यक अंग है। खेल के बिना शिक्षा कभी पूर्ण नहीं हो सकती। खेलों से जहाँ एक ओर शारीरिक शक्ति की वृद्धि होती है, वहाँ दूसरी ओर मानसिक शक्ति का भी विकास होता है। इससे विद्यार्थियों में संकल्प की दृढ़ता, संगठन की क्षमता तथा अनुशासनप्रियता बढ़ती है। खेलों के प्रचार तथा खेलों के प्रति छात्रों को जागृत करने के लिए मैचों का आयोजन किया जाता है। प्रतिवर्ष विभिन्न स्कूलों के बीच प्रतियोगिताओं का आयोजन किया जाता है जिनमें फुटबॉल, कबड्डी, क्रिकेट आदि के मैच होते हैं। विद्यार्थी अपनी-अपनी रुचि के अनुसार इन खेलों में भाग लेते हैं। विजेता टीम को पारितोषिक के रूप में ट्रॉफी दी जाती है। अन्य अच्छे खिलाड़ियों को भी पुरस्कार दिए जाते हैं।
1. अंतर्विद्यालय खेल प्रतियोगिता का आयोजन:
पिछले सप्ताह अंतर्विद्यालय खेल प्रतियोगिता (इंटर-स्कूल टूर्नामेंट) के अंतर्गत हमारे स्कूल का मैच राजकीय उच्च विद्यालय, पानीपत के साथ हुआ। यह एक फुटबॉल मैच था। यह मैच हमारे स्कूल के मैदान में खेला जाना तय हुआ। मैच रविवार को 5 बजे होना था। हमारे स्कूल के सभी खिलाड़ी 4 बजे स्कूल में इकट्ठे हो गए। क्रीड़ाध्यक्ष ने 15-20 मिनट तक खेल के विषय में कुछ आवश्यक निर्देश दिए। इसके बाद सभी खिलाड़ी स्कूल के गणवेश में सुसज्जित होकर चल पड़े। उनमें उत्साह एवं उल्लास था। सभी खिलाड़ी खेलने के लिए पूर्ण रूप से तैयार थे। दर्शक भी इस मैच को देखने के लिए मैदान में इकट्ठे होने लगे थे।
साढ़े चार बजे ही दोनों पक्षों के खिलाड़ी मैदान में पहुँच गए। हमारे स्कूल के खिलाड़ियों ने सफेद रंग के तथा विपक्षी टीम के खिलाड़ियों ने नीले रंग के कपड़े पहने हुए थे। सभी खिलाड़ी चुस्त दिखाई दे रहे थे। मैदान के चारों ओर दर्शकों की भीड़ लगी हुई थी। अतिथियों के लिए एक ओर कुर्सियाँ लगाई गई थीं। सामने ही मेज़ों पर पुरस्कार सजाकर रखे गए थे। ठीक पौने पाँच बजे रेफरी ने सीटी बजाई। दोनों टीमों के कप्तान टॉस के लिए रेफरी के पास आ गए। टॉस हमारे स्कूल के पक्ष में रही। इसके पश्चात दोनों टीमों के खिलाड़ी यथास्थान खड़े हो गए।
2. मैच का आरंभ:
ठीक पाँच बजे रेफरी ने सीटी बजाई तथा मैच शुरू हो गया। खेल के मैदान के चारों ओर रंग-बिरंगी झंडियाँ लगाई गई थीं। दृश्य बहुत ही मनोहर था। पुरस्कार वितरण के लिए मुख्यमंत्री को आमंत्रित किया गया था। अन्य अतिथियों के साथ वे भी मैच का आनंद ले रहे थे। मैच पूरे उत्साह एवं जोश के साथ प्रारंभ हो गया। दोनों ही टीमें बराबर दिख रही थीं। पहले आधे घंटे तक किसी भी ओर से गोल नहीं हआ। कभी गेंद इस ओर तो कभी उस ओर चली जाती थी। एक बार तो गेंद विपक्षियों के गोल में घुसते-घुसते रह गई। कुछ लोग विपक्षियों को प्रोत्साहित करने के लिए हूटिंग कर रहे थे। चारों ओर ‘वेल प्लेड’ की आवाजें आ रही थीं। हमारा गोलकीपर बड़ा ही फुर्तीला था, इसलिए विपक्षियों को गोल करने में कठिनाई अनुभव हो रही थी।
खेल धीरे-धीरे अधिक रोमांचकारी होता जा रहा था। हमारी टीम ने विपक्षी टीम को दबाना शुरू कर दिया था और मौका पाकर हमारी टीम ने गेंद को ऐसा फेंका कि गेंद विपक्षी गोलकीपर के पैरों के बीच से निकलती हुई गोल में जा पहुँची। उसी समय रेफरी ने सीटी बजा दी। हमारे विद्यालय के छात्रों तथा दर्शकों ने तालियाँ बजाईं परंतु विपक्षी टीम हतोत्साहित नहीं हुई। विपक्षी टीम के समर्थकों ने खिलाड़ियों के नाम लेकर उन्हें प्रोत्साहित करना शुरू कर दिया। दोनों टीमें जी-जान से खेल रही थीं। कुछ समय पश्चात खेल का आधा समय हो गया। रेफरी की सीटी के साथ मैच को रोक दिया गया। खिलाड़ी विश्राम के लिए चल पड़े। सभी खिलाड़ियों को जलपान करवाया गया तथा खाने के लिए संतरे दिए गए।
3. पानीपत टीम की पराजय:
दस मिनट के पश्चात फिर से सीटी बजी तथा सभी खिलाड़ी अपने-अपने स्थान पर पहुंच गए। खेल फिर से शुरू हुआ। विपक्षी टीम पूरी शक्ति लगाकर खेल रही थी परंतु गोल कर पाने में असमर्थ रही। कभी गेंद इधर आ जाती, कभी उधर चली जाती। उतने में ही हमारी टीम के एक खिलाड़ी ने गेंद को ऐसी हिट लगाई कि वह विपक्षी टीम के गोल के ठीक सामने जा गिरी और जैसे ही गेंद उछली, दूसरे खिलाड़ी ने उसको ऐसा हेड दिया कि गेंद सीधे गोल में थी। चारों ओर तालियों की गड़गड़ाहट थी। हमारी प्रसन्नता का ठिकाना न था। मैच समाप्त होने में अभी पाँच मिनट शेष थे। विपक्षी टीम की हिम्मत अब टूट चुकी थी। वे कुछ नहीं कर पा रहे थे। अंत में रेफरी ने एक लंबी सीटी बजाई और मैच समाप्त हो गया। अधिकांश दर्शक जा चुके थे परंतु हमारे स्कूल के खिलाड़ी अब वहाँ इकट्ठे हो रहे थे जहाँ पुरस्कार वितरण होना था।
उपसंहार:
पुरस्कार वितरण से पूर्व मुख्यमंत्री महोदय ने खेलों के महत्त्व पर प्रकाश डाला तथा खेलों की उपयोगिता बताई। अंत में उन्होंने टूर्नामेंट के अधिकारियों को धन्यवाद देते हुए हमारे कप्तान को ट्रॉफी प्रदान की। हमारे गोलकीपर को ईनाम दिया गया। सभी खिलाड़ियों ने हर्ष से करतल ध्वनि की। प्रधानाध्यापक जी ने मैच जीतने की खुशी में अगले दिन की छुट्टी कर दी। लगभग सात बजे हम अपने-अपने घर लौटे। फुटबाल का यह मैच मेरे जीवन की चिरस्मरणीय घटना रहेगी। आज भी जब कभी फुटबाल के खेल की चर्चा होती है तो इस मैच का दृश्य मेरी आँखों के सामने घूम जाता है।
40. दूरदर्शन के लाभ और हानियाँ
भूमिका:
‘दूरदर्शन’ शब्द दो शब्दों से बना है-‘दूर’ और ‘दर्शन’ अर्थात दूर स्थित वस्तु या दृश्य को घर बैठे हुए देखना। यह शब्द अंग्रेज़ी के Television का पर्यायवाची है। आधुनिक जीवन में दूरदर्शन महत्त्वपूर्ण स्थान ग्रहण कर चुका है। आज प्रत्येक मध्यवर्गीय परिवार में टी०वी० अवश्य मिलता है। जो स्थान पहले रेडियो का था, वही आज दूरदर्शन का है। यह निश्चय ही विज्ञान का अद्भुत चमत्कार है। कुछ विद्वानों का मत है कि महाभारत काल में दूरदर्शन का प्रयोग होता था। ऐसा कहा जाता है कि संजय ने घर बैठे राजा धृतराष्ट्र को महाभारत के युद्ध का आँखों देखा वर्णन किया था।
1. दूरदर्शन का आविष्कार:
सर्वप्रथम मारकोनी नाम के वैज्ञानिक ने रेडियो का आविष्कार किया। इससे देश-विदेश के कार्यक्रम सुनने को मिल जाते थे लेकिन दूरदर्शन पर हम प्रसारण केंद्र से बोलने वाले के चित्र को भी देख सकते हैं। एक प्रकार से दूरदर्शन रेडियो का ही परिवर्तित रूप है। वर्ष 1926 में स्कॉटलैंड के वैज्ञानिक जे०एल० ब्रेयर्ड ने टेलीविज़न का आविष्कार किया। सर्वप्रथम बी०बी०सी० लंदन से दूरदर्शन के कार्यक्रम प्रसारित होने लगे। भारत में 5 सितंबर, 1959 को दिल्ली में प्रथम दूरदर्शन केंद्र की स्थापना हुई। तत्पश्चात, लखनऊ, कोलकाता, चेन्नई, मुंबई आदि प्रमुख नगरों में दूरदर्शन केंद्रों की स्थापना हुई।
2. मनोरंजन एवं लोक:
शिक्षा का लोकप्रिय साधन-आधुनिक युग में दूरदर्शन मनोरंजन का प्रसिद्ध साधन बन गया है। जैसे ही रात होती है, लोगों के घरों में टेलीविज़न चलने लगते हैं। सारा दिन कठोर परिश्रम करने के बाद व्यक्ति अपने बच्चों सहित घर के सुखद वातावरण में मनोरंजन का आनंद लेता है। आजकल टेलीविज़न पर अनेक प्रकार के कार्यक्रम और समाचार नियमित रूप से आने लगे हैं। प्रतिदिन कोई-न-कोई सीरियल आता ही रहता है। इसी प्रकार से प्रातः और सायं दोनों समय हिंदी तथा अंग्रेज़ी में बारी-बारी से समाचार भी दिए जाते हैं।
दूरदर्शन जन-शिक्षा के प्रसार का लोकप्रिय साधन है। आजकल दूरदर्शन पर कृषि, विज्ञान, उद्योग, शिक्षा आदि सभी विषयों से संबंधित कार्यक्रम दिखाए जा रहे हैं। प्रतिदिन कृषि से संबंधित कार्यक्रम दिखाया जाता है। इससे खेती के साधनों में काफी सुधार हुआ है। इस कार्य के लिए ‘साइट’ का पूरा उपयोग किया जा रहा है। इसके द्वारा कृषि तथा ज्ञान-विज्ञान, समाज-शिक्षा तथा परिवार कल्याण के कार्यक्रम देहात में भी पहुंचाए जा रहे हैं।
3. दूरदर्शन के लाभ:
दूरदर्शन के कार्यक्रमों का दर्शकों पर अमिट प्रभाव पड़ रहा है। सामाजिक बुराइयों, रूढ़ियों, अंधविश्वासों तथा सड़ी-गली परंपराओं को दूर करने के लिए दूरदर्शन का उचित प्रयोग किया जा रहा है। इससे अन्य देशों की जानकारी भी दर्शकों को दी जाती है। इस प्रकार के अनेक कार्यक्रम दिखाए जा रहे हैं जो राष्ट्रीय एकता की भावना को सुदृढ़ करते हैं। अनेक प्रकार के तकनीकी प्रशिक्षणों का परिचय भी इसके द्वारा दिया जा रहा है।
4. विद्यार्थियों के लिए उपयोग:
देश के युवा वर्ग, विशेषकर, विद्यार्थियों के लिए दूरदर्शन के अनेक उपयोग किए जा रहे हैं। विश्वविद्यालय अनुदान आयोग द्वारा प्रतिदिन दोपहर को शिक्षा संबंधी कुछ कार्यक्रम प्रसारित किए जाते हैं। इनमें विज्ञान, अर्थशास्त्र, जीव-विज्ञान, भौतिकी, कंप्यूटर आदि अनेक विषयों के बारे में जानकारी दी जाती है। इससे विद्यार्थियों को काफी लाभ हुआ है। इसी प्रकार से भूगोल, इतिहास, समाजशास्त्र, मनोविज्ञान आदि पर भी कुछ कार्यक्रम दिए जा रहे हैं। पुनः इसी समय ‘घर-बाहर’ शीर्षक के अंतर्गत महिलाओं के लिए भी कार्यक्रम दिए जा रहे हैं। उदाहरण के रूप में, खाना पकाने की विभिन्न पद्धतियाँ, सिलाई, कढ़ाई, चित्रकारी आदि विषयों से महिला वर्ग को भी काफी लाभ पहुंच रहा है।
5. विभिन्न कार्यक्रम:
दूरदर्शन द्वारा प्रसारित नाटक, नृत्य, संगीत कला आदि कार्यक्रम दर्शकों को रुचि संपन्न बनाते हैं। क्रिकेट, हॉकी, टेनिस, फुटबाल, वॉलीबाल, बास्केटबाल आदि मैचों का सीधा प्रसारण दर्शकों में खेलों के प्रति रुचि उत्पन्न करता है। ‘युवा-मंच’ कार्यक्रम के अंतर्गत भाषण और वाद-विवाद प्रतियोगिताओं का आयोजन किया जाता है। प्रश्न-मंच के अंतर्गत पूछे गए प्रश्न दर्शकों के ज्ञान में वृद्धि करते हैं। इसी प्रकार से चित्रहार और चलचित्र हमें शिक्षित करने के साथ-साथ हमारा मनोरंजन भी करते हैं।
पिछले दो-तीन वर्षों में प्रसारित किए गए सीरियल काफी लोकप्रिय हुए हैं। उदाहरण के रूप में, ‘रामायण’ और ‘महाभारत’ द्वारा हमें प्राचीन धर्म तथा संस्कृति की जानकारी दी गई है। इसके अतिरिक्त, अनेक नाटक तथा शिक्षा प्रद कार्यक्रम तथा बच्चों के मनोरंजक कार्टून आदि ऐसे कार्यक्रम हैं जिन्होंने लोगों को भरपूर मनोरंजन दिया है और शिक्षित भी किया है। दूरदर्शन विज्ञापन का श्रेष्ठ साधन है। प्रत्येक कार्यक्रम के साथ कुछ-न-कुछ विज्ञापन अवश्य जुड़े रहते हैं।
उपसंहार:
दूरदर्शन की कुछ हानियाँ भी हैं। विद्यार्थी अपना अधिकतर समय टी०वी० के कार्यक्रम देखने में बरबाद कर देते हैं। इससे उनकी पढ़ाई की हानि होती है। दूरदर्शन के कारण लोगों में आपस में मिलना-जुलना कम हो गया है। लोग घंटों टी०वी० के सामने बैठे रहते हैं। इससे आँखों की रोशनी पर भी काफी दुष्प्रभाव पड़ता है। विशेषकर, रंगीन टी०वी० महँगा होने के साथ-साथ आँखों के लिए अधिक हानिकारक है किंतु फिर भी दूरदर्शन के उपयोग अधिक हैं और हानियाँ नगण्य । यदि हम इसका सही प्रयोग करें तो यह हमारे लिए अत्यधिक लाभकारी है।
41. हॉकी मैच
भूमिका:
अंग्रेज़ी की एक कहावत है “A sound mind lives in a sound body.” अर्थात स्वस्थ शरीर में ही स्वस्थ मस्तिष्क का निवास होता है। जब तक मानव का तन स्वस्थ नहीं है, तब तक मानव का मन भी स्वस्थ नहीं रह सकता। अतः खेल चाहे कोई भी हो, हमारे शरीर को शक्ति देता है, मन को गति देता है और बुद्धि को तेज करता है। यह भी कहा गया है “The War of Water Loo Was Won in the Playground.” अर्थात नेपोलियन ने वाटर लू का जो युद्ध जीता था, उसका अनुभव उसने खेल के मैदान से प्राप्त किया था। समूह में खेले गए खेलों से अपनत्व की भावना और मिलजुल कर काम करने की भावना बढ़ती है। इसके अतिरिक्त, कुछ खेल तो ऐसे होते हैं जो खिलाड़ी के तन-मन में प्राण फूंक देते हैं, दर्शकों के हृदय की धड़कनों को स्पंदित करते हैं। हॉकी, फुटबाल, क्रिकेट आदि इसी प्रकार के खेल हैं। ये खेल हमारे शरीर को स्फूर्ति, ताज़गी और चुस्ती प्रदान करते हैं। आज के भोले-भाले बच्चों को ही कल के सफल नागरिक बनना है।
1. हॉकी:
एक प्रिय खेल हॉकी मेरा प्रिय खेल है। प्रत्येक गाँव, नगर, स्कूल, कॉलेज आदि में यह खेल खेला जाता है। यह एक ऐसा खेल है जिसमें दर्शकों को दम साधकर बैठना पड़ता है। इसमें तो किसी भी क्षण कुछ भी हो सकता है। हॉकी हमारा राष्ट्रीय खेल है। अनेक वर्षों तक भारत हॉकी में विश्व-विजेता रहा है। यहाँ मैं उस हॉकी मैच का वर्णन करने जा रहा हूँ जो गत वर्ष हमारे नगर में हुआ था। इस बार कुरुक्षेत्र विश्वविद्यालय, कुरुक्षेत्र के हॉकी टूर्नामेंट का फाइनल मैच राजकीय महाविद्यालय करनाल तथा डी०ए०वी० महाविद्यालय, सोनीपत के बीच हुआ। खेल के लिए कर्ण स्टेडियम निश्चित किया गया। दोनों ही टीमें उच्च स्तर की थीं। इस मैच की घोषणा दो सप्ताह पहले ही कर दी गई। इसलिए इस मैच की सभी क्षेत्रों और गाँवों में चर्चा होने लगी। बच्चे, बूढ़े सभी इस मैच को देखने की लालसा लिए हुए थे। मैच दोपहर 12 बजे आरंभ किया गया।
2. खेल का आरंभ:
लगभग साढ़े ग्यारह बजे दर्शकों का आगमन शुरू हो गया। विशेष अतिथि के रूप में पुलिस अधीक्षक को आमंत्रित किया गया था। पौने बारह बजे वे भी आ पहुँचे। उस समय तक दोनों टीमें भी मैदान में पहुँच गई थीं। राजकीय महाविद्यालय, करनाल के खिलाड़ी सफेद वर्दी तथा उनकी विरोधी टीम के खिलाड़ी नीली वर्दी पहने थे। खिलाड़ियों की कमीजों के पीछे उनके नंबर लिखे हुए थे। ठीक बारह बजे रेफरी ने सीटी बजाई तथा दोनों टीमों के कप्तान रेफरी के सामने उपस्थित हुए। टॉस हुआ। करनाल की टीम ने टॉस जीता। तब दोनों टीमों ने अपना-अपना स्थान ले लिया। रेफरी की दूसरी सीटी बजते ही दोनों टीमों की अग्रिम पंक्ति के खिलाड़ी आगे आए तथा तीन बार हॉकी का स्पर्श करके खेल आरंभ कर दिया गया। खेल शुरू होने के 20 मिनट बाद तक कोई भी टीम गोल नहीं कर सकी। स्टेडियम में करनाल के विद्यार्थी अधिक थे। वे ऊँची आवाज़ में करनाल के खिलाड़ियों को प्रोत्साहित कर रहे थे। सोनीपत के खिलाड़ियों को प्रोत्साहित करने वाले भी पर्याप्त थे। इसी बीच सोनीपत के राजेश नामक खिलाड़ी ने बाईं ओर से एक लंबी हिट मारकर गेंद को गोल के अंदर पहुंचा दिया। सोनीपत के खिलाड़ियों के समर्थकों ने तालियाँ बजानी शुरू कर दी।
3. मध्यांतर की घोषणा:
करनाल के खिलाड़ी कुछ हतोत्साहित हो गए परंतु शीघ्र ही उन्होंने स्थिति को समझा तथा सक्रिय होकर खेलना शुरू कर दिया। वे जल्दी-से-जल्दी गोल करके अपनी टीम को बराबरी की स्थिति में लाना चाहते थे। इस कारण खिलाड़ियों में जोश आ गया। करनाल के खिलाड़ियों ने एक बार फिर गोल करने का प्रयत्न किया परंतु सोनीपत के गोलकीपर की कुशलता के आगे उनकी एक न चली। उसी समय एक लंबी सीटी बजी और रेफरी ने मध्यांतर की घोषणा कर दी।
सोनीपत के विद्यार्थियों ने राजेश को कंधों पर उठा लिया। सभी खिलाड़ी उसे शाबाशी देने लगे। उधर करनाल के कॉलेज के खेलाध्यक्ष अपने खिलाड़ियों को ढाढस बँधा रहे थे तथा कुछ नई तकनीक समझा रहे थे। फिर उनके हॉकी कोच ने कहा, “घबराने की कोई बात नहीं, इस बार हिम्मत करोगे तो एक की क्या बात, दो गोल कर दोगे।” इसके पश्चात सभी खिलाड़ियों को अल्पाहार दिया गया। एक लंबी सीटी के बाद खेल फिर आरंभ हो गया। इस बार करनाल के खिलाड़ी आक्रामक थे तथा सोनीपत के खिलाड़ियों ने बचाव की नीति अपनाई। सोनीपत की टीम का गोलकीपर इतना कुशल था कि गेंद आने से पहले ही गेंद की स्थिति को भाँप लेता था तथा उधर पहुँचकर, लेटकर अथवा उछलकर गेंद को पकड़ लेता था।
4. करनाल के खिलाड़ियों की विजय:
खेल समाप्त होने में अभी दस मिनट बाकी थे। करनाल के खिलाड़ी जी-जान से गेंद को गोल में पहुँचाने की कोशिश में थे। इसी बीच करनाल के खिलाड़ी मनोज ने ‘डी’ से गेंद को ऐसी हिट मारी कि गेंद गोलकीपर को चकमा देकर सीधे गोल में पहुंच गई। अब करनाल के खिलाड़ियों का उत्साह और भी बढ़ गया। सोनीपत की टीम ने भी आक्रामक रुख अपनाया तथा वे गेंद को गोल में पहुँचाने का भरसक प्रयत्न करने लगे। इसी समय करनाल के खिलाड़ी दीपक ने गेंद को सहसा हिट लगाया कि गेंद एक बार फिर से गोल में थी। अब करनाल के खिलाड़ियों की प्रसन्नता का ठिकाना न रहा। खेल का पलड़ा पलट गया अर्थात रेफरी ने एक लंबी सीटी बजाकर खेल समाप्ति की घोषणा कर दी।
उपसंहार:
अब सोनीपत के खिलाड़ी बहुत थके हुए तथा हतोत्साहित दिखाई दे रहे थे। करनाल के खिलाड़ियों के आनंद की सीमा नहीं थी। उन्होंने अपने साथियों को कंधों पर उठा लिया। वे नारेबाजी करने लगे। इसके बाद मुख्य अतिथि ने खेलों के मध्य खेल-भावना को बनाए रखने की आवश्यकता पर भाषण देते हुए करनाल के राजकीय महाविद्यालय की टीम के कप्तान को चैंपियन ट्रॉफी प्रदान की। डी०ए०वी० महाविद्यालय सोनीपत को भी ‘रनर्स कप’ की ट्रॉफी प्रदान की गई।

42. सदाचार का महत्त्व
भूमिका:
प्रत्येक मानव की यह इच्छा होती है कि समाज में उसका आदर-सम्मान हो। वस्तुतः आदर एवं सम्मान के बिना मनुष्य का अस्तित्व प्रकाश में नहीं आता। इसके लिए मानव अनेक साधनों का आश्रय लेता है। कोई धन के बल पर तो कोई सत्ता के बल पर प्रतिष्ठा प्राप्त करना चाहता है। इसी प्रकार से कोई विद्या का आश्रय लेता है तो कोई अपनी शक्ति का। कोई समाज-सेवा द्वारा तो कोई चरित्र के बल पर नाम कमाना चाहता है। इन सब साधनों में से सच्चरित्रता ही जीवन के समस्त गुणों, ऐश्वर्यों एवं समृद्धियों की आधारशिला है। यदि हम चरित्रवान हैं तो सभी हमारा आदर-सम्मान करते हैं। परंतु चरित्रहीन व्यक्ति समाज में निंदा और तिरस्कार का पात्र बनता है। वह समाज के लिए एक अभिशाप है। दुश्चरित्र व्यक्ति का जीवन अंधकारमय होता है, जबकि सच्चरित्र व्यक्ति ज्ञान एवं प्रकाश के उज्ज्वल वातावरण में विचरण करता है। प्रसिद्ध कवि मैथिलीशरण गुप्त ने उचित ही कहा है-
“खलों का कहीं भी नहीं स्वर्ग है भलों के लिये तो यही स्वर्ग है।
सुनो स्वर्ग क्या है ? सदाचार है। मनुष्यत्व ही मुक्ति का द्वार है।।”
1. सदाचार एवं चरित्र का महत्त्व:
अंग्रेजी की एक कहावत का भाव इस प्रकार है-यदि मनुष्य का धन नष्ट हुआ तो उसका कुछ भी नष्ट नहीं हुआ, यदि उसका स्वास्थ्य नष्ट हुआ तो कुछ नष्ट हुआ, परंतु यदि उसका चरित्र नष्ट हुआ तो उसका सब कुछ नष्ट हो गया। यह कहावत सच्चरित्रता के महत्त्व को दर्शाती है। सच्चरित्र बनने के लिए मनुष्य को सशिक्षा, सत्संगति और स्व + अनुभव की आवश्यकता होती है। परंतु यह जरूरी नहीं है। अनेक बार अशिक्षित व्यक्ति भी सत्संगति के कारण अच्छे चरित्र के देखे गए हैं। फिर भी बुद्धि का परिष्कार और विकास शिक्षा के बिना नहीं हो सकता। अच्छी शिक्षा के साथ-साथ सत्संगति भी अनिवार्य गुण है। संस्कृत में कहा भी गया है-
“संसर्ग जाः दोषगणाः भवन्ति।”
अर्थात् दोष और गुण संसर्ग से ही उत्पन्न होते हैं। मानव जिस प्रकार के लोगों के साथ रहेगा, उनकी विचारधारा, व्यसन या आदतें उसे अवश्य प्रभावित करेंगी। सत्संगति नीच व्यक्ति को भी उत्तम बना देती है। कीड़ा भी फूलों की संगति पाकर सज्जनों के सिर की शोभा बनता है। गोस्वामी तुलसीदास ने भी कहा है
“सठ सुधरहिं सत्संगति पाइ। पारस परस कुधांतु सुहाई।।”
2. स्वानुभव और चरित्र:
स्वानुभव भी मानव को चरित्रवान् बनाने में काफी सहायक होता है। जब बच्चा एक बार आग को छूकर अँगुली जला बैठता है तो दुबारा अग्नि से सावधान रहता है। दुष्कर्म करने वाला व्यक्ति जब दुष्ट कर्मों के परिणामों को जान लेता है तो वह दुबारा दुष्कर्म नहीं करता। व्यक्ति अपने अनुभव से यह जान लेता है कि अमुक काम करने से उसे यह फल भोगना पड़ा। यह बात बुरी है, उससे जीवन को हानि होती है अथवा झूठ बोलने से व्यक्ति को हानि होती है, लोगों में अपमान होता है तो वह झूठ बोलना छोड़ देता है। इस प्रकार व्यक्तिगत अनुभव भी मनुष्य को सच्चरित्रता की ओर ले जाते हैं। सच्चरित्रता लाने के लिए हमें अपने पूर्वजों के चरित्रों को पढ़ना चाहिए और उनका अनुसरण करना चाहिए।
3. विद्यार्थी जीवन और सच्चरित्रता:
विद्यार्थी जीवन सच्चरित्रता के विकास के लिए सर्वाधिक उचित समय है। युवाकाल में विद्यार्थी का मन काफी कोमल होता है। उसे जैसा चाहो वैसा बनाया जा सकता है। विद्यार्थी जीवन उस साधनावस्था का समय है जिसमें बालक अपने जीवनोपयोगी अनंत गुणों का संचय करता है। वह इस काल में अपने मन और मस्तिष्क का परिष्कार कर सकता है। इसी काल में संयम और नियमों का पालन करना सीखता है। समय पर सोना, समय पर उठना, ब्रह्मचर्य का पालन करना, नियमित रूप से अध्ययन करना, सज्जनों की संगति करना आदि विद्यार्थी को चरित्रवान बनाते हैं।
सच्चरित्रता से मानव अनेक प्रकार से लाभान्वित होता है। सच्चरित्रता किसी विशेष प्रवृत्ति की बोधक भी नहीं है। अनेक गुण जैसे-सत्य भाषण, उदारता, सहृदयता, विनम्रता, दयालुता, सुशीलता, सहानुभूतिपरता आदि समन्वित रूप से सच्चरित्रता कहलाते हैं। जो व्यक्ति चरित्रवान है, वह समाज में प्रतिष्ठा प्राप्त करता है, उसे समाज में आदर और सम्मान मिलता है, इस लोक में उसकी कीर्ति फैलती है तथा मर कर भी उसका नाम अमर हो जाता है। सच्चरित्र व्यक्ति की उच्च भावनाएँ तथा दृढ़ संकल्प हमेशा संसार में विचरण करते रहते हैं। सच्चरित्रता से व्यक्ति में शूरवीरता, धीरता, निर्भयता आदि गुण स्वतः आ जाते हैं, परंतु सदाचार एवं सच्चरित्रता के अभाव में मानव कदम-कदम पर ठोकरें खाता है, अपमानित होता है और पशु-तुल्य जीवन व्यतीत करता है।
मर्यादा पुरुषोत्तम भगवान् राम की सच्चरित्रता किसी से भी छिपी नहीं है। भारत के लाखों-करोड़ों नर-नारी उनके सच्चरित्र का अनुसरण करके अपने जीवन को पावन करते हैं। शिवाजी और महाराणा प्रताप की चारित्रिक विशेषताएँ आज भी भारतीय जन-साधारण का मार्ग-दर्शन करती हैं। इसी प्रकार से आधुनिक काल में लोकमान्य तिलक, मदन मोहन मालवीय, महात्मा गाँधी, सुभाषचंद्र बोस आदि महान् विभूतियाँ भारतीयों के लिए अनुकरणीय हैं। इन महान् आत्माओं ने भारत माता की परतंत्रता की बेड़ियों को छिन्न-भिन्न करने में अपने जीवन का बलिदान दिया। निश्चय ही सदाचार मानव को महान् बनाता है, एक साधारण व्यक्ति को भी युग-पुरुष बनाता है और उसे यश प्रदान करता है। संस्कृत में एक श्लोक है-
आचाराल्लभते आयुः आचारादीप्सिताः प्रजाः।
आचाराल्लभते ख्याति, आचाराल्लभते धनम्।।
4. सदाचार के लाभ:
चरित्रवान बनना प्रत्येक नागरिक का कर्तव्य है। चरित्रता से मानव अपना कल्याण तो करता ही है, समाज का हित भी करता है। इससे वह सुख और समृद्धि को प्राप्त करता है। सच्चरित्रता से हम आज देश की असंख्य समस्याओं का हल निकाल सकते हैं। जिस राष्ट्र के नागरिकों का चरित्र भ्रष्ट है, वह राष्ट्र कदापि विकास नहीं कर सकता। हमारे देश में फैली हुई गरीबी की जड़ भ्रष्टाचार एवं चरित्रहीनता है। प्रमुख आलोचक एवं साहित्यकार डॉ० हजारीप्रसाद द्विवेदी के शब्दों में-“चरित्र बल हमारी प्रध न समस्या है। हमारे महान् नेता महात्मा गाँधी ने कूटनीतिक चातुर्य को बड़ा नहीं समझा, बुद्धि-विकास को बड़ा नहीं माना, चरित्र बल को ही महत्त्व दिया। आज हमें सबसे अधिक इसी बात को सोचना है। यह चरित्र-बल भी केवल एक व्यक्ति का नहीं, समूचे देश का होना चाहिए।”
उपसंहार:
अतः आज हमारा प्रमुख कर्त्तव्य यही है कि हम चरित्रवान् बनें। विशेषकर, छात्र-छात्राओं को तो इस दिशा में भागीरथ प्रयत्न करने चाहिएँ, क्योंकि उन्हीं पर हमारे देश का भविष्य निर्भर है।
43. भारतीय समाज में नारी का स्थान
भूमिका:
‘यत्र नार्यस्तु पूज्यन्ते रमन्ते तत्र देवताः’ अर्थात जहाँ स्त्रियों की पूजा होती है, वहाँ देवता निवास करते हैं। यहाँ स्त्रियों की पूजा का अर्थ है-स्त्रियों के मान-मर्यादा की रक्षा अथवा उनके अधिकारों की समुचित रक्षा । यह पंक्ति इस बात को भी सिद्ध करती है कि प्राचीन भारत में नारी-जाति का स्थान गौरवपूर्ण था। उस समय नारी को गृह-लक्ष्मी और गृह-देवी के रूप में जाना जाता था। उन्हें पुरुषों के समान शिक्षा मिलती थी और पुरुषों के समान ही उनको अधिकार प्राप्त थे। प्रत्येक परिवार में नारी को सम्मान की दृष्टि से देखा जाता था। जीवन के बड़े-से-बड़े धार्मिक काम में भी नारी को पुरुष के बराबर स्थान दिया जाता था।
1. प्राचीन काल में नारी:
हमें ऐसे अनेक उदाहरण मिलते हैं जो यह सिद्ध करते हैं कि प्राचीन काल में नारी तथा पुरुष के अधिकार और कर्तव्य बराबर थे। कैकेयी ने बड़ी कुशलता से युद्ध में फंसे हुए अपने पति राजा दशरथ के रथ को निकाला था। इसी प्रकार से द्रोपदी निरंतर अपने पतियों को विद्वतापूर्ण परामर्श देती थी। द्रोपदी स्वयंवर भी यह सिद्ध करता है कि उस काल में स्त्रियों को अपना पति वरण करने की भी स्वतंत्रता थी। मैत्रेयी, शकुंतला, सीता, अनुसूइया, दमयंती, सावित्री आदि असंख्य नारियाँ इस तथ्य को सिद्ध करती हैं कि प्राचीन भारत में नारियों को उचित सम्मान प्राप्त था।
2. मध्यकाल में नारी:
समय के परिवर्तन के साथ-साथ सभी कुछ बदलता है। परतंत्रता के युग के आरंभ के साथ-साथ नारी-परतंत्रता का भी युग आरंभ हुआ। स्त्रियों का प्रेम और बलिदान ही उनके लिए विष बन गया। समाज के ही ठेकेदारों ने नारी का बराबरी का दर्जा समाप्त कर दिया। अतः उसका पद गौण बन गया। उसे पर्दे में कैद रखा जाने लगा। उसका शिक्षा का अधिकार भी उससे छीन लिया गया। अब नारी का क्षेत्र घर की चारदीवारी तक ही सीमित हो गया। इस परिवर्तन के पीछे तत्कालीन राजनीतिक परिस्थितियाँ थीं। वस्तुतः उस समय की विदेशी सरकार की पक्षपातपूर्ण नीति के कारण हिंदुओं की बहु-बेटियों की इज़्ज़त सुरक्षित नहीं थी। अतः अनमेल विवाह, बाल-विवाह तथा सती-प्रथा जैसी बुराइयाँ पनपने लगीं।
3. वर्तमान स्थिति:
स्वतंत्रता-प्राप्ति के बाद नारी जाति को ऊँचा उठाने का भरसक प्रयास हुआ है। संविधान द्वारा उसे पुरुष के बराबर का दर्जा दिया गया है। प्रत्येक लड़की को अपने पिता की संपत्ति से बराबर का हिस्सा पाने की व्यवस्था है। आज स्त्रियाँ स्कूलों और कॉलेजों में शिक्षा प्राप्त कर रही हैं और प्रत्येक व्यवसाय में पुरुषों के साथ कंधे-से-कंधा मिलाकर काम कर रही हैं। राजनीति में भी स्त्रियाँ खुलकर भाग लेने लगी हैं। सरकार की ओर से इस बात का भरसक प्रयास किया जा रहा है कि नारी को समाज में उचित सम्मान मिले।
4. आधुनिक नारी की समस्याएँ:
नारी के उत्थान के नारे तो बहुत लगाए जा रहे हैं लेकिन नारी का स्थान कितना ऊँचा उठा है, यह आज के समाचार-पत्रों से ज्ञात हो जाता है। आज भी कन्या को ‘पराया धन’ की संज्ञा दी जाती है। दहेज प्रथा के कारण न जाने कितनी नारियाँ जीवित ही आग की भेंट कर दी जाती हैं। लोगों की मानसिकता में कोई विशेष परिवर्तन दिखाई नहीं देता। बड़े-बड़े नगरों से निकलकर अगर बाहर गाँवों में झाँका जाए तो ज्ञात होगा कि वहाँ नारी की स्वतंत्रता नाम मात्र की है। आज भी लोग अपनी लड़कियों को शिक्षित करने से डरते हैं। कुछ लोग तो लड़की को बचपन से ही बोझ मानते हैं। घर में लड़का पैदा हो तो खुशियाँ मनाई जाती हैं परंतु लड़की पैदा होते ही घर में निराशा का वातावरण छा जाता है।
5. सुधार के प्रयत्न:
आजकल नारी वर्ग के उत्थान के प्रयास भी चल रहे हैं। संयुक्त राष्ट्र संघ द्वारा कुछ वर्ष पहले अंतर्राष्ट्रीय महिला वर्ष मनाया गया था। भूतपूर्व प्रधानमंत्री श्रीमती इंदिरा गांधी ने स्त्रियों के उत्थान के लिए अनेक कदम उठाए थे। सबसे पहली बात तो यह है कि लड़कियों को समुचित शिक्षा दी जानी चाहिए। नारियाँ शिक्षित होंगी तो वे अपने अधिकारों के प्रति सजग रहेंगी। इससे उनको आर्थिक स्वतंत्रता मिलने में भी आसानी होगी। अगर स्त्रियाँ अपने पैरों पर खड़ी हों तो वे मज़बूती से उपर्युक्त समस्याओं का सामना कर सकती हैं। अनमेल विवाह एवं बाल-विवाह भी आज नहीं के बराबर हैं।
उपसंहार:
प्रसन्नता की बात है कि हमारी वर्तमान सरकार इस दिशा में काफी प्रयत्नशील है। काम और वेतन की समानता के संबंध में हमारे संविधान में स्पष्ट निर्देश हैं। धीरे-धीरे स्त्रियाँ पर्दे की कैद से बाहर निकल रही हैं। स्कूलों, कॉलेजों, हस्पतालों, बैंकों तथा अन्य कार्यालयों में स्त्रियाँ काम करने लगी हैं। इस संबंध में पुरुषों को भी उदार दृष्टिकोण अपनाना चाहिए। किसी कवि ने कहा है-
नारी निंदक निपट अनारी, ते जन ताड़न के अधिकारी।
सबसे बड़ी बात यह है कि उनके लिए उपयोगी साहित्य तैयार किया जाए ताकि नारी को समाज में वही सम्मान मिले जो प्राचीन काल में सीता, अनुसूइया, सावित्री आदि स्त्रियों को प्राप्त था। तभी हमारा देश विकास कर सकता है।
44. शिक्षा में खेल-कूद का महत्त्व
संकेत : खेल-कूद का महत्त्व, शिक्षा व खेल-एक-दूसरे के पूरक, खेलों के प्रकार, लाभ, सर्वांगीण विकास। ग्रीक दार्शनिक प्लेटो ने कहा है-
“स्वस्थ शरीर वाला व्यक्ति ही भली प्रकार अपने
मस्तिष्क का विकास कर सकता है।”
शिक्षा का अभिप्राय केवल पुस्तकों का ज्ञान अर्जित करना ही नहीं, अपितु शिक्षा का उद्देश्य व्यक्ति के मानसिक विकास के साथ-साथ उसके शारीरिक विकास की ओर भी ध्यान देना है। अच्छे स्वास्थ्य के लिए खेल-कूद का महत्त्व किसी से कम नहीं। यदि शिक्षा से बुद्धि का विकास होता है तो खेलों से शरीर का। ईश्वर ने मनुष्य को शारीरिक, मानसिक तथा आत्मिक-तीन शक्तियाँ प्रदान की हैं। जीवन में सफलता प्राप्त करने के लिए इन तीनों का संतुलित रूप से विकास होना आवश्यक है।
शिक्षा तथा खेल एक-दूसरे के पूरक हैं। एक के बिना दूसरा अपंग है। शिक्षा यदि परिश्रम लगन, संयम, धैर्य तथा भाईचारे का उपदेश देती है तो खेल के मैदान में विद्यार्थी इन गुणों को वास्तविक रूप में अपनाता है। जैसे कहा भी गया है-‘स्वस्थ शरीर में ही स्वस्थ मस्तिष्क का निवास होता है।’
यदि व्यक्ति का शरीर ही स्वस्थ नहीं है तो संसार के सभी सुख तथा भोग-विलास बेकार हैं। एक स्वस्थ शरीर ही सभी सखों का भोग कर सकता है। रोगी व्यक्ति सदा उदास तथा अशांत रहता है। उसे कोई भी कार्य करने में आनंद प्राप्त नहीं होता है। स्वस्थ व्यक्ति सभी कार्य प्रसन्नचित्त होकर करता है। इस प्रकार स्वस्थ शरीर एक नियामत है।
खेलना बच्चों के स्वभाव में होता है। आज की शिक्षा प्रणाली में इस बात पर जोर दिया जा रहा है कि बच्चों को पुस्तकों की अपेक्षा खेलों के माध्यम से शिक्षा ग्रहण करवाई जाएँ। आज शिक्षा-विदों ने खेलों को शिक्षा का विषय बना दिया है, ताकि विद्यार्थी खेल-खेल में ही जीवन के सभी मूल्यों को सीख जाएं और अपने जीवन में अपनाएँ।
अच्छे स्वास्थ्य की प्राप्ति के लिए अनेक प्रकार के खेलों का सहारा लिया जा सकता है। दौड़ना, कूदना, कबड्डी, टेनिस, हॉकी, फुटबॉल, क्रिकेट, जिमनास्टिक, योगाभ्यास आदि से उत्तम व्यायाम होता है। इनमें से कुछ आउटडोर होते हैं और कुछ इंडोर। आउटडोर खेल खुले मैदान में खेले जाते हैं। इंडोर खेलों को घर के अंदर भी खेला जा सकता है। इनमें कैरम, शतरंज, टेबलटेनिस आदि हैं। न केवल अपने देश में, बल्कि विदेशों में भी खेलों का आयोजन होता रहता है। खेलों से न केवल खिलाड़ियों का अपितु देखने वालों का भी भरपूर मनोरंजन होता है। आज रेडियो तथा टी०वी० आदि माध्यमों के विकास से हम आँखों देखा हाल अथवा सीधा प्रसारण देख सकते हैं तथा उभरते खिलाड़ी प्रेरणा प्राप्त कर सकते हैं।
आज के युग में खेल न केवल मनोरंजन का साधन हैं, बल्कि इनका सामाजिक तथा राष्ट्रीय महत्त्व भी है। इनमें स्वास्थ्य प्राप्ति के साथ-साथ मनोरंजन भी होता है। इनसे छात्रों में अनुशासन की भावना आती है। खेल के मैदान में छात्रों को नियमों में बंधकर खेलना पड़ता है, जिससे आपसी सहयोग और मेल-जोल की भावना का भी विकास होता है। खेल-कूद मनुष्य में साहस और उत्साह की भावना पैदा करते हैं। विजय तथा पराजय दोनों स्थितियों को खिलाड़ी प्रसन्नतापूर्वक स्वीकार करता है। खेल-कूद से शरीर में स्फूर्ति आती है, बुद्धि का विकास होता है तथा रक्त संचार बढ़ता है। खेलों में भाग लेने से आपसी मन-मुटाव समाप्त हो जाता है तथा खेल भावना का विकास होना है। जीविका-अर्जन में भी खेलों का बहुत महत्त्व है।
आज खेलों में ऊँचा स्थान प्राप्त खिलाड़ी संपन्न व्यक्तियों में गिने जाते हैं। किसी भी खेल में मान्यता प्राप्त खिलाड़ी को ऊँचे पद पर आसीन कर दिया जाता है तथा उसे सभी सुविधाएँ उपलब्ध कराई जाती हैं। खेल राष्ट्रीय एकता की भावना को भी विकसित करते हैं। राष्ट्रीय अथवा अंतर्राष्ट्रीय स्तर पर खेलने वाले खिलाड़ी अपना तथा अपने देश का नाम रोशन करते हैं।
भारत जैसे विकासशील देश के लिए आवश्यकता है कि प्रत्येक युवक-युवती खेलों में भाग ले तथा श्रेष्ठता प्राप्त करने का प्रयास करे। आज का युग प्रतियोगिता का युग है और इसी दौड़ में किताबी कीड़ा बनना पर्याप्त नहीं, बल्कि स्वस्थ, सफल तथा उन्नत व्यक्ति बनने के लिए आवश्यक है मानसिक, शारीरिक तथा आत्मिक विकास ।

45. बेरोज़गारी की समस्या
अथवा
बेकारी की समस्या
भूमिका:
आज भारत के सम्मुख अनेक समस्याएँ हैं; जैसे महँगाई, बेरोज़गारी, आतंकवाद आदि। इन सब में बेरोज़गारी की समस्या प्रमुख है। बेरोज़गारी की समस्या ने परिवारों की आर्थिक दशा को खोखला कर दिया है। आज हम स्वतंत्र अवश्य हैं परंतु हम अभी आर्थिक दृष्टि से समृद्ध नहीं हुए हैं। चारों ओर चोरी-डकैती, छीना-झपटी, लूटमार और कत्ल के समाचार सुनाई पड़ते हैं। आज देश में जगह-जगह पर उपद्रव एवं हड़तालें हो रही हैं। मनुष्य के जीवन से आनंद और उल्लास न जाने कहाँ चले गए हैं। प्रत्येक मानव को अपनी तथा अपने परिवार की रोटियों की चिंता है चाहे उनका उपार्जन सदाचार से हो या दुराचार से। निरक्षर तो फिर भी अपना पेट भर लेते हैं परंतु साक्षर वर्ग की आज बुरी हालत है।
बेकारी का चरमोत्कर्ष तो उस समय दिखाई दिया, जब रुड़की विश्वविद्यालय में पूर्ण प्रशिक्षित इंजीनियरों को उपाधि देने हेतु 1967 के दीक्षांत समारोह में भारत की भूतपूर्व प्रधानमंत्री श्रीमती इंदिरा गांधी जैसे ही भाषण देने के लिए खड़ी हुईं, उसी समय लगभग एक हज़ार इंजीनियरों ने खड़े होकर एक स्वर में कहा, ‘हमें भाषण नहीं, नौकरी चाहिए।’ प्रधानमंत्री के पास उस समय कोई उत्तर न था।
हमारे देश में बेकारी के प्रमुख कारण निम्नलिखित हैं-
1. जनसंख्या में वृद्धि:
हमारे देश में जनसंख्या दिन-प्रतिदिन बढ़ती जा रही है। वर्ष भर में जितने व्यक्तियों को रोजगार मिलता है, उससे कई गुणा अधिक बेकारों की संख्या बढ़ जाती है। अनेक अप्राकृतिक उपायों के बावज़द भी जनसंख्या बढ़ती ही जा रही है। जनसंख्या की वृद्धि के फलस्वरूप ही बेरोज़गारी बढ़ती जा रही है।
2. अधूरी शिक्षा प्रणाली:
हमारी शिक्षा प्रणाली अधूरी एवं दोषपूर्ण है। परतंत्र भारत में अंग्रेज़ों ने ऐसी शिक्षा-पद्धति केवल क्लर्कों को पैदा करने के लिए ही लागू की थी परंतु अब स्वतंत्र भारत में समयानुसार समस्याएँ भी बदल गई हैं। एक बार पंडित जवाहरलाल नेहरू ने कहा था, “प्रतिवर्ष नौ लाख पढ़े-लिखे लोग नौकरी के लिए तैयार हो जाते हैं जबकि हमारे पास नौकरियाँ शतांश के लिए भी नहीं हैं। हमें बी०ए० नहीं चाहिएँ, वैज्ञानिक और तकनीकी विशेषज्ञ चाहिएँ।” उनका यह कथन हमारी शिक्षा-प्रणाली की ओर संकेत करता है।
3. उद्योग:
धंधों की अवनति-प्राचीन काल में हमारे देश के प्रत्येक घर में कोई-न-कोई उद्योग-धंधा चलता था। कहीं चरखा काता जाता था, कहीं खिलौने बनते थे तो कहीं गुड़। इन्हीं उद्योग-धंधों से लोग रोजी-रोटी कमाते थे परंतु अंग्रेज़ों ने अपने स्वार्थ के लिए इन्हें नष्ट कर दिया जिससे लोगों में आत्मनिर्भरता समाप्त हो गई। इसीलिए पढ़े-लिखे लोग भूखों मरना पसंद करते हैं परंतु वे मजदूरी करना पसंद नहीं करते। वे सोचते हैं कि दुनिया कहेगी कि पढ़-लिखकर मजदूरी कर रहा है। यही मिथ्या स्वाभिमान मनुष्य को कुछ करने नहीं देता। पढ़ाई-लिखाई ने मजदूरी करने को मना तो नहीं किया है।
4. सामाजिक तथा धार्मिक मनोवृत्ति:
हमारे समाज की धार्मिक तथा सामाजिक मनोवृत्ति भी बेकारी को बढ़ावा देती है। वर्तमान युग में साधु-संन्यासियों को भिक्षा देना पुण्य समझा जाता है। दानियों की उदारता देखकर बहुत से स्वस्थ व्यक्ति भी भिक्षावृत्ति पर उतर आते हैं। इस प्रकार, बेकारों की संख्या बढ़ती जा रही है।
5. सरकारी विभागों में छंटनी:
हमारे यहाँ सामाजिक नियम कुछ ऐसे हैं कि वर्ण-व्यवस्था के अनुसार विशेष-विशेष वर्गों के लिए विशेष-विशेष कार्य हैं। सरकारी विभागों में भी वर्ण-व्यवस्था के अनुसार काम दिए जाते हैं। यदि उन्हें काम मिले तो करें अन्यथा हाथ-पर-हाथ धरकर बैठे रहें। इस प्रकार की सामाजिक व्यवस्था से भी बेरोज़गारी को बढ़ावा मिलता है। बेकारी के कारण युवा-आक्रोश और असंतोष ने समाज में अव्यवस्था एवं अराजकता उत्पन्न कर दी है।
निम्नलिखित उपायों द्वारा बेकारी दूर की जा सकती है-
6. जनसंख्या वृद्धि पर रोक और शिक्षा:
देश में जनसंख्या वृद्धि को रोककर बेकारी की समस्या को काफी सीमा तक हल किया जा सकता है। बढ़ती हुई आबादी को रोकने के लिए विवाह की आयु का नियम कठोरता से लागू करना चाहिए। साथ ही, . हमें शिक्षा-पद्धति में सुधार लाना होगा। शिक्षा को व्यावहारिक बनाना होगा। विद्यार्थियों में आरंभ से ही स्वावलंबन की भावना पैदा करनी होगी।
7. उद्योग एवं कृषि का विकास:
सरकार को कुटीर उद्योगों तथा घरेलू दस्तकारी को प्रोत्साहन देना चाहिए; जैसे सूत कातना, कागज़ बनाना, शहद तैयार करना आदि। उद्योग-धंधों एवं धन की उचित व्यवस्था न होने के कारण गाँव के बड़े-बड़े कारीगर भी बेकार हैं। भारतीय किसान कृषि के लिए वर्षा पर आश्रित रहता है। अतः उसे अपने खाली समय में कुटीर उद्योग चलाने चाहिएँ। इससे उसकी तथा देश की आर्थिक दशा सुदृढ़ होगी। आजकल हज़ारों ग्रामीण शहरों में आकर बसते जा रहे हैं, इससे बेकारी की समस्या बढ़ती जा रही है। – उपसंहार केंद्र तथा राज्य सरकारें इस दिशा में व्यापक पग उठा रही हैं। चाहे हमारी सरकार बेकारी पर काबू पाने में अभी सफल नहीं हो सकी है परंतु ऐसा विश्वास किया जाता है कि आने वाली पीढ़ियों के लिए रोज़गार के साधन जुटाकर बेकारी की समस्या को सुलझाने के लिए सरकार विस्तृत पग उठाएगी। अब वह दिन दूर नहीं, जब देश के प्रत्येक नागरिक को काम मिलेगा और देश का भविष्य उज्ज्वल होगा।
46. मद्य-निषेध
भूमिका:
संघर्ष मानव जीवन का दूसरा नाम है। इसी संघर्ष में से गुज़रकर मानव कुंदन के समान शुद्ध और पावन बन जाता है लेकिन जिन लोगों का हृदय कमज़ोर होता है अथवा जिनका निश्चय सुदृढ़ नहीं होता है, वे संघर्ष के सामने घुटने टेक देते हैं। वे अपनी असफलता से मुँह छिपाने के लिए नशे की शरण लेते हैं। कहने को तो वे कह देते हैं कि हम गम गलत करने के लिए शराब पीते हैं।
इससे हमारे मन को राहत मिलती है। दुःखों और चिंताओं से कुछ समय के लिए मुक्ति मिलती है लेकिन क्या सचमुच नशा करने से आदमी दुखों से मुक्त हो सकता है ? यदि ऐसा होता तो संसार में संभवतः कोई भी दुखी और चिंताग्रस्त नहीं होता।
1. सवृत्तियाँ बनाम दुष्प्रवृत्तियाँ:
मानव जीवन यद्यपि निर्मल है, उसमें सात्विकता, सज्जनता, उदारता और चरित्र का उत्कर्ष है। वह स्वयं का ही नहीं अपितु अपने संपर्क में आने वालों का भी उद्धार कर सकता है। इसके विपरीत, तामसी वृत्तियाँ मनुष्य को पतनोन्मुख करती हैं, उसका ह्रास करती हैं। एक पतनोन्मुखी व्यक्ति मानव समाज और राष्ट्र के लिए भी घातक होता है। इसीलिए तो समाज-सुधारक और धार्मिक नेता समय-समय पर दुष्प्रवृत्तियों की निंदा करते हैं और उनसे बचने की प्रेरणा देते हैं। वस्तुतः मदिरापान तथा नशा सब बुराइयों की जड़ है। कबीर ने भी एक स्थल पर कहा है-
“ऊँचे कुल का जनमिया, जे करनी ऊँच न होइ।
सुबरन कलस सुरा भरा, साधू निंदा सोई॥”
2. मदिरापान बुराइयों की जड़:
एक विद्वान ने सत्य ही कहा है कि मदिरापान सभी बुराइयों की जड़ है। यह मनुष्य को असंतुलित बनाती है। उसमें तामसी वृत्तियों को उत्पन्न करती है। शराबी व्यक्ति से किसी भी समाज-विरोधी बुराई की अपेक्षा की जा सकती है। इसलिए हमारे शास्त्रों में मदिरापान को पाप समझा गया है। यह कोई सामान्य पाप नहीं अपितु सभी पापों का मूल है। एक लेखक ने ठीक ही कहा है-बाढ़ ने भी इतने मनुष्यों को नहीं डुबोया, जितनों को शराब ने।
आरंभ में व्यक्ति शौकिया तौर पर नशा करता है। मित्र लोग मुफ्त में शराब पिलाते हैं। अथवा कुछ लोग यह बहाना करेंगे कि वे तो औषधि के रूप में शराब पी रहे हैं परंतु धीरे-धीरे उन्हें शराब पीने की लत पड़ जाती है। एक बार शराब पीने की आदत पड़ जाए तो फिर छूटती नहीं। शराबी व्यक्ति विवेकशून्य होकर अनुचित, असंगत और अनर्गल प्रलाप करने लगता है। उसकी चेष्टाओं में अश्लीलता का समावेश हो जाता है। वह अपनी समूची शिक्षा, सभ्यता, संस्कार और सामाजिक मर्यादा का उल्लंघन करके अनुचित व्यवहार करने लगता है। गाली-गलौच और मारपीट तो उसके लिए सामान्य-सी बात हो जाती है।
3. मदिरापान पारिवारिक बरबादी का कारण:
यूँ तो अल्प मात्रा में नशा औषधि का भी काम करता है। डॉक्टर और वैद्य भी इसकी सलाह देते हैं लेकिन अति तो प्रत्येक वस्तु की बुरी है-‘अति सर्वत्र वर्जयेत्।’ अति से यही शराब विष बन जाती है। नशे के चक्कर में बड़े-बड़े घरानों को उजड़ते हुए देखा गया है। जिस पैसे को खून और पसीना एक करके मनुष्य प्रातः से सायं तक कमाता है और जिसके आने की प्रतीक्षा में बच्चे और पत्नी घर पर बैठे होते हैं, वह उस पैसे की शराब पीकर लड़खड़ाता हुआ घर आता है। पड़ोसी उसे देखकर हँसते हैं, मोहल्ले वाले उसकी निंदा करते हैं लेकिन बेचारी पत्नी आह भरकर रह जाती है। उसे तो एक ही डर है-शराबी पति आकर उसे और उसके बच्चों को पीटेगा। वह बेचारी दिल पर पत्थर रखे सारा जीवन काट देती है।
4. विषैली शराब के दुष्परिणाम:
विषैली शराब के दुष्परिणाम तो प्रतिदिन समाचार-पत्रों में छपते रहते हैं। आर्थिक संकट की स्थिति में शराबी घटिया शराब पीने लगता है। उधर शराब बेचने वाले अधिक आय के चक्कर में शराब में मिलावट कर देते हैं। इस प्रकार की विषैली शराब हज़ारों की जान ले चुकी है परंतु यह प्रक्रिया समाप्त नहीं होती। पुलिस और सरकार के नाक के नीचे यह धंधा चल रहा है। लोग पी रहे हैं, मर रहे हैं। समाचार-पत्र छाप रहे हैं, सब कुछ हो रहा है। सिनेमा तक में इस समस्या को बार-बार उछाला गया है लेकिन सरकार है कि उसके कान पर जूं तक नहीं रेंगती। कारण यह है कि विषैली शराब बेचने वालों को राजनीतिज्ञों का संरक्षण प्राप्त है।
5. नशाबंदी कानून के लाभ:
पश्चिमी सभ्यता के अंधानुकरण ने शराब सेवन को बढ़ावा दिया है। शराब पश्चिमी राष्ट्रों के लोगों के लिए अनुकूल है क्योंकि वहाँ सरदी अधिक पड़ती है। भारत एक कृषि-प्रधान देश है। शराब यहाँ के वातावरण के सर्वथा प्रतिकूल है। स्वतंत्रता-पूर्व काल में मदिरालयों के सामने धरने दिए जाते थे। गांधी जी ने इसके विरुद्ध ज़ोरदार अभियान भी आरंभ किया। अतः नशे की हानियों को सामने रखकर भारत सरकार ने देश के समुचित उन्नयन के लिए नशाबंदी कानून बनाया। इस कानून के अनुसार केवल कुछ लाइसेंस प्राप्त व्यक्ति ही नशीली वस्तुओं, विशेषकर, शराब को बेच सकते थे। इन लाइसेंस प्राप्त व्यक्तियों के अतिरिक्त और कोई शराब नहीं बेच सकता। मद्य-निषेध के लिए समय-समय पर अनेक प्रयास हुए हैं।
उपसंहार:
देश में पूर्ण नशाबंदी से अनेक लाभ हो सकते हैं। हमारे बड़े-बड़े नेता चारित्रिक पतन के लिए बार-बार चीखते और चिल्लाते हैं। नशाबंदी लागू करने से उन्हें फिर नहीं रोना पड़ेगा। देश स्वयं सुधरेगा। हज़ारों घर उजड़ने से बच जाएँगे। देश सामूहिक शक्ति प्राप्त करेगा। विलासिता के स्थान पर कर्मण्यता और अध्यवसाय बढ़ेगा। उदासी और अकर्मण्यता दूर हो जाएँगे। लोग चरित्रवान और बलवान बनेंगे। तामसी वृत्ति समाप्त होकर सात्विकी वृत्ति बढ़ेगी। धर्म और कर्तव्य की भावना विकसित होगी।
47. प्राकृतिक प्रकोप : सुनामी लहरें
प्रकृति का मनोरम रूप जहाँ मनुष्य के विकास के लिए सदा सहायक है, वहाँ भयंकर रूप उसके विनाश का कारण भी बनता रहा है। मानव जहाँ आदिकाल से प्रकृति की गोद में खेलकूद कर बड़ा होता है, वही गोद कभी-कभी उसको निगल भी जाती है। अतिवृष्टि (अत्यधिक वर्षा), बाढ़, भूकंप, समुद्री तूफान, आँधी आदि प्राकृतिक प्रकोप के विभिन्न रूप हैं। 26 दिसंबर, 2004 को समुद्र में उठी भयंकर लहरें भी विनाशकारी प्राकृतिक प्रकोप था।
महाविनाशकारी ‘सुनामी’ की उत्पत्ति भी वास्तव में समुद्रतल में भूकंप आने से होती है। समुद्र के भीतर भूकंप, ज्वालामुखी विस्फोट या भू-स्खलन के कारण यदि बड़े स्तर पर पृथ्वी की सतहें (प्लेटें) खिसकती हैं तो इससे सतह पर 50 से 100 फुट ऊँची लहरें, 800 कि०मी० प्रति घण्टे की तीव्र गति से तटों की ओर दौड़ने लग जाती हैं। पूर्णिमा की रात्रि को तो ये लहरें और भी भयंकर रूप धारण कर लेती हैं। 26 दिसंबर को भूकंप के कारण उठी इन लहरों ने भयंकर रूप धारण करके लाखों लोगों की जाने ले ली और अरबों की सम्पत्ति को नष्ट कर डाला। आज भी उस दृश्य के विषय में सोचकर दिल दहल जाता है।
वस्तुतः ‘सुनामी’ शब्द जापानी भाषा का है। जहाँ अत्यधिक भूकंप आने के कारण वहाँ के लोगों को बार-बार प्रकृति के इस प्रकोप का सामना करना पड़ता है। हिंद महासागर के तल में आए भूकंप के कारण ही 26 दिसंबर को समुद्र में भयंकर सुनामी लहरें उत्पन्न हुई थीं। इस सुनामी तूफान ने चार अरब वर्ष पुरानी पृथ्वी में ऐसी हलचल मचा दी कि इंडोनेशिया, मालद्वीप, श्रीलंका, मलेशिया, अंडमान, निकोबार, तमिलनाडु, आंध्र-प्रदेश, केरल आदि सारे तटीय क्षेत्रों पर तबाही का नग्न तांडव हुआ। मछलियाँ पकड़कर आजीविका कमाने वाले कई हजार मछुआरे इस भयंकर सुनामी लहरों की चपेट में आकर जीवन से हाथ धो बैठे।
अंडमान-निकोबार द्वीप समूहों में स्थित वायु-सेना के अड्डे को भी भयंकर क्षति पहुंची तथा सौ से अधिक वायु-सेना के जवान, अधिकारी वर्ग और उनके परिजन काल के ग्रास बन गए। नौ-सेना के जलयान बँधी हुई रस्सियों के टूट जाने के कारण बच तो गए, किंतु चार नागरिक जहाज क्षतिग्रस्त हो गए। पोर्ट ब्लेयर हवाई पट्टी को क्षति पहुँची। उसकी पाँच हजार फीट की पट्टी सुरक्षित होने से राहत पहुंचाने वाले 14 विमान उतारे गए। इस संकट के समय में भारतीय विमानों को श्रीलंका और मालदीव के लोगों की सहायता के लिए भेजा गया। कई मीटर ऊँची सुनामी लहरों ने निकोबार में ए०टी०सी० टावर को भी ध्वस्त कर डाला था, किंतु तत्काल सचल ए०टी०सी० टावर की व्यवस्था कर ली गई थी।
यदि पुराने इतिहास पर दृष्टि डालकर देखा जाए तो पता चलेगा कि यह समुद्री तूफान व बाढ़ कोई नई घटना नहीं है। प्राचीन इराक में आज से लगभग छह हजार वर्ष पूर्व आए समुद्री तूफान और बाढ़ से हुई तबाही के प्रमाण मिलते हैं। बाइबल और कुरान शरीफ में भी विनाशकारी तूफानों का वर्णन मिलता है। ‘श्रीमद्भागवद्पुराण’ में भी प्रलय का उल्लेख मिलता है। उसमें बताया गया है कि जब प्रलय से सृष्टि का विनाश हो रहा था तब मनु भगवान् ने एक नौका पर सवार होकर सभी प्राणियों के एक-एक जोड़े को बचा लिया था।
भले ही यह वर्णन कथा के रूप में कहा गया है, किंतु इससे यह सिद्ध होता है कि समुद्र में तूफान आदिकाल से आते रहे हैं जिनका सामना मनुष्य करता आया है। इतना ही नहीं, भूकंप और समुद्री तूफानों ने पृथ्वी पर अनेक परिवर्तन भी कर दिए हैं। इन्हीं ने नए द्वीपों व टापुओं की रचना भी की है। भारत में प्राचीन द्वारिका समुद्र में डूब गई थी। इसके आज भी प्रमाण मिलते हैं। इसी प्रकार वैज्ञानिक विश्व के अन्य स्थानों की परिवर्तित स्थिति का कारण समुद्री तूफानों व भूकंपों को मानते हैं।
यू०एस० जियोलॉजिकल सर्वे के विशेषज्ञ केन हडनर के अनुसार सुमात्रा द्वीप से 250 किलोमीटर दक्षिण पूर्व में समुद्र-तल के नीचे आए, इस भूकंप की तीव्रता रिक्टर पैमाने पर 9 के लगभग थी। यह भूकंप इतना शक्तिशाली था कि इसने कई छोटे-बड़े द्वीपों को 20-20 मीटर तक अपने स्थान से हिलाकर रख दिया। विद्वानों का यह भी मत है कि यदि भारत अथवा एशिया के क्षेत्र में कहीं भी महासागर की तलहटी में होने वाली भूगर्भीय हलचलों के आकलन की चेतावनी प्रणाली विकसित होती तो इस त्रासदी से होने वाली जान-माल की क्षति को कम किया जा सकता था।
यह बात भी सही है कि प्राकृतिक प्रकोपों को रोक पाना मनुष्य व उसके साधनों के वश में नहीं है, फिर भी यथासंभव सूचना देकर बचने की कुछ व्यवस्था की जा सकती है। 26 दिसंबर, 2004 को सुनामी समुद्री भूकंप भारतीयों के लिए एक नया अनुभव है। भारतीय मौसम विभाग के सामने अन्य महासागरों में उठी सुनामी लहरों से हुई जान-माल की हानि के उदाहरण थे। किंतु भारतीय मौसम विभाग समुद्री भूकंप की सूचना होते हुए भी यह कल्पना तक नहीं कर सका कि सुनामी तरंगों से भारतीय तटीय क्षेत्र की दशा कैसी हो सकती है।
भारत में सुनामी तूफान से हुए विनाश को देखकर विश्वभर के लोगों के हृदय दहल उठे थे। अतः उस समय हम सबका कर्तव्य है कि हम तन, मन और धन से ध्वस्त लोगों के परिवार के साथ खड़े होकर उनकी सहायता करें। इसमें संदेह नहीं कि विश्व के अनेक देशों व संस्थाओं ने सुनामी से पीड़ित लोगों की धन से सहायता की है, किंतु इससे उनके अपनों के जाने का गम तो दूर नहीं किया जा सकता। हमें उनके प्रति सहानुभूति रखनी चाहिए और उनका धैर्य बँधाना चाहिए।

48. राष्ट्रभाषा की समस्या
अथवा
राष्ट्रभाषा हिंदी
भूमिका:
‘राष्ट्र’ शब्द का प्रयोग किसी देश तथा वहाँ बसने वाले लोगों के लिए किया जाता है। प्रत्येक राष्ट्र का अपना स्वतंत्र अस्तित्व होता है। उसमें विभिन्न जातियों एवं धर्मों के लोग रहते हैं। विभिन्न स्थानों अथवा प्रांतों में रहने वाले लोगों की भाषा भी अलग-अलग होती है। इस भिन्नता के साथ-साथ उनमें एकता भी बनी रहती है। पूरे राष्ट्र के शासन का एक केंद्र होता है।
अतः राष्ट्र की एकता को और दृढ़ बनाने के लिए एक ऐसी भाषा की आवश्यकता होती है, जिसका प्रयोग संपूर्ण राष्ट्र में महत्त्वपूर्ण कार्यों के लिए किया जाता है। ऐसी व्यापक भाषा ही राष्ट्रभाषा कहलाती है। भारतवर्ष में अनेक भाषाएँ बोली जाती हैं। भारतवर्ष को यदि भाषाओं का अजायबघर भी कहा जाए तो अतिशयोक्ति न होगी लेकिन एक संपर्क भाषा के बिना आज पूरे राष्ट्र का काम नहीं चल सकता।
1. अंग्रेज़ी-हिंदी का विवाद:
सन 1947 में भारतवर्ष को स्वतंत्रता प्राप्त हुई। जब तक भारत में अंग्रेज़ शासक रहे, तब तक अंग्रेज़ी का बोलबाला था किंतु अंग्रेज़ों के जाने के बाद यह असंभव था कि देश के सारे कार्य अंग्रेज़ी में हों। जब देश के संविधान का निर्माण किया गया तो यह प्रश्न भी उपस्थित हुआ कि राष्ट्र की भाषा कौन-सी होगी ? क्योंकि राष्ट्रभाषा के बिना राष्ट्र के स्वतंत्र अस्तित्व की पहचान नहीं होगी। कुछ लोग अंग्रेज़ी भाषा को ही राष्ट्रभाषा बनाए रखने के पक्ष में थे परंतु अंग्रेज़ी को राष्ट्रभाषा इसलिए घोषित नहीं किया जा सकता था क्योंकि देश में बहुत कम लोग ऐसे थे जो अंग्रेज़ी बोल सकते थे। दूसरे, उनकी भाषा को यहाँ बनाए रखने का तात्पर्य यह था कि हम किसी-न-किसी रूप में उनकी दासता में फंसे रहें।
2. राष्ट्रभाषा के रूप में हिंदी:
हिंदी को राष्ट्रभाषा घोषित करने का प्रमुख तर्क यह है कि हिंदी एक भारतीय भाषा है। दूसरे, जितनी संख्या यहाँ हिंदी बोलने वाले लोगों की थीं, उतनी किसी अन्य प्रांतीय भाषा बोलने वालों की नहीं। तीसरे, हिंदी समझना बहुत आसान है। देश के प्रत्येक अंचल में हिंदी सरलता से समझी जाती है, भले ही इसे बोल न सकें। चौथी बात यह है कि हिंदी भाषा अन्य भारतीय भाषाओं की तुलना में सरल है, इसमें शब्दों का प्रयोग तर्कपूर्ण है। यह भाषा दो-तीन महीनों के अल्प समय में ही सीखी जा सकती है। इन सभी विशेषताओं के कारण भारतीय संविधान सभा ने यह निश्चय किया कि हिंदी को भारत की राष्ट्रभाषा तथा देवनागरी लिपि को राष्ट्रलिपि बनाया जाए।
3. हिंदी के विकास के प्रयत्न:
हिंदी को राष्ट्रभाषा घोषित करने के बाद उसका एकदम प्रयोग करना कठिन था। अतः राजकीय कर्मचारियों को यह सुविधा दी गई थी कि सन 1965 तक केंद्रीय शासन का कार्य व्यावहारिक रूप से अंग्रेज़ी में चलता रहे और पंद्रह वर्षों में हिंदी को पूर्ण समृद्धिशाली बनाने के लिए प्रयत्न किए जाएँ। इस बीच सरकारी कर्मचारी भी हिंदी सीख लें। कर्मचारियों को हिंदी पढ़ने की विशेष सुविधाएँ दी गईं। शिक्षा में हिंदी को अनिवार्य विषय बना दिया गया। शिक्षा मंत्रालय की ओर से हिंदी के पारिभाषिक शब्द-निर्माण का कार्य प्रारंभ हुआ तथा इसी प्रकार की अन्य सुविधाएँ हिंदी को दी गईं ताकि हिंदी, अंग्रेज़ी का स्थान पूर्ण रूप से ग्रहण कर ले। अनेक भाषा-विशेषज्ञों की राय में यदि भारतीय भाषाओं की लिपि को देवनागरी स्वीकार कर लिया जाए तो राष्ट्रीय भावात्मक एकता स्थापित करने में सुविधा होगी। सभी भारतीय एक-दूसरे की भाषा में रचे हुए साहित्य का रसास्वादन कर सकेंगे।
4. हिंदी का विरोध:
आज जहाँ शासन और जनता हिंदी को आगे बढ़ाने और उसका विकास करने के लिए प्रयत्नशील हैं वहाँ ऐसे लोगों की भी कमी नहीं है जो उसको टाँग पकड़कर पीछे घसीटने का प्रयत्न कर रहे हैं। इन लोगों में कुछ ऐसे भी हैं जो हिंदी को संविधान के अनुसार सरकारी भाषा बनाने से तो सहमत हैं किंतु उसे राष्ट्रभाषा के रूप में स्वीकार नहीं करना चाहते। कुछ ऐसे भी हैं जो उर्दू का निर्मूल पक्ष में समर्थन करके राज्य-कार्य में विघ्न डालते रहते हैं। धीरे-धीरे पंजाब, बंगाल और चेन्नई के निवासी भी प्रांतीयता की संकीर्णता में फँसकर अपनी-अपनी भाषाओं की मांग कर रहे हैं परंतु हिंदी ही एक ऐसी भाषा है जिसके द्वारा संपूर्ण भारत को एक सूत्र में पिरोया जा सकता है।
5. हिंदी राष्ट्रभाषा बनने में समर्थ:
निःसंदेह हिंदी एक ऐसी भाषा है जिसमें राष्ट्रभाषा बनने की पूर्ण क्षमता है। इसका समृद्ध साहित्य और इसके प्रतिभा संपन्न साहित्यकार इसे समूचे देश की संपर्क भाषा का दर्जा देते हैं किंतु आज हमारे सामने सबसे बड़ा प्रश्न यह है कि हिंदी का प्रचार-प्रसार कैसे किया जाए ? सर्वप्रथम तो हिंदी भाषा को रोज़गार से जोड़ा जाए। हिंदी सीखने वालों को सरकारी नौकरियों में प्राथमिकता दी जाए। सरकारी कार्यालयों तथा न्यायालयों में केवल हिंदी भाषा का ही प्रयोग होना चाहिए। अहिंदी भाषी क्षेत्रों में हिंदी का अधिकाधिक प्रचार होना चाहिए। वहाँ हिंदी की पत्र-पत्रिकाओं के प्रकाशकों एवं संपादकों को और आर्थिक अनुदान दिया जाए।
उपसंहार:
आज हिंदी के प्रचार-प्रसार में कुछ बाधाएँ अवश्य हैं किंतु दूसरी ओर केंद्रीय सरकार, राज्य सरकारें एवं जनता सभी एकजुट होकर हिंदी के विकास के लिए प्रयत्नशील हैं। सरकार द्वारा अनेक योजनाएँ बनाई गई हैं। उत्तर भारत में अधिकांश राज्यों में सरकारी कामकाज हिंदी में किया जा रहा है। राष्ट्रीयकृत बैंकों ने भी हिंदी में कार्य करना आरंभ कर दिया है। विभिन्न संस्थाओं एवं अकादमियों द्वारा हिंदी लेखकों की श्रेष्ठ पुस्तकों को पुरस्कृत किया जा रहा है। दूरदर्शन और आकाशवाणी द्वारा भी इस दिशा में काफी प्रयास किए जा रहे हैं।
49. परोपकार
भूमिका:
‘परोपकार शब्द दो शब्दों के मेल से बना है-‘पर’ + ‘उपकार’ । इसका अर्थ है-दूसरों की भलाई। अन्य शब्दों में, हम कह सकते हैं कि अपने स्वार्थ का त्याग करके दूसरों को अपना भाग दे देना अथवा अपनी भलाई को छोड़कर दूसरों की भलाई करना ही परोपकार कहलाता है।
कवि वेदव्यास ने 18 पुराणों की रचना की है परंतु उनका सार केवल यही है कि पुण्य के लिए परोपकार किया जाता है और पाप के लिए परपीड़न। गोस्वामी तुलसीदास ने भी इस संदर्भ में लिखा है-
पर हित सरिस धरम नहिं भाई।
पर पीड़ा सम नहिं अधमाई ॥
अर्थात परोपकार के समान कोई दूसरा धर्म नहीं है। जब व्यक्ति ‘स्व’ की सीमा से बाहर निकलकर ‘पर’ के बारे में सोचता है, तब समझिए कि उसमें परोपकार की भावना उत्पन्न होती है।
1. प्रकृति और परोपकार:
प्रकृति दूसरों के लिए अपना सर्वस्व बलिदान कर देती है। पृथ्वी अपने प्राणों का रस निचोड़कर हमारा पेट भरती है। भर्तृहरि ने एक श्लोक में सही कहा है-
पिबन्ति नद्यः स्वमेव नाम्भः स्वयं न खादन्ति फलानि वृक्षाः।
खादन्ति शस्यं न खलु वारिवाहाः परोपकाराय सतां विभूतयः ॥
नदियाँ स्वयं जल नहीं पीतीं और वृक्ष स्वयं फल नहीं खाते, न ही बादल फसलें खाते हैं। इसी प्रकार से सज्जनों का जन्म तो परोपकार के लिए होता है। माता के समान मानी जाने वाली गौ अनादि काल से अपने दुग्ध का दान दूसरों के लिए करती आई है। बाल-वृद्ध सबको ‘समानरूपेण मातृवत्’ पालने वाला यह पशु कितना सम्मान का पात्र बना हुआ है। इसलिए सभी पशुओं में इसका पद सम्मानित है। इसके दूध की एक-एक बूंद दूसरों के निमित्त है, स्वयं के लिए कुछ भी नहीं । निश्चय से यह पशु परोपकारी है।
2. परोपकार एक सात्विक गुण:
दूसरों का हित करना एक सात्विक गुण है। यह गुण ही वस्तुओं को अमरता प्रदान करता है। प्रकृति का जो भी पदार्थ अपने में यह गुण ला सका अर्थात दूसरों के निमित्त अपने को गला सका, वह अमर पद को पा गया। इसके विपरीत जो पदार्थ अपने ही संग्रह में लीन रहा, वह आया और गया, जन्मा और मरा। आज उसका नाम मात्र भी अवशिष्ट नहीं रहा। सूर्य, चंद्र, अग्नि, वायु, इंद्र आदि प्राकृतिक पदार्थ देव-पद के अधिकारी तभी बन पाए, जब इनका एक-एक अंश दूसरों के निमित्त कार्यरूप में आने लगा। इनका सर्वस्व संसार भर के लिए ही काम आया, अपने लिए नहीं।
3. परोपकारी महापुरुष:
प्रकृति के इन देवों की भाँति मानव जाति में भी अनेक देव अवतरित हुए जिनका सर्वस्व दूसरों को अर्पण रहा। अपने तुच्छ स्वार्थ की अवहेलना कर वे अपना सुख, ऐश्वर्य, वैभव सब दूसरे के सुख के लिए अर्पण कर अमर पद को प्राप्त कर गए। उन्होंने सुख-दुःख की तनिक भी परवाह नहीं की। वे किसी के दुःख को सहन नहीं कर सकते थे। पर वेदना उनके लिए सर्वथा असंभव थी। सांसारिक विषमता उन्हें छू तक न सकी थी। अपना बलिदान दूसरों के निमित्त दे देना उन्हें सर्वथा रुचिकर था। यहाँ तक कि अपने शरीर तक की बलि देने वाले हमारे महापुरुष अपने नाम को सदा के लिए अमर कर गए। इस नश्वर संसार में वे कभी नष्ट नहीं हो सकते। राजा शिवि, ऋषि दधीचि , राजा कर्ण आदि अनेक महापुरुष हमारी इस भारत-भूमि पर हुए हैं जिन्होंने परोपकार के लिए अपने-आपको भी बलिदान कर दिया। हमारी संस्कृति तो मानवमात्र की कल्याण-भावना से युक्त है। हमारा आदर्श है ‘बहुजन हिताय बहुजन सुखाय।’ हमारे शास्त्रों का एक ही लक्ष्य था-‘वसुधैव कुटुम्बक’ अर्थात यह पूरा संसार ही मेरा परिवार है। इसीलिए हमारे ऋषि-मुनि सभी की मंगलकामना का उपदेश देते रहे। यही तो है परोपकार का सार तत्त्व।।
4. मानवता का उद्देश्य:
मनुष्य के जीवन की सार्थकता यही है कि वह अपने कल्याण के साथ दूसरों का भी कल्याण करे। उसका कर्तव्य है कि वह स्वयं उठे और दूसरों को भी उठाए । दुखी और दीन की पुकार को सुने, अशक्त और असहाय पर दया करे और भूखों को भोजन दे। मनुष्य के पास यदि धन है तो दुर्बलों की रक्षा करे। ऐसा करके ही वह सच्चा मनुष्य कहलाने का अधिकारी है अन्यथा कुत्ता भी जहाँ-तहाँ मुँह मारकर अपना पेट भर लेता है।
उपसंहार:
निश्चय से यह संसार नश्वर है। यहाँ कोई वस्तु चिरस्थाई नहीं है। जिसका जन्म हुआ है, उसकी मृत्यु भी निश्चित है लेकिन जो व्यक्ति परोपकार करता है; दूसरों के लिए त्याग करता है; उसी का नाम अमर होता है और उसी को आने वाली पीढ़ियाँ याद करती हैं। अस्तु, इस क्षणभंगुर और नश्वर संसार में परोपकार की भावना से ही मानव जाति की सार्थकता को सिद्ध किया जा सकता है। परोपकार ही मानव को स्थिरता, अमरता और चिरस्थायित्व प्रदान करता है। श्री मैथिलीशरण गुप्त जी ने ठीक ही कहा है
यही पशु प्रवृत्ति है कि आप आप ही चरे।
वही मनुष्य है कि जो मनुष्य के लिए मरे ॥

50. किसी भारतीय ऐतिहासिक दर्शनीय स्थान की सैर
(ताजमहल या आगरा)
भूमिका:
देशाटन या पर्यटन के अनेक लाभ हैं। इससे मानव का ज्ञान बढ़ता है और वह अपने आस-पास के वातावरण से परिचित होता है। मनुष्य भ्रमण द्वारा विभिन्न वस्तुओं, स्थानों, जीव-जंतुओं और भवनों को प्रत्यक्ष देखकर अपने अनुभव में वृद्धि करता है। विदेशों में तो पर्यटन एक उद्योग के रूप में विकसित हो चुका है। हमारे देश में भी पर्यटन मंत्रालय की स्थापना हो चुकी है जो कि सफलतापूर्वक कार्य कर रहा है। भारत में प्रतिवर्ष लाखों की संख्या में विदेशों से यात्री आते हैं और भारत के दर्शनीय स्थलों को देखकर प्रसन्न होते हैं। वे भारतवासियों के रहन-सहन, रीति-रिवाज तथा सभ्यता-संस्कृति का भी ज्ञान प्राप्त करते हैं।
1. सुंदर भारत:
भारत एक विशाल देश है। यहाँ प्रकृति का अक्षय भंडार है। संसार में हिमालय क्षेत्र ही एक ऐसा देश है जहाँ बारह महीने ठंड रहती है। यहाँ ऐसे भी स्थान हैं, जहाँ वर्ष के आधे भाग में सरदी और आधे भाग में गर्मी रहती है। पुनः यहाँ पर्वतीय क्षेत्र के साथ-साथ रेगिस्तान अथवा सामुद्रिक क्षेत्र भी हैं। इसी प्रकार से अजंता-अलोरा की गुफाएँ, कोलकाता के अजायबघर, खजुराहो के मंदिर, जयपुर का किला, दिल्ली का कुतुबमीनार, लालकिला और जामा मस्ज़िद तथा आगरा का ताजमहल सभी कुछ दर्शनीय हैं।
2. यात्रा की योजना:
पिछले वर्ष हमारे विद्यालय के विद्यार्थियों में इच्छा उत्पन्न हुई कि किसी दर्शनीय स्थल की यात्रा की जाए। कोई शिमला जाना चाहता था तो कोई जयपुर । किसी ने विचार रखा कि कन्याकुमारी तक की लंबी यात्रा की जाए। अंततः सभी ने मिलकर कक्षा के अध्यापक के समक्ष अपनी इच्छा व्यक्त की। पुनः मुख्याध्यापक की सलाह पर यही निर्णय हुआ कि आगरा की यात्रा की जाए और संसार के महान सुंदर भवन ताजमहल को अपनी आँखों से देखा जाए। हम अपने नगर कुरुक्षेत्र से बस द्वारा दिल्ली पहुंचे। वहाँ से रेलगाड़ी द्वारा हम आगरा पहुंचे। संसार के इस सातवें अजूबे को देखकर हम भौचक्के रह गए।
3. ताजमहल का निर्माण:
ताजमहल की गणना संसार के सात आश्चर्यों में की जाती है। यमुना नदी के किनारे पर स्थित यह एक सुंदर स्मारक है। इसे मुगल सम्राट शाहजहाँ ने अपनी प्रेयसी मुमताज़ की स्मृति में बनवाया था। शाहजहाँ मुमताज़ से अत्यधिक प्रेम करता था। एक बार मुमताज़ बहुत बीमार पड़ गई। उसके जीवन का दीपक बुझने वाला था। वह कृष्ण पक्ष की रात थी। सम्राट के हृदय का चाँद हमेशा के लिए बुझने जा रहा था। देखते-देखते मुमताज़ की आँखें हमेशा के लिए बंद हो गईं। शाहजहाँ अपनी पत्नी की याद में व्याकुल था। वह उसकी याद को हमेशा के लिए अपने मन में बसा लेना चाहता था। अतः उसने मुमताज़ की स्मृति में ताजमहल बनवाया। समूचा भवन श्वेत संगमरमर से बना हुआ है। इस भवन को बनाने में बीस वर्ष लगे थे।
4. ताजमहल का वर्णन:
हम सब अपने शिक्षक के साथ प्रवेश द्वार से अंदर आए। ताजमहल के चारों ओर सुंदर उद्यान हैं। प्रवेश द्वार लाल पत्थरों से बना हुआ है। इन पत्थरों पर कुरान की आयतें खुदी हुई हैं। सामने पानी के उछलते हुए फव्वारे बहुत ही सुंदर दृश्य प्रस्तुत करते हैं। चारों ओर मखमली घास उगी हुई है। सरू के ऊँचे-ऊँचे वृक्ष भी सुंदर लगते हैं। घास का मैदान पार करके हम मुख्य भवन के पास पहुँचे। सामने संगमरमर के चबूतरे पर ताजमहल बना हुआ है। चबूतरे के चारों कोनों में श्वेत संगमरमर की ऊँची मीनारें हैं। मुख्य भवन के मध्य में एक बड़ा-सा हॉल है। इस हॉल के मध्य भाग में मुमताज़ महल और उसके प्रेमी (पति) शाहजहाँ की साथ-साथ कनें हैं। ये कबें भी संगमरमर की बनी हुई हैं।
5. चाँदनी रात में ताजमहल:
ताजमहल को देखने का आनंद तो चाँदनी रात में है। अगले दिन शुक्ल पक्ष की पूर्णिमा थी। अतः हमने अपने शिक्षक को रुकने का आग्रह किया। वे हमारी बात मान गए। हम रात को 10 बजे धर्मशाला से चलकर ताजमहल पहुँचे। क्या सुंदर दृश्य था! लगता था मानो समूचे भवन पर कूची फेरी गई हो। काफी पर्यटक इस समय पहुँचे हुए थे। ऐसा लग रहा था मानो सारा भवन सफेद मखमल की चादर ओढ़कर सो रहा हो।
उपसंहार:
ताजमहल साहित्यकारों की प्रेरणा का स्रोत रहा है। असंख्य कवियों, कलाकारों और चित्रकारों ने इसे देखकर अपनी रचनाएँ लिखीं परंतु मुझे उस रात को ताजमहल देखकर कविवर सुमित्रानंदन पंत की निम्नोक्त पंक्तियाँ याद आ गईं जिनमें कवि ने यह बताने का प्रयास किया है कि ताजमहल मृत्यु की पूजा है जबकि लाखों, करोड़ों लोग भूख और बेरोज़गारी से व्याकुल हैं
हाय! मृत्यु का ऐसा अमर, अपार्थिव पूजन ? जब विषण्ण निर्जीव पड़ा हो जग का जीवन ! स्फटिक सौध में हो श्रृंगार मरण का शोभन! नग्न, क्षुधातुर, वास विहीन रहें जीवित जन!
51. सांप्रदायिक एकता
भूमिका:
जिस समय भारत में सांप्रदायिक एकता थी, उस समय भारत का विश्व पर शक्ति में, शिक्षा में और शांति में एकछत्र आधिपत्य था। विश्व हमारी ओर आदर की दृष्टि से देखता था। हम विश्व के आदिगुरु समझे जाते थे। भारतीय जीवन के आदर्शों से विश्व के लोग शिक्षा ग्रहण करते थे। संपूर्ण राष्ट्र एकता के सूत्र में बँधा हुआ था। भारत विश्व में सर्वोत्तम राष्ट्र समझा जाता था। वेदों में भी भारतवासियों को एकता का संदेश देते हुए कहा गया है-तुम सब मिलकर चलो, संगठित हो जाओ, एक साथ मिलकर बोलो। तुम्हारे मन समान विचार वाले हों। जैसे देवता मिलजुल कर विचार प्रकट करते हैं, उसी प्रकार तुम भी मिलजुल कर रहो।
1. पराधीनता का समय:
परंतु जब से हमारी एकता अनेकता में छिन्न-भिन्न हो गई, तब से हमें ऐसे दुर्दिन देखने पड़े जिनकी आज हम कल्पना भी नहीं कर सकते। हमें पराधीन होना पड़ा। हमें अपने धर्म एवं संस्कृति पर कुठाराघात सहन करना पड़ा। फलस्वरूप. हमारा राष्ट छिन्न-भिन्न हो गया। हमारी सत्रबद्ध सामहिक शक्ति हमेशा के लिए समाप्त हो गई। हम अनेक वर्षों के लिए गुलामी की जंजीरों में बाँध दिए गए। उन जंजीरों से मुक्त होने की कल्पना भी संदिग्ध प्रतीत होने लगी थी। अनेक बलिदानों के पश्चात बहुत कठिनाई से हमें यह आज़ादी मिली है। सहस्रों वर्षों की परतंत्रता के बाद बड़े भाग्य से हमें ये दिन देखने को मिले हैं। इस आत्म-गौरव को प्राप्त करने के लिए हमें कितना मूल्य चुकाना पड़ा, कितनी यातनाएँ सहन करनी पड़ी। हमारी सभ्यता एवं संस्कृति को कितने भयानक थपेड़े सहन करने पड़े और फिर भी हमारी संस्कृति साँस लेती रही। सैकड़ों वर्षों की अनंत साधनाओं के बाद हमें जो अमूल्य स्वतंत्रता मिली है, हम उसे बरबाद करने में लगे हुए हैं। पारस्परिक फूट के कारण हम अपने राष्ट्र को विनाश की ओर ले जा रहे हैं।
2. राष्ट्रीय एकता के लिए खतरा;
आज भारतवर्ष स्वतंत्र है। यहाँ हमारा अपना शासन है किंतु फिर भी सांप्रदायिक एकता अथवा राष्ट्रीय एकता के मार्ग में बाधाएँ आ खड़ी हुई हैं। धर्म, जाति और प्रांत के नाम पर स्थान-स्थान पर लोग आपस में लड़-झगड़ रहे हैं। आज हमारे समाज में कुछ ऐसी जहरीली हवा चल रही है कि सब अपनी-अपनी डफली और अपना-अपना राग अलाप रहे हैं। अपने स्वार्थ में डूबे लोग यह भी नहीं सोचते कि जिसके कारण हमें यह जीवन प्राप्त हुआ है, वह ही नहीं रहेगा अथवा खतरे में पड़ जाएगा तो न हमारी भाषा,बचेगी और न हमारा धर्म तथा न जाति। प्रांतीयता के भाव का यह विष अब शनैः-शनैः फैलता जा रहा है। प्रत्येक व्यक्ति केवल अपने ही प्रांत के विषय में सोचता है। बंगाली बंगाल की, मद्रासी मद्रास की, गुजराती गुजरात की तथा पंजाबी पंजाब की उन्नति चाहता है। किसी को भी न तो समूचे राष्ट्र का ध्यान है और न ही कोई अपना दायित्व समझना चाहता है।
3. भाषागत विभिन्नताएँ:
हमारे देश में भिन्न-भिन्न भाषाएँ हैं। प्रत्येक प्रांत का निवासी अपने प्रांत की भाषा को प्रमुखता देता है। दक्षिणी भारत के कुछ स्थानों पर राष्ट्रगीत इसलिए नहीं गाया जाता क्योंकि वह हिंदी में है। कोई गीता के श्लोकों और रामायण की चौपाइयों को इसलिए मिटा रहा है क्योंकि वे हिंदी में या हिंदी वर्णमाला में हैं। स्वार्थ भावना में इतनी वृद्धि हो चुकी है कि स्वार्थी नागरिक केवल अपने तक ही सोचता है, अपना कल्याण और अपनी उन्नति ही उसका ध्येय है। न उसे देश की चिंता है, न राष्ट्र की। विभिन्न भाषाओं के साथ-साथ भारत में विभिन्न धर्मों के लोगों का वास है। भारत एक धर्मनिरपेक्ष राज्यं है। भारत सरकार किसी भी धर्म को आश्रय नहीं देती परंतु हर धर्म का आदर करती है। लोग केवल अपने-अपने धर्म से प्रेम करते हैं, राष्ट्र से नहीं। धार्मिक संकीर्णता के कारण जहाँ-तहाँ दंगे होते हैं।
4. प्रांतीयता का विष:
पराधीन भारत में सर्वत्र सांप्रदायिक सद्भावना अथवा एकता थी। सभी संप्रदायों के लोगों ने सच्चे मन से, एक-दूसरे के साथ बिना किसी भेदभाव के स्वतंत्रता आंदोलन में भाग लिया और निःस्वार्थ भाव से महान बलिदान देकर भारत को स्वतंत्र करवाया था। उस समय प्रत्येक भारतीय के मुख पर केवल एक ही नारा रहता था-‘हिंदुस्तान हमारा है’ किंतु आज परिस्थितियाँ बदल गई हैं। हम आज नारा लगाते हैं-“पंजाब हमारा है, असम हमारा है।” प्रत्येक व्यक्ति पहले अपनी बात सोचता है फिर प्रदेश की तथा बाद में देश की। यह सोच देश की एकता के लिए घातक है। देश में सांप्रदायिक सद्भावना और राष्ट्रीय एकता के लिए समय-समय पर अनेक उपाय किए गए हैं। अनेक राष्ट्रीय नेताओं ने इसके लिए अपने जीवन का त्याग किया है। महात्मा गांधी ने अपना पूर्ण जीवन ही सांप्रदायिक सद्भावना और एकता के लिए न्योछावर कर दिया है। हमारे संतों ने सदा परस्पर मिलकर रहने का उपदेश दिया है। पंडित जवाहरलाल नेहरू, लालबहादुर शास्त्री आदि नेताओं ने सदैव राष्ट्रीय एकता को दृढ़ बनाने के प्रयास किए।
उपसंहार:
प्रत्येक नागरिक का यह पुनीत कर्तव्य है कि वह सांप्रदायिक सद्भावना को बनाए रखने के लिए हर संभव प्रयास करे। सांप्रदायिक सद्भावना ही किसी राष्ट्र की एकता एवं मज़बूती का आधार होती है। अतः प्रत्येक व्यक्ति का परम कर्तव्य है कि वह संकीर्ण भावनाओं को त्यागकर समूचे समाज अथवा राष्ट्र के लिए सोचे, मनन करे और उसकी समृद्धि के लिए प्रयास करे। यदि समय रहते इस दिशा में उचित कदम नहीं उठाया गया तो सांप्रदायिक भावना के कारण समाज बिखर जाएगा और राष्ट्र की एकता टूट जाएगी। सशक्त एवं सुदृढ़ राष्ट्र के लिए सांप्रदायिक एकता और सद्भावना का होना नितांत आवश्यक है।
52. यदि मैं शिक्षामंत्री बन जाऊँ ?
भूमिका:
‘शिक्षा’ अत्यंत छोटा-सा शब्द है किंतु दिखने में यह जितना छोटा लगता है, इसका अर्थ उतना ही व्यापक है। यह वास्तव में प्रकाश का स्तंभ है। वास्तव में, शिक्षा ही मानव को अपनी अच्छाई या बुराई को समझने के योग्य बनाती है। शिक्षित होकर ही व्यक्ति सही मार्ग पर चलकर विकास को प्राप्त होता है। शिक्षा ही अज्ञान को नष्ट करके ज्ञान का प्रकाश फैलाती है।
अतः शिक्षा मानव जीवन का मूल आधार है। उसके अभाव में मानव, मानव कहलाने योग्य नहीं बन सकता। अतः यह आधारशिला जितनी मज़बूत होगी, उतना ही मानव जाति का विकास होगा।
1. वर्तमान शिक्षा प्रणाली:
आज की शिक्षा प्रणाली का यदि गहराई से अध्ययन किया जाए तो हमारे सामने उसकी अनेक कमियाँ आएँगी। आज की शिक्षा-प्रणाली दोषपूर्ण है। भारत की आधुनिक शिक्षा-प्रणाली पाश्चात्य शिक्षा-प्रणाली से प्रभावित है। भारत में आधुनिक शिक्षा प्रणाली का आरंभ लॉर्ड मैकाले ने किया था। उसका प्रमुख लक्ष्य भारतीयों को अधिक-से-अधिक क्लर्क बनाना था, न कि भारतवासियों का सर्वांगीण विकास करना। आज भारत के स्वतंत्र होने के इतने वर्षों के बाद भी वही शिक्षा-प्रणाली चली आ रही है। इस शिक्षा प्रणाली के द्वारा शिक्षा ग्रहण करने से विद्यार्थी का न तो मानसिक विकास होता है और न ही नैतिक। यह शिक्षा हमारी धार्मिक परंपराओं के भी अनुकूल प्रतीत नहीं होती। यह मात्र किताबी कीड़े उत्पन्न करती है। छात्र वर्ष भर तोते की भाँति किताबें रटते रहते हैं और परीक्षा में तीन घंटे में उस रटे हुए विषय को उगल देते हैं। वे परीक्षा में पास होकर डिग्री प्राप्त करके रोज़गार कार्यालय में नाम दर्ज करवाकर रोज़गार प्राप्त करने की प्रतीक्षा करते रहते हैं।
2. शिक्षा प्रणाली में परिवर्तन:
यदि मैं शिक्षामंत्री होता तो शिक्षा के द्वारा ऐसा खिलवाड़ न करता। यद्यपि कहाँ मैं और कहाँ शिक्षा-मंत्री का पद । यह तो वही बात हुई कहाँ राजा भोज और कहाँ गंगू तेली ? फिर भाग्य पर भरोसा तो करना ही चाहिए। इसलिए भाग्य के भरोसे रहते हुए मैं अभी से यह विचार करने लगा हूँ कि यदि मैं शिक्षा मंत्री बन जाऊँ तो क्या-क्या काम करूँगा? यदि मैं शिक्षामंत्री बन जाऊँ तो सर्वप्रथम शिक्षा-प्रणाली में परिवर्तन करूँगा। मैं शिक्षा-प्रणाली में ऐसे परिवर्तन करने का प्रयास करूँगा कि शिक्षा सैद्धांतिक कम और व्यावहारिक अधिक हो। इसलिए मैं शिक्षा को व्यवसाय से जोड़ने का आदेश देता ताकि विद्यार्थियों को शिक्षा प्राप्त करने पर भी सरकारी नौकरियों के लिए रिश्वत के रूप में अपना शोषण न करवाना पड़े।
शिक्षामंत्री बनने पर मैं प्राथमिक शिक्षा सबके लिए अनिवार्य करने के साथ-साथ प्रत्येक विद्यार्थी के लिए व्यावसायिक शिक्षा की सुविधा उपलब्ध करवाने का प्रयास करता। तकनीकी सुविधाओं को अधिक चुस्त-दुरुस्त बनाता। ऐसी संस्थाओं की संख्या भी बढ़ाता ताकि अधिक छात्र-छात्राएँ तकनीकी शिक्षा प्राप्त करके अपना जीवन सरलता से व्यतीत कर सकें। शिक्षामंत्री बन जाने पर दूसरा प्रमुख कार्य होता है योग्य अध्यापकों को नौकरी प्रदान करना। परिश्रमी, योग्य और कर्तव्यनिष्ठ अध्यापक ही अच्छी शिक्षा प्रदान कर सकते हैं। मैं शिक्षकों के वेतनों में वृद्धि का प्रावधान भी करता ताकि वे समाज में सम्मानपूर्ण जीवन व्यतीत कर सकें और उन्हें ट्यूशन आदि के पीछे न भागना पड़े। जो अध्यापक अपने कर्तव्य का पालन नहीं करते उनके विरुद्ध तुरंत कड़ी कार्रवाई करने की योजना भी बनाता।
3. समान पाठ्यक्रम पर बल:
यदि मैं शिक्षामंत्री बन जाऊँ तो मेरा तीसरा प्रमुख कार्य होगा-समान पाठ्यक्रम लागू करना। मैं सारे देश में समान पाठ्यक्रम की योजना लागू करता ताकि सारे देश में शिक्षा का स्तर एक समान बन सके। त्रिभाषा सूत्रों को भी अनिवार्य रूप से लागू करता ताकि लोग मातृभाषा के साथ राष्ट्रभाषा हिंदी, अंग्रेज़ी आदि भाषाओं का भी अध्ययन कर सकते। मैं यह बात भली-भाँति समझता हूँ कि शिक्षा एवं पाठ्यक्रम की समानता से देश की एकता मज़बूत होगी। यदि मुझे शिक्षामंत्री बनने का अवसर मिल जाए तो मेरी यह भी योजना होगी कि मैं छात्र-छात्राओं के व्यक्तित्व के विकास के लिए पाठ्यपुस्तकों के अध्ययन के साथ-साथ अन्य गतिविधियों में भाग लेना अनिवार्य बनाऊँ और इन गतिविधियों की परीक्षा भी हो जिससे विद्यार्थी उनमें पूर्ण मन से भाग लेना आरंभ कर दें। पुस्तकों का बोझ कम करके छात्र-छात्राओं को अपनी रुचि के खेलों में भाग लेने के अवसर भी प्राप्त हों।।
4. राजनीति मुक्त शिक्षा:
आज हमारे देश में जीवन के प्रत्येक क्षेत्र में राजनीति अपनी जड़ें जमा चुकी है। शिक्षा भी इस रोग से नहीं बच सकी। विद्यालय, महाविद्यालय और विश्वविद्यालय राजनीति के अखाड़े बन चुके हैं। यदि मैं शिक्षामंत्री बन जाऊँ तो मैं शिक्षा के इन मंदिरों को राजनीति से अलग रखने का हर संभव प्रयास करूँगा जिससे विद्या के इन मंदिरों में विद्यार्थी सुचारु रूप से शिक्षा ग्रहण कर सकें और छात्र-छात्राएँ अपनी रुचि के अनुरूप शिक्षा प्राप्त कर सकें। मैं तो चाहता हूँ कि वार्षिक परीक्षा के साथ-साथ आंतरिक मूल्यांकन भी होना चाहिए। परीक्षा में लघूत्तरात्मक और निबंधात्मक प्रश्नों के साथ-साथ वस्तुनिष्ठ प्रश्न भी पूछे जाने चाहिएँ। परीक्षा केवल लिखित नहीं अपितु मौखिक भी होनी चाहिए। शारीरिक स्वास्थ्य भी परीक्षा का भाग होना चाहिए ताकि विद्यार्थी अपने शारीरिक स्वास्थ्य की ओर भी ध्यान देने लगे।
उपसंहार:
अंत में मैं यह कहना चाहूँगा कि आज की शिक्षा दिनों-दिन महँगी होती जा रही है। इस संबंध में मुंशी प्रेमचंद ने भी कहा था, “खर्चीली शिक्षा कभी चरित्रवान व्यक्ति पैदा नहीं कर सकती।” अतः मेरी यह कोशिश रहेगी कि शिक्षा को इतना सस्ता बना दूं कि हर सामान्य व्यक्ति के बच्चे शिक्षा प्राप्त कर सकें। इससे शिक्षा का प्रचार-प्रसार अधिक-से-अधिक लोगों तक हो सकेगा और हम शत-प्रतिशत साक्षरता का लक्ष्य प्राप्त कर सकने में भी सफल हो सकेंगे।

53. मेरी प्रिय पुस्तक-रामचरितमानस
भूमिका:
मेरी प्रिय पुस्तक ‘रामचरितमानस’ है। इसके कवि लोकनायक महाकवि तुलसीदास हैं। शायद ही कोई हिंदू घर ऐसा हो, जिसमें रामचरितमानस की एक प्रति न हो। बड़े-बड़े महलों से लेकर झोंपड़ी तक में रहने वाले निर्धन भी इस महाकाव्य के प्रति आदर और श्रद्धा रखते हैं। रामचरितमानस का गंभीर अध्ययन करके व्यक्ति एक श्रेष्ठ नागरिक बन सकता है। यह महान ग्रंथ हमें सही अर्थों में मानव बनाता है। यह एक ऐसा रसायन है जिसका सेवन करने से मनुष्य सभी मानसिक और शारीरिक रोगों से मुक्त हो सकता है। यह व्यापक जीवानुभूति का काव्य है। तुलसीदास जी ने जहाँ एक ओर इसमें वैभवशाली रघुवंश का वर्णन किया है, वहाँ दूसरी ओर मुनियों के शांत आश्रम और उनके संयम तथा धर्माचरण का भी वर्णन किया है। इसमें कुल सात कांड हैं। इसका मूल आधार ‘वाल्मीकि रामायण’ है।
1. तत्कालीन परिस्थितियों का वर्णन:
तुलसीदास के रामराज के वर्णन में उच्च आदर्शों का वर्णन है तो कलिकाल के वर्णन में तत्कालीन मर्यादाविहीन सामाजिक स्थिति का भी। उन्होंने विश्वामित्र, वशिष्ठ जैसे विद्वान, सदाचारी और तपस्वी गुरुओं के वर्णन के साथ-साथ गाने-बजाने वाले और दूसरों का धन हरने वाले पंडितों का भी वर्णन किया है। उन्होंने सौभाग्यशाली पतिव्रता स्त्रियों के साज-शृंगार के साथ-साथ विधवाओं की दुर्दशा का भी वर्णन किया है। इसी प्रकार, उन्होंने धन लोभी गुरु, गुरु अपमान-रत शिष्य, उदर-पूर्ति की साधिका विद्या, निम्न जातियों के ज्ञानी बनने के ढोंग और ब्राह्मणों के आचरण का भी स्वाभाविक चित्रण किया है। उन्होंने अकाल और अन्नाभाव का जो वर्णन किया है, वह आज भी कितना स्वाभाविक है। रामायण में जहाँ राम, सीता और भरत जैसे आदर्श पात्र हैं, वहाँ रावण, शूर्पनखा, खर-दूषण जैसे पतित पात्र भी हैं। इस प्रकार, हम देखते हैं कि तुलसीदास जी ने जीवन और जगत के प्रत्येक वर्ग का स्वाभाविक वर्णन किया है। रामचरितमानस में लोकमंगल की भावना और उच्च आदर्शों की परिकल्पना की गई है। तुलसीदास जी एक ओर मोह-माया से ऊपर और रामभक्ति में रत हैं तो दूसरी ओर दूसरों के दुःख से दुखी और लोकमंगल के आकांक्षी भी हैं। उन्होंने रामचरितमानस के प्रारंभ में ही अपना उद्देश्य लोकहित घोषित करते हुए लिखा है
कीरति भनिति भूति भलि सोई।
सुरसरि सम सब कर हित होई।।
2. लोक-कल्याण की भावना:
लोक-कल्याण की भावना ही इस ग्रंथ का प्रेरणास्रोत है। राम उस वंश में पैदा हुए जो पूर्ण मर्यादित, धर्मशील, सत्प्रतिज्ञ, बल-विद्या संपन्न और वैभवशाली है। जब विश्वामित्र यज्ञ की रक्षा के लिए राजा दशरथ से राम-लक्ष्मण को माँगते हैं तो राजा दशरथ उन्हें महर्षि विश्वामित्र के साथ भेज देते हैं। राम-वनवास के समय तो जैसे उनकी सत्यनिष्ठा निखर उठती है। यद्यपि वात्सल्य भाव से उनका मन द्रवित हो उठा तथापि राम को वनवास देने और भरत को राजा बनाने के निश्चय पर वे अटल रहे। राम का अवतार मर्यादा की स्थापना के लिए हुआ है। वे अंत तक मर्यादा की स्थापना में लगे रहे। उन्होंने धर्माचरण की रक्षा की। रामचरितमानस में राम जैसे आज्ञापालक पुत्र, राजा दशरथ जैसे दृढ़प्रतिज्ञ एवं सत्यनिष्ठ पिता, सीता जैसी सती और पतिपरायणा पत्नी, भरत और लक्ष्मण जैसे आज्ञाकारी एवं निष्ठावान भाई का बड़ा ही सुंदर चित्रण किया गया है। मर्यादाविहीन एवं पाप-परायण समाज के सामने श्रद्धा-भक्ति पूरित काव्य की रचना करके महाकवि तुलसीदास ने समाज को पतन के गर्त में गिरने से बचाया।
3. रामकथा के चार वक्ता:
रामकथा के चार वक्ता याज्ञवल्क्य, शंकर, काकभुशुंडि तथा स्वयं कवि (तुलसीदास) हैं और क्रमशः भारद्वाज, पार्वती, गरुड़ तथा पाठक चार ही श्रोता हैं। याज्ञवल्क्य प्रयाग में, शंकर कैलाश पर्वत पर तथा काकभुशुंडि नीलगिरि पर रहते हैं। ये तीनों भारत के उत्तर, दक्षिण और मध्य भाग हैं। इस कथा में मानो संपूर्ण राष्ट्र की भौगोलिक, सांस्कृतिक और आध्यात्मिक कथा को ही गूंथ दिया गया है। राम ने अयोध्या से रामेश्वरम तक की पद-यात्रा की। उन्होंने वन्य जातियों का संगठन कर आततायियों का दमन किया। अंत में रावण जैसे निशाचर को मारकर चारों ओर सुव्यवस्था की स्थापना की। इसमें भारत की एकता, शांति और व्यवस्था की भावना का ही समावेश था।
4. समन्वयवादी महाकाव्य:
इस काव्य में अपूर्व समन्वय दिखाई पड़ता है। निर्गुण और सगुण तथा ज्ञान और भक्ति के विरोध में बड़ा विवेकपूर्ण सामंजस्य स्थापित किया गया है। उत्तर कांड में जहाँ ब्रह्मज्ञान को कष्टसाध्य और अनेक विघ्नों से युक्त बताया गया है, वहीं भक्ति को मणिदीप कहा गया है। शैवों और वैष्णवों के विरोध को शांत करने के लिए राम को शिवभक्त बताया गया है।
उपसंहार:
रामचरितमानस में रामभक्ति की ऐसी पावन गंगा बहाई गई है, जिसमें नहाकर कोई भी व्यक्ति शांति और मोक्ष प्राप्त कर सकता है। इसलिए रामचरितमानस मेरी सबसे प्रिय पुस्तक है। हिंदी साहित्य में यही एकमात्र रचना है जिस पर सर्वाधिक टीकाएँ लिखी गई हैं। इसका हिंदू धर्म में वही स्थान है जो ईसाई धर्म में बाइबल का और मुस्लिम धर्म में कुरान शरीफ का है लेकिन यह केवल धार्मिक पुस्तक ही नहीं, हिंदी साहित्य का सर्वश्रेष्ठ महाकाव्य भी है।
54. युवा शक्ति और समाज-निर्माण
भूमिका:
यौवन काल में व्यक्ति के अंदर शक्ति का अथाह सागर ठाठे मारता है। इस काल में व्यक्ति में अनेक इच्छाएँ और अभिलाषाएँ तरंगित होती रहती हैं। इसलिए नवयुवकों की शक्ति पर किसी प्रकार का अंकुश लगाना अत्यधिक कठिन होता है। युवा शक्ति असीम शक्ति का भंडार है। यौवन काल में युवकों में दुर्दमनीय शक्ति होती है। यदि युवकों की शक्ति को सही दिशा न दी जाए तो वह समाज और राष्ट्र के लिए घातक भी सिद्ध हो सकती है। अतः यह आवश्यक है कि युवकों को समाज कल्याण के कार्यों में लगाया जाए और उनकी असीम शक्ति को एक नई दिशा दी जाए।
1. शक्ति का भंडार युवा शक्ति:
विद्यार्थी समाज को बदलना चाहता है। उसके अंदर रचनात्मक कार्यों की असीम शक्ति है। वह सभी सपनों को सच्चाई में बदल सकता है। युवा शक्ति राष्ट्र की रीढ़ की हड्डी है। वह देश की संपत्ति है। इतिहास साक्षी है कि संसार में जहाँ कहीं भी क्रांति हुई है, युवा शक्ति ने उसमें महत्त्वपूर्ण भूमिका निभाई है। आज का विद्यार्थी ही कल का नेता है तथा आगे चलकर उसे ही देश का नेतृत्व करना है।
2. रूढ़ियों और कुरीतियों के उन्मूलन में युवकों का योगदान:
आज विज्ञान की उन्नति ने मानव को चाँद पर पहुँचा दिया है, फिर भी हमारा भारतीय समाज कुरीतियों और रूढ़ियों से ग्रसित है। दहेज प्रथा, छुआछूत, जाति प्रथा, वर्ग भेद, वर्ण व्यवस्था, सती प्रथा, बाल-विवाह आदि जैसी अनेक कुरीतियाँ हमारे समाज के लिए कलंक बन गई हैं। इसी प्रकार के अनेक अंधविश्वास हमारे समाज की जड़ों को खोखला कर रहे हैं। इसी कारण, भारत आज इतने वर्षों की स्वतंत्रता के बाद भी पिछड़ा हुआ है। यदि युवा वर्ग समाज में फैली कुरीतियों को दूर करने के लिए अपनी शक्ति का उपयोग करे तो एक स्वस्थ एवं उन्नतशील समाज का निर्माण हो सकता है।
3. आर्थिक क्षेत्र में युवकों का योगदान:
भारत की अधिकतर जनसंख्या गाँवों में रहती है। गाँवों की जनता बहुत पिछड़ी हुई है। आज कोई भी सरकारी कर्मचारी अथवा शिक्षक गाँवों में जाकर काम नहीं करना चाहता। गाँव के लोग खेती के आधुनिक साधन न जानने के कारण उनका प्रयोग नहीं कर सकते। उनका आर्थिक स्तर भी बहुत गिरा हुआ है। अन्न उपजाने वाले किसान को दो वक्त की रोटी मिलनी भी मुश्किल हो जाती है। इसके विपरीत, नगरों में पूँजी कुछ लोगों के हाथों में जमा हो रही है। गरीब और गरीब होता जा रहा है तथा अमीर और अमीर । इसका परिणाम यह हुआ कि देश में गरीबी, भुखमरी तथा बेरोज़गारी फैली हुई है। नवयुवक इस दिशा में काफी योगदान दे सकते हैं। वे गाँवों में जाकर वहाँ के लोगों को शिक्षित कर सकते हैं और खेती के नए-नए तरीकों से उन्हें परिचित करा सकते हैं। इस प्रकार, किसान अपने अनाज की उपज़ भी बढ़ा सकता है।
4. सांस्कृतिक संकट और युवा वर्ग:
आज देश में सांस्कृतिक संकट आ चुका है। हमारा युवा वर्ग पाश्चात्य सभ्यता का अंधानुकरण कर रहा है। हमारे उपयोगी मूल्य लुप्त हो रहे हैं। हमारी राष्ट्रीय एकता भी खतरे में पड़ गई है। विदेशी शक्तियाँ हमें नष्ट करने पर तुली हुई हैं। उग्रवाद अनेक रूपों में उभरकर हमारे सामने आ रहा है। ऐसी स्थिति में युवा वर्ग का दायित्व और भी बढ़ जाता है। यह सभी को विदित है कि पाश्चात्य जीवन-शैली ने भारतीय समाज के लिए संकट उत्पन्न कर दिया है। हमारा युवा वर्ग बिना सोचे-समझे विदेशी खान-पान और पहनावे की ओर आकृष्ट हो रहा है। यह न केवल समाज के लिए अपितु राष्ट्र के लिए भी घातक है। हमारा युवा वर्ग ही ऐसी स्थिति में भारतीय संस्कृति और उसकी धरोहर की रक्षा कर सकता है। विशेषकर, दूरदर्शन के विभिन्न चैनलों के दुष्परिणाम सभी के सामने उजागर हैं।
5. राष्ट्रीय सेवा योजना:
समाज-सेवा एवं निर्माण के लिए स्कूलों एवं कॉलेजों में राष्ट्रीय सेवा योजना के यूनिटों की स्थापना की गई है। ये यूनिट समाज के पिछड़े वर्गों और गाँवों में अनेक प्रकार के रचनात्मक कार्य कर रही हैं। विशेषकर, अवकाश के दिनों में युवक-युवतियाँ गाँवों में जाकर शिविर लगाते हैं। गाँवों में जाकर अनपढ़ों को पढ़ाना, स्वास्थ्य, सफाई और कृषि की जानकारी देना, गाँवों की गलियाँ पक्की बनाना आदि अनेक समाज-कल्याण के काम युवक कर रहे हैं। इसी प्रकार से प्रौढ़ शिक्षा के क्षेत्र में भी युवकों का सहयोग सराहनीय है लेकिन इस दिशा में अभी और बहुत कुछ करना है।
एन०सी०सी० एक अन्य समाज कल्याण का कार्यक्रम है। यदि हम युवक-युवतियों को समाज कल्याण के कामों में लगाना चाहते हैं तो राष्ट्रीय सेवा योजना, एन०सी०सी० आदि के कार्यक्रमों में युवकों को अधिकाधिक भाग लेना होगा। देश के राजनीतिक, सामाजिक, आर्थिक तथा सांस्कृतिक विकास के लिए देश की युवा शक्ति का सहयोग नितांत आवश्यक है। विशेषकर, आतंकवाद हमारे देश में उग्र रूप धारण कर चुका है। विदेशी शक्तियाँ हमें हर प्रकार से कमज़ोर करना चाहती हैं। 13 दिसंबर, 2001 को भारतीय संसद पर उग्रवादियों का आक्रमण उनके इसी लक्ष्य को सिद्ध करता है। इसी प्रकार, देश के अन्य भागों में भी उग्रवादियों की गतिविधियाँ बढ़ती जा रही हैं।
उपसंहार:
युवा शक्ति देश की अमूल्य निधि है, उसकी शक्ति असीम है। देश के नेताओं, विशेषकर, वर्तमान सरकार को इस शक्ति का सोच-समझकर प्रयोग करना होगा। हमारी समायोजना ऐसी होनी चाहिए कि देश के निर्माण और कल्याण के कामों में धुवक-युवतियों को भाग लेने का समुचित मौका मिले। युवकों को भी चाहिए कि वे अपने दायित्वों को समझें और योजनाबद्ध तरीके से स्वस्थ समाज का निर्माण करने में सरकार की सहायता करें।

55. है अंधेरी रात पर दीपक जलाना कब मना है
भूमिका-प्रस्तुत पंक्ति कविवर बच्चन की एक प्रसिद्ध कविता का अंश है। कवि यहाँ स्पष्ट करना चाहता है कि भले ही चारों ओर दुःख, निराशा और अवसाद का वातावरण छाया हुआ हो लेकिन ऐसी स्थिति में भी मानव का कर्तव्य है कि वह निरंतर संघर्ष करे तथा निराशा और दुःख को आशा तथा सुख में परिवर्तित कर दे।
1. प्रकृति का नियम:
प्रकृति का नियम है कि कृष्ण पक्ष के बाद शुक्ल पक्ष आता है। जेठ-वैशाख में भयंकर लू चलती है। चारों ओर तपती हुई धूप और उड़ती हुई धूल मनुष्य के लिए कष्टों और पीड़ाओं को उत्पन्न करती है, फिर आते हैं सावन के काले बादल। मूसलाधार वर्षा समाप्त होते ही शीत अपना प्रकोप फैलाना शुरू कर देती है। पुनः पतझड़ और अंततः वसंत का आगमन होता है। प्रकृति की यह प्रक्रिया निरंतर चलती रहती है। इसी प्रकार से मानव जीवन में आशा-निराशा और सुख-दुःख का क्रम भी चलता रहता है।
2. जीवन रुकना नहीं चलने का नाम है:
डॉ० बच्चन की पद्य-पंक्ति है अंधेरी रात पर दीपक जलाना कब मना है’ मनुष्य में आशा की किरण उत्पन्न करती है। सर्वप्रथम हम विद्यार्थी जीवन को लेते हैं। जो विद्यार्थी परीक्षा में असफल हो जाते हैं, वे निराश होकर बैठ जाते हैं। वे समझते हैं कि जीवन में सब कुछ समाप्त हो गया है; अब तो अंधकार रूपी निराशा ही चारों ओर है। वे यह नहीं सोचते कि रात के बाद ही प्रभात होती है। अगर एक बार परीक्षा में असफल हो गए तो इससे जीवन तो समाप्त नहीं हो जाता। पुनः परीक्षा तो दे सकते हैं। इस बात की मनाही तो नहीं है कि जो अनुत्तीर्ण है वह पुनः परीक्षा नहीं दे सकता। ऐसे विद्यार्थी को पुनः परिश्रम करना चाहिए-परीक्षा देनी चाहिए। उसे सफलता अवश्य मिलेगी।
विद्या चाहने वाले को हमेशा आशावादी बने रहना चाहिए। असफलता जीवन की प्रथम सीढ़ी है। आशा का सहारा लेकर ही मनुष्य को मुसीबतों का सामना करना चाहिए। जीवन रुकने का नहीं, चलने का नाम है। पगडंडी भी मानव को यही उपदेश देती है। यह कहीं भी नहीं रुकती। कोई सड़क या रास्ता ले लो, वह निरंतर चलता जाता है। नदी भी यही कहती है। पर्वतों से फूटकर बहती है; पत्थरों, पहाड़ों और जंगलों को पार करती हुई। वह सागर की ओर दौड़ती चलती है। अंततः वह अपना लक्ष्य प्राप्त करती है। एक कवि ने सही कहा है-
चलता चल रे साथी चलता चल
एक अन्य कविता में कविवर बच्चन ने इसी से मिलते-जुलते भाव प्रकट किए हैं-
अग्नि पथ! अग्नि पथ! अग्नि पथ!
वृक्ष हों भले खड़े, हों घने, हों बड़े,
एक पत्र-छाँह भी माँग मत, माँग मत, माँग मत
अग्नि पथ! अग्नि पथ! अग्नि पथ!
3. प्रयत्न और परिश्रम की महिमा:
अंधेरी रात अर्थात् असफलता के बाद सफलता के लिए पुनः प्रयास आवश्यक है। प्रयत्न और परिश्रम की बड़ी महिमा है। परिश्रम के द्वारा हम पुनः अपना खोया हुआ मान-सम्मान अर्जित कर सकते हैं। परिश्रम के बल पर एक साधारण व्यक्ति भी असाधारण बन जाता है। अब्राहिम लिंकन, नेपोलियन बोनापार्ट, लालबहादुर शास्त्री, डॉ० सर्वपल्ली राधाकृष्णन आदि ऐसे अनेक महान पुरुष हुए हैं जिन्होंने परिश्रम द्वारा महान कार्य कर दिखाए।
राष्ट्रपिता महात्मा गांधी का उदाहरण भी हमारे सामने है जिन्होंने एक लँगोटी पहनकर अपनी लगन और दृढ़ इच्छाशक्ति के बल पर अंग्रेज़ों को भारत से बाहर निकाल दिया। हमारे सामने एक और उदाहरण चाणक्य का है। नंद की सभा में चाणक्य ने प्रतिज्ञा की-“जब तक मैं नंद वंश का उन्मूलन नहीं कर लूँगा, तब तक अपनी चोटी को गाँठ नहीं लगाऊँगा।” इसी चाणक्य ने संपूर्ण नंद वंश को जड़ से उखाड़कर ही साँस ली और चंद्रगुप्त जैसे धीर, वीर और स्वाभिमानी को भारत का सम्राट् बनाया।
उपसंहार:
निश्चय से, इस संसार में जीना आग पर चलने के समान है। जिस प्रकार आग पर चलने के लिए धैर्य और साहस की आवश्यकता है, उसी प्रकार से जीवन में संघर्षों का सामना करने के लिए भी साहस चाहिए। संघर्षों से जूझने वाले का जीवन ही सार्थक है। कविवर गिरिजाकुमार माथुर भी लिखते हैं-
दुविधा-हत साहस है, दिखता है पंथ नहीं
देह सुखी हो पर मन के दुःख का अंत नहीं
दुःख है न चाँद खिला शरद्-रात आने पर
क्या हुआ जो खिला फूल रस वसंत जाने पर
जो न मिला भूल उसे कर तू भविष्य का वरण।
अस्तु, असफलता और पराजय जीवन के साथ-साथ हैं। यही अंधकार है जो मन रूपी सूर्य को ढक लेता है। अतः निराशा रूपी अंधकार में आशा रूपी दीपक जलाना अनिवार्य है। संघर्षों के बाद प्राप्त सफलता का अपना ही आनंद है।
56. ‘बेटी बचाओ, बेटी पढ़ाओ’
भूमिका:
21वीं सदी में ‘बेटी बचाओ, बेटी पढ़ाओ’ नारे की अनुगूंज चारों ओर सुनाई पड़ने लगी। इस नारे की क्या आवश्यकता है जब भारतवर्ष में प्राचीनकाल से नारी की स्तुति ‘यत्र नार्यस्तु पूज्यन्ते रमन्ते तत्र देवता’ कहकर की जाती रही है। यहाँ तक कि असुरों पर विजय प्राप्त करने के लिए भी देवताओं को नारी की शरण में जाना पड़ा था। देवी दुर्गा ने राक्षसों का संहार किया था।
विद्या की देवी सरस्वती, धन की देवी महालक्ष्मी और दुष्टों का नाश करने वाली महाकाली की आराधना आज भी की जाती है। इतना ही नहीं, आधुनिक काल में आजादी की लड़ाई में महिलाओं ने पर्दा प्रथा को त्यागकर देश को स्वतंत्रता दिलवाने में बढ़-चढ़कर भाग लिया। कविवर पंत ने नारी को ‘देवी माँ’, ‘सहचरी’, ‘सखी’, ‘प्राण’ तक कहकर सम्बोधित किया है। आज नारी चपरासी से लेकर प्रधानमंत्री के पद तक विराजमान है। पुलिस, सुरक्षा बल व सेना तक में भी उच्च पदों पर नियुक्त है। कोई भी ऐसा क्षेत्र नहीं जहाँ उसने अपनी प्रबल कर्मठता का परिचय नहीं दिया। वह हर पद पर पुरुषों से अधिक ईमानदारी से काम करती है, यह सत्य भी किसी से छिपा नहीं है। वह माँ बनकर सृष्टि की रचना करने जैसा पवित्र काम करती है। पत्नी और बहन बनकर अपने सामाजिक दायित्व को निभाती है। आज अपने महान सहयोग से देश के विकास में बराबर की सहभागी बन गई है। फिर उसे हीन-भाव से क्यों देखा जाता है। वे कौन-से कारण हैं जिनके रहते बेटी को गर्भ में ही मार दिया जाता है। आज इन कारणों पर विचार करने की आवश्यकता है।
1. चुनौतीपूर्ण परवरिश:
यदि ध्यान से देखा जाए तो आजकल माता-पिता के लिए बेटी की परवरिश करना चुनौतीपूर्ण होता जा रहा है, क्योंकि यौन अपराधों की संख्या बढ़ती जा रही है। माता-पिता की सोच बनती जा रही है कि यदि हम बेटी की इज्जत की रक्षा न कर पाए तो बेटी को जन्म देने का क्या फायदा होगा। माता-पिता की यही सोच कन्या भ्रूण हत्या का प्रमुख कारण है। इसके साथ-साथ दहेज प्रथा के कारण भी लोग बेटी को आर्थिक बोझ समझते हैं। बेटी का बाप बनना अच्छा नहीं समझा जाता है। बेटे वंश चलाते हैं, बेटी नहीं। आज यौन-अपराध व बलात्कार की घटनाएँ भी बढ़ती जा रही हैं।
इसके अतिरिक्त बेटी को पराया धन कहकर उसकी तौहीन की जाती है। इन सभी कारणों से बेटी को जन्म से पहले ही मार दिया जाता है। यदि समस्या की गहराई में झांका जाए तो इसमें बेटी कहाँ दोषी है ? दोषी तो समाज या उसकी संकीर्ण सोच है। आज बेटियों की कमी के कारण अनेक नई-नई समस्याएँ उत्पन्न हो रही हैं। स्त्री-पुरुष संख्या का संतुलन बिगड़ रहा है। यदि बेटियाँ नहीं होंगी तो बहुएँ कहाँ से आएंगी। आज आवश्यकता है, बेटियों को बेटों के समान समझने की। उन्हें जीवन में आगे बढ़ने के अवसर प्रदान करने की। समाज के अपराध बोध को दूर करने की। दहेज जैसी कुप्रथा को समाप्त किया जाना चाहिए। कन्या भ्रूण हत्या अपनी कब्र खोदने के समान है।
‘इनकी आहों को रोक न पाएंगे हम
अपने किए पर पछताएंगे हम।’
2. बेटी की सुरक्षा के लिए प्रयत्न:
इस दिशा में सरकार ने अब अनेक कदम उठाएँ हैं ताकि बेटियों को बचाया जा सके और जिन कारणों से बेटियों को बोझ समझकर जन्म से पहले ही मार दिया जाता है, उन्हें दूर किया जा सके। कन्या भ्रूण हत्या पर पूर्णतः प्रतिबंध लगा दिए गए हैं। सरकार की इन योजनाओं में ‘बेटी धन’ योजना प्रमुख है। इसमें बेटी की शिक्षा व विवाह में आर्थिक सहायता की जाती है।
3. बेटी बचाओ, बेटी पढ़ाओ का नारा:
‘बेटी बचाओ, बेटी पढ़ाओ’ नारे के दूसरे भाग ‘बेटी पढ़ाओ’ पर भी विचार करना जरूरी है। ‘बेटी पढ़ाओ’ का सम्बन्ध नारी-शिक्षा से है। नारी हो या पुरुष शिक्षा सबके लिए अनिवार्य है किन्तु बेटी-जीवन के सम्बन्ध में शिक्षा का महत्त्व इसलिए भी बढ़ जाता है क्योंकि यदि बेटी शिक्षित होकर स्वावलम्बी बन जाती है तो वह माता-पिता पर बोझ न बनकर उनका बेटों के समान सहारा बन सकती है। बेटी बड़ी होकर देश की भावी पीढ़ी को योग्य बनाने के कार्य में उचित मार्ग-दर्शन कर सकती है।
बच्चे सबसे अधिक माताओं के सम्पर्क में रहते हैं। माता के व्यवहार का प्रभाव बच्चों के मन पर सबसे अधिक पड़ता है। ऐसी स्थिति में बेटियों का पढ़ना अति-आवश्यक है। आज की शिक्षित बेटी कल की शिक्षित माँ होगी जो देश और समाज के उत्थान में सहायक बन सकती है। इसीलिए बेटियों का सुशिक्षित होना अनिवार्य है। शिक्षित व्यक्ति ही अपना हित-अहित, लाभ-हानि भली-भाँति समझ सकता है। शिक्षित बेटियाँ ही अपने विकसित मन-मस्तिष्क से घर की व्यवस्था सुचारु रूप से चला सकती हैं तथा घर-परिवार के लिए आर्थिक उपार्जन भी कर सकती हैं। बेटियाँ पढ़-लिखकर योग्य बनकर आधुनिक देश-काल के अनुरूप उचित धारणाओं, संस्कारों और प्रथाओं का विकास करके कुप्रथाओं व कुरीतियों को मिटाकर एक स्वस्थ एवं उन्नत समाज का निर्माण कर सकती हैं। बेटी पढ़ाओ की दिशा में आज समाज, देश व सरकार प्रयत्नशील हैं।
उपसंहार:
आज बेटी बचाने की आवश्यकता के साथ-साथ बेटियों को शिक्षित, विवेकी, आत्मनिर्भर, आत्मविश्वासी बनाने की भी आवश्यकता है। इसी से बेटियों व नारियों संबंधी अनेक समस्याओं का समाधान सम्भव है। तभी, वे बराबरी और सम्मान के साथ जीवन व्यतीत कर सकेंगी। तंब बेटी बचाओ जैसे नारों की आवश्यकता नहीं रहेगी।

57. भूकंप : एक प्राकृतिक आपदा
भूमिका:
मानव आदि युग से प्रकृति के साहचर्य में रहता आया है। प्रकृति के प्रांगण में मानव को कभी माँ की गोद का सुख मिलता है तो कभी वही प्रकृति उसके जीवन में संकट बनकर भी आती है। बसंत की सुहावनी हवा के स्पर्श से जहाँ मानव पुलकित हो उठता है तो वहीं उसे ग्रीष्म ऋतु की जला देने वाली गर्म हवाओं का सामना भी करना पड़ता है। इसी प्रकार मनुष्य को तेज आँधियों, अतिवृष्टि, बाढ़, भूकंप आदि प्राकृतिक आपदाओं का सामना भी करना पड़ता है।
1. भूकंप का अर्थ:
‘भूकंप’ का अर्थ है भू का काँप उठना अर्थात् पृथ्वी का डांवाडोल होकर अपनी धुरी से हिलकर और फटकर अपने ऊपर विद्यमान जड़ और चेतन प्रत्येक प्राणी और पदार्थ को विनाश की चपेट में ले लेना तथा सर्वनाश का दृश्य उपस्थित कर देना। जापान में तो अकसर भूकंप आते रहते हैं जिनसे विनाश के दृश्य उपस्थित होते हैं। यही कारण है कि वहाँ लकड़ी के घर बनाए जाते हैं। भारतवर्ष में भी भूकंप के कारण अनेक बार विनाश के दृश्य उपस्थित हुए हैं। पूर्वजों की जुबानी सुना है कि भारत के कोटा नामक (पश्चिम सीमा प्रांत, अब पाकिस्तान में स्थित एक नगर) स्थान पर विनाशकारी भूकंप आया। यह भूकंप इतनी तीव्र गति से आया था कि नगर तथा आस-पास के क्षेत्रों के हजारों घर-परिवारों का नाम तक भी बाकी नहीं रहा था।
2. भूकंप से ग्रस्त क्षेत्र:
विगत वर्षों में गढ़वाल, महाराष्ट्र और गुजरात के कुछ क्षेत्रों में विनाशकारी भूकंप आया था जिससे वहाँ का जन-जीवन तहस-नहस हो गया था। पहले गढ़वाल के क्षेत्र में भूकंप के तेज झटके महसूस किए गए थे। वह एक पहाड़ी क्षेत्र है, जहाँ भूकंप के झटकों के कारण पहाड़ियाँ खिसक गई थीं, उन पर बने मकान भी नष्ट हो गए थे। हजारों लोगों की जानें गई . थीं। वहाँ की विनाशलीला से विश्व भर के लोगों के दिल दहल उठे थे। उस विनाशलीला को देखकर मन में विचार उठते हैं कि प्रकृति की लीला भी कितनी अजीब है। वह मनुष्य को बच्चों की भाँति अपनी गोद में खिलाती हुई एकाएक पूतना का रूप धारण कर लेती है। वह मनुष्य के घरों को बच्चों के द्वारा कच्ची मिट्टी के बनाए गए घरौंदों की भाँति तोड़कर बिखेर देती है और मनुष्यों को मिट्टी के खिलौनों की भाँति कुचल डालती है। गढ़वाल के क्षेत्र में भूकंप के कारण वहाँ का जन-जीवन बिखर गया था। कुछ समय के लिए तो वहाँ का क्षेत्र भारतवर्ष के अन्य क्षेत्रों से कट-सा गया था।
3. भूकंप का तांडव नाच:
इसी प्रकार महाराष्ट्र के विभिन्न क्षेत्रों में आए भूकंप के समाचार मिले। यह भूकंप इतना भयंकर और विशाल था कि धरती में जगह-जगह दरारें पड़ गईं। हजारों लोगों की जानें चली गईं। चारों ओर त्राहि-त्राहि मच गई। सरकार की ओर से पहुंचाई जाने वाली सहायता के अतिरिक्त अनेक सामाजिक संस्थाओं और विश्व के अनेक देशों ने भी संकट की इस घड़ी में वहाँ के लोगों की हर प्रकार से सहायता की, किंतु उनके अपनों के जाने के दुःख को कम न कर सके।
धीरे-धीरे समय बीतता गया और समय ने वहाँ के लोगों के घाव भर दिए। वहाँ का जीवन सामान्य हुआ ही था कि 26 जनवरी, 2003 को प्रातः आठ बजे गुजरात में विनाशकारी भूकंप ने फिर विनाश का तांडव नृत्य कर डाला। वहाँ रहने वाले लाखों लोग भवनों के मलबे के नीचे दब गए थे। मकानों के मलबे के नीचे दबे हुए लोगों को निकालने का काम कई दिनों तक चलता रहा। कई लोग तो 36 घंटों के बाद भी जीवित निकाले गए थे। वहाँ भूकंप के झटके कई दिनों तक अनुभव किए गए थे। इस प्राकृतिक प्रकोप की घटना से विश्वभर के लोगों के दिल दहल उठे थे। कई दिनों तक चारों ओर रुदन की आवाजें सुनाई देती रहीं। अनेक स्वयंसेवी संस्थाओं के सदस्य अपने साधनों के अनुरूप समान रूप से सहानुभूति और सहृदयता दिखा रहे थे तथा पीड़ितों को राहत पहुंचा रहे थे। यह भूकंप कितना भयानक था इसका अनुमान वहाँ पर हुए विनाशं से लगाया जा सकता है। वहाँ के लोगों ने बहुत हिम्मत से काम लिया और अपना कारोबार फिर जमाने में जुट गए।
लोग अभी प्रकृति की भयंकर आपदा से उभर ही रहे थे कि 8 अक्तूबर, 2005 को कश्मीर और उससे लगते पाकिस्तान के क्षेत्र में भयंकर भूकंप आया। संपूर्ण क्षेत्र की धरती काँप उठी थी। पर्वतीय क्षेत्र होने के कारण पहाड़ों पर बसे हुए गाँव-के-गाँव तहस-नहस हो गए। साथ ही ठंड सर्दी के प्रकोप ने वहाँ के लोगों को ओर भी मुसीबत में डाल दिया। कड़कती सर्दी में वहाँ के लोगों को खुले मैदानों में रहना पड़ा। सरकार ने हैलीकाप्टरों व अन्य साधनों से वहाँ के लोगों की सहायता के लिए सामान पहुँचाया। भारतीय क्षेत्र की अपेक्षा पाकिस्तान क्षेत्र में अत्यधिक हानि हुई। लाखों लोगों को जान से हाथ धोने पड़े। प्राणियों को जन्म देने वाली और उनकी सुरक्षा करने वाली प्रकृति माँ ही उनकी जान की दुश्मन बन गई थी। 25 अप्रैल, 2015 को नेपाल में आए भूकंप ने हजारों लोगों की जाने ले ली और अनेक लोगों को बेघर कर दिया। देखते-ही-देखते नेपाल की खूबसूरत राजधानी काठमांडू तहस-नहस हो गई।
प्राकृतिक प्रकोप के कारण पीड़ित मानवता के प्रति हमें सच्ची सहानुभूति रखनी चाहिए और सच्चे मन से हमें उनकी सहायता करनी चाहिए। जिनके प्रियजन चले गए, हमें उनके प्रति सद्व्यवहार एवं सहानुभूति दिखाते हुए उनके दुःख को कम करना चाहिए। उनके साथ खड़े होकर उन्हें धैर्य बँधाना चाहिए। यही उनके लिए सबसे बड़ी सहायता होगी।
उपसंहार:
कितनी अजीब है यह प्रकृति और कैसे अनोखे हैं उसके नियम, यह समझ पाना बहुत कठिन कार्य है। भूकंप के दृश्यों को देखकर आज भी एक सनसनी-सी उत्पन्न हो जाती है। किन्तु प्रकृति की अजीब-अजीब गतिविधियों के साथ-साथ मानव की हिम्मत और साहस की भी प्रशंसा किए बिना नहीं रहा जा सकता कि वह प्रत्येक प्राकृतिक आपदा का सदा ही साहसपूर्वक मुकाबला. करता आया है।
![]()
![]()
![]()
![]()
![]()
![]()
![]()

![]()
![]()
![]()
![]() = \(\frac {50}{20}\) g cm-3
= \(\frac {50}{20}\) g cm-3![]()
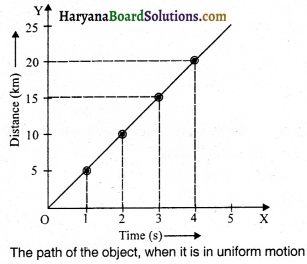
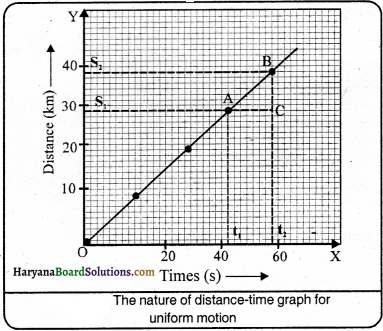
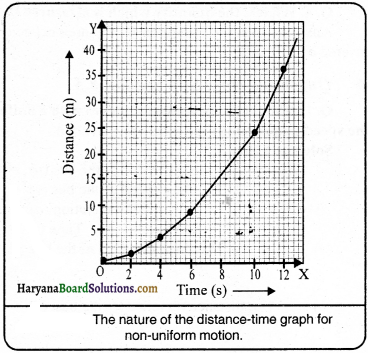
 =\(\frac {x}{30}\) h
=\(\frac {x}{30}\) h