Haryana State Board HBSE 9th Class Maths Solutions Chapter 8 Quadrilaterals Ex 8.1 Textbook Exercise Questions and Answers.
Haryana Board 9th Class Maths Solutions Chapter 8 Quadrilaterals Exercise 8.1
Question 1.
The angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13. Find all the angles of the quadrilateral
Solution :
Ratio of the angles of a quadrilateral = 3 : 5 : 9 : 13.
Sum of ratios= 3 + 5 + 9 + 13 = 30
Sum of angles of a quadrilateral = 360°
Ist angle = \(\frac {3}{30}\) × 360° = 36°
IInd angle = \(\frac {5}{30}\) × 360° = 60°
IIIrd angle = \(\frac {9}{30}\) × 360° = 108°
IVth angle = \(\frac {13}{30}\) × 360° = 156°
Hence, angles of a quadrilateral are 36°, 60°, 108° and 156°.
![]()
Question 2.
If the diagonals of a parallelogram are equal, then show that it is a rectangle.
Solution :
Given: A parallelogram ABCD in which diagonal AC = diagonal BD.
To prove: ABCD is a rectangle.

Proof: In ΔABC and ΔDCB, we have
AB = DC, (Opposite sides of a parallelogram)
BC = BC, (Common)
and AC = BD, (Given)
∴ ΔABC ≅ ΔDCB, (By SSS congruence rule)
⇒ ∠ABC ≅ ∠DCB, (CPCT) …(i)
But AB || CD and BC intersects them.
∠ABC + ∠DCB = 180°,
[∵ Sum of co-interior angles is 180°]
⇒ ∠ABC + ∠ABC = 180°, [Using (i)]
⇒ 2∠ABC = 180°
⇒ ∠ABC = \(\frac {180°}{2}\) = 90°
∴ ∠ABC – ∠DCB = 90°
Thus, ABCD is a parallelogram in which one angle is 90°.
Hence, ABCD is a rectangle. Proved
Question 3.
Show that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus. Solution :
Given : A quadrilateral ABCD in which diagonals AC and BD bisect each other at right angles i.e., AO = OC, OB = OD and ∠AOB = ∠BOC = 90°.
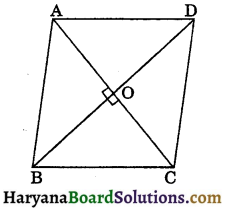
To prove : ABCD is a rhombus.
Proof: In ΔAOB and ΔCOB, we have
AO = OC, (Given)
∠AOB = ∠COB, (Each = 90°)
and BO = BO, (Common)
∴ ΔAOB ≅ ΔCOB,
(By SAS congruence rule)
⇒ AB = BC, (CPCT) …(i)
Similarly, ΔBOC ≅ ΔDOC,
(By SAS congruence rule)
⇒ BC = CD, (CPCT) …(ii)
and ΔCOD ≅ ΔAOD,
(By SAS congruence rule)
⇒ CD = AD, (CPCT) …(iii)
From (i), (ii) and (iii), we get
AB = BC = CD = AD
Hence, ABCD is a rhombus. Proved
![]()
Question 4.
Show that diagonals of a square are equal and bisect each other at right angles.
Solution:
Given: A square ABCD.
To prove : AC = BD, OA = OC, OB = OD and AC ⊥ BD.
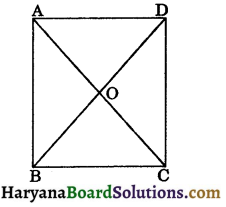
Proof : In ΔABC and ΔDCB, we have
AB = DC, (Sides of square)
∠ABC = ∠DCB, (Each angle of square is 90°)
and BC = BC (Common)
∴ ΔABC ≅ ΔDCB,
(By SAS congruence rule)
⇒ AC = BD, (CPCT)
Since ABCD is a square.
∴ AB || CD and AC intersects them.
⇒ ∠BAC = ∠DCA,
(Alternate interior angles)
⇒ ∠BAO = ∠DCO …….(i)
Again, AB || CD and BD intersects them.
⇒ ∠ABD = ∠CDB,
(Alternate interior angles)
⇒ ∠ABO = ∠CDO …(ii)
Now, in ΔAOB and ΔCOD, we have
∠BAO = ∠DCO, [From (i)]
∠ABO = ∠CDO, [From (ii)]
and AB = CD
ΔAOB ≅ ΔCOD,
(By ASA congruence rule)
⇒ AO = OC and BO = OD, (CPCT) …(iii)
In ΔAOB and ΔCOB, we have
AO = OC (From (iii)]
BO = BO, (Common)
and AB = BC, (Square’s sides)
∴ ΔAOB ≅ ΔCOB, (By SSS congruence rule)
⇒ ∠AOB = ∠COB, (CPCT)
But ∠AOB + ∠COB = 180°, (Linear pair)
⇒ ∠AOB + ∠AOB = 180°, [∵ ∠COB = ∠AOB]
⇒ 2∠AOB = 180°
⇒ ∠AOB = \(\frac {180°}{2}\) = 90°
∴ ∠AOB = ∠COB = 90°
Hence, diagonals of a square are equal and bisect each other at 90°. Proved
Question 5.
Show that if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square.
Solution:
Given : A quadrilateral ABCD in which diagonal AC = diagonal BD, AO = OC, OB = OD and BD ⊥ AC.
To prove: ABCD is a square.
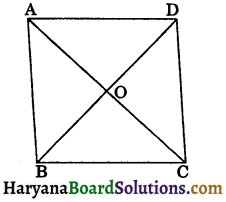
Proof: We know that in a parallelogram diagonals bisect each other.
∴ ABCD is a parallelogram.
(By theorem 8.7)
In ΔAOB and ΔCOB, we have
AO = OC, (Given)
∠AOB = ∠COB, (Each = 90°)
and OB = OB, (Common)
∴ ΔAOB ≅ ΔCOB,
(By SAS congruence rule)
AB = CB, (CPCT)
But, AD = BC and AB = CD, (Opposite sides of a parallelogram)
∴ AB = BC = CD = AD …(i)
Now in ΔDAB and ΔCBA, we have
AD = BC, [From (i)]
AB = AB, (Common)
and BD = AC, (Given)
ΔDAB ≅ ΔCDA,
(By SSS congruence rule)
⇒ ∠DAB = ∠CBA, (CPCT) …(ii)
But, ∠DAB + ∠CBA = 180°,
[∵ Sum of co-interior angles is 180°]
⇒ ∠DAB + ∠DAB = 180°
⇒ 2∠DAB = 180°
⇒ ∠DAB = \(\frac {180°}{2}\) = 90°
Thus, in a parallelogram ABCD, AB = BC = CD = DA and ∠A = 90°.
Hence, ABCD is a square. Proved
![]()
Question 6.
Diagonal AC of a parallelogram ABCD bisects ∠A (see figure 8.31). Show that:
(i) it bisect ∠C also.
(ii) ABCD is a rhombus. [NCERT Exemplar Problems]
Solution :
(i) Since ABCD is a parallelogram.
AD || BC and AC intersects them.
⇒ ∠1 = ∠4 ……(i) (Alternate interior angles)
Again, AB || CD and AC intersects them.
⇒ ∠2 = ∠3 ……(ii) (Alternate interior angles)
But, ∠1 = ∠2, (Given) … (iii)
From (i), (ii) and (iii), we get
∠3 = ∠4
Hence AC bisects ∠C. Proved
(ii) ∠2 = ∠3, [From (ii) …(iv)]
∠4 = ∠3,
(As proved above) …(v)
From, (iv) and (v), we get
∠2 = ∠4
⇒ AB = BC
Thus, adjacent sides of a parallelogram are equal. Hence, ABCD is a rhombus. Proved
Question 7.
ABCD is a rhombus. Show that diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.
Solution:
Given: A rhombus ABCD.
To prove : (i) Diagonal AC bisects ∠A as well as ∠C
i.e.,∠1 = ∠2 and ∠3 = ∠4.
(ii) Diagonal BD bisects ∠B as well as ∠D.
i.e., ∠5 = ∠6 and ∠7 = ∠8

Proof: Since ABCD is a rhombus.
AB = BC, (Rhombus sides)
⇒ ∠2 = ∠4 …(i)
(Angles opposite to equal sides are equal)
Now, AD || BC and AC intersects them.
∴ ∠1 = ∠4 …(ii)
(∵ Alternate interior angles)
From (i) and (ii), we get
∠1 = ∠2 …(iii)
Again, AB || CD and AC intersects them.
∴ ∠2 = ∠3 …(iv)
(∵ Alternate interior angles)
From (i) and (iv), we get
∠3 = ∠4
Thus, ∠1 = ∠2 and ∠3 = ∠4
Hence, AC bisects ∠A as well as ∠C.
Similarly, we can prove that
∠5 = ∠6 and ∠7 = ∠8
Hence, BD bisects ∠B as well as ∠D.
Proved
![]()
Question 8.
ABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that:
(i) ABCD is a square.
(ii) diagonal BD bisects ∠B as well as ∠D.
Solution :
Given: A rectangle ABCD in which diagonal AC bisects ∠A as well as ∠C
i.e., ∠1 = ∠2 and ∠3 = ∠4.
To prove : (i) ABCD is a square.
(ii) diagonal BD bisects ∠B as well as ∠D
i.e., ∠5 = ∠6 and ∠7 = ∠8.
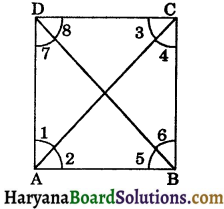
Proof :
(i) Since AC bisects ∠A as well as ∠C.
∠1 = ∠2 …(i)
But, ABCD is a rectangle.
AD || BC and AC intersects them.
∠1 = ∠4, …(ii)
(Alternate interior angles)
From (i) and (ii), we get
∠2 = ∠4
⇒ AB = BC (Sides opposite to equal angles are equal)
Thus, adjacent sides of a rectangle are equal.
Hence, ABCD is a square. Proved
(ii) In ΔABD, AB = AD, (Sides of a square)
⇒ ∠7 = ∠5 …….(iii)
(Angles opposite to equal sides are equal)
But AD || BC and BD intesects them.
∴ ∠7 = ∠6, ……(iv) (Alternate interior angles)
From (iii) and (iv), we get
∠5 = ∠6
In ΔBCD, BC = CD (Sides of a square)
⇒ ∠8 = ∠6 …..(v) (Angles opposite to equal sides are equal]
But, AD || BC and BD intersects them.
⇒ ∠7 = ∠6 …..(vi) (Alternate interior angles)
From (v) and (vi), we get
∠7 = ∠8
Thus, ∠5 = ∠6 and ∠7 = ∠8
Hence, diagonal BD bisects ∠B as well as ∠D.
Hence Proved
Question 9.
In a parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ (see figure 8.84). Show that:
(i) ΔAPD ≅ ΔCQB
(ii) AP = CQ
(iii) ΔAQB ≅ ΔCPD
(iv) AQ = CP
(v) APCQ is a parallelogram.

Solution :
Since ABCD is a parallelogram.
BC || AD and BD intersects them.
∴ ∠ADB = ∠CBD,
(Alternate interior angles)
⇒ ∠ADP = ∠CBQ ……..(i)
In ΔAPD and ΔCQB,
we have DP = BQ, (Given)
∴ ∠ADP = ∠CBQ, [From (i)]
and AD = BC, (Opposite sides of a parallelogram)
∴ ΔAPD ≅ ΔCQB, (By SAS congruence rule)
Hence proved
(ii) ΔAPD ≅ ΔCQB,
⇒ AP = CQ (CPCT) …(ii)
Hence proved
(iii) AB || CD and BD intersects them.
∠ABD = ∠CDB,
(Alternate interior angles)
⇒ ∠ABQ = ∠CDP …(iii)
Now in ΔAQB and ΔCPD, we have
BQ = DP, (Given)
∠ABQ = ∠CDP, [From (iii)]
and AB = CD, (Opposite sides of a parallelogram)
∴ ΔAQB ≅ ΔCPD, (By SAS congruence rule)
Hence proved
(iv) ∵ ΔAQB ≅ ΔCPD
⇒ AQ = CP, (CPCT) …(iv)
Hence proved
(v) From (ii) and (iv), we have
AP = CQ and
AQ = CP,
Hence, APCQ is a parallelogram.
(By theorem 8.3) Hence proved
![]()
Question 10.
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD (see figure 8.36). Show that:
(i) ΔAPB ≅ ΔCQD.
(ii) AP = CQ.
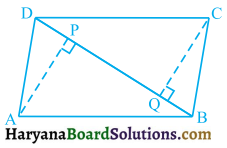
Solution:
(i) Since, ABCD is a parallelogram.
AB || CD and BD intersects them.
∴ ∠ABD = ∠CDB, (Alternate interior angles)
⇒ ∠ABP = ∠CDQ …..(i)
In ΔAPB and ΔCQD, we have
∠APB = ∠CQD, (Each = 90°)
∠ABP = ∠CDQ, [From (i)] and
AB = CD, (Opposite sides of a parallelogram)
∴ ΔAPB ≅ ΔCQD,
(By AAS congruence rule)
(ii) ∵ ΔAPB ≅ ΔCQD
⇒ AP = CQ (CPCT)
Hence proved
Question 11.
In ΔABC and ΔDEF, AB = DE, AB || DE, BC=EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see figure 8.36). Show that
(i) quadrilateral ABED is a parallelogram.
(ii) quadrilateral BEFC is a parallelogram.
(iii) AD || CF and AD = CF.
(iv) Quadrilateral ACFD is a parallelogram
(v) AC = DE
(vi) ΔABC ≅ ΔDEF,
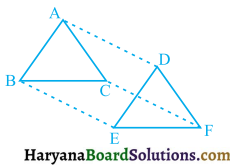
Solution :
(i) In quadrilateral ABED, we have
AB = DE and AB || DE, (Given)
∴ ABCD is a parallelogram.
(By theorem 8.8)
(ii) In quadrilateral BEFC, we have
BC = EF and BC || EF
∴ BEFC is a parallelogram.
(By theorem 8.8)
(iii) Since, ABED is a parallelogram.
[Proved in (i)]
BE = AD and BE || AD …… (1)
(Opposite sides of a parallelogram) and BEFC is a parallelogram.
BE = CF and BE || CF ……(2)
(Opposite sides of a parallelogram)
From (1) and (2), we get
AD = CF and AD || CF ……(3)
(iv) Since, AD = CF and AD || CF,
(As proved above)
∴ ACFD is a parallelogram.
(By theorem 8.8)
(v) ∵ ACFD is a parallelogram.
∴ AC = DF,
(Opposite sides of a parallelogram)
(vi) In ΔABC and ΔDEF, we have
AB = DE (Given)
BC = EF, (Given) and
AC = DF, [From (4)]
∴ ΔABC ≅ ΔDEF, (By SSS congruence rule)
Hence proved
![]()
Question 12.
ABCD is a trapezium in which AB CD and AD = BC (see figure 8.37). Show that:
(i) ∠A = ∠B.
(ii) ∠C = ∠D.
(iii) ΔABC ≅ ΔBAD.
(iv) diagonal AC = diagonal BD.
(Hint : Extend AB and draw a line through C parallel to DA intersecting AB produced at E] OR ABCD is a quadrilateral in which AB || CD and AD = BC. Prove that ∠A = ∠B and ∠C = ∠D. [NCERT Exemplar Problems]

Solution:
Given : ABCD is a trapezium in which AB || CD and AD = BC.
To prove : (i) ∠A = ∠B,
(ii) ∠C = ∠D,
(iii) ΔABC = ΔBAD,
(iv) diagonal AC = diagonal BD.
Construction : Extend AB and draw a line through C parallel to DA intersecting AB propduced at E. Join AC and BD.
Proof: (i) Since AD || CE and AE intersects them.
∴ ∠A + ∠E = 180° ….(i)
[∵ Sum of co-interior angles is 180°]
Now AE || CD (Given)
and AD || CE, (By construction)
∴ AECD is a parallelogram.
⇒ AD = CE
(Opposite sides of parallelogram)
But, AD = BC (Given)
∴ BC = CE
⇒ ∠CBE = ∠CEB …….(ii)
(Angles opposite to equal sides are equal)
Now, ∠B + ∠CBE = 180°, (Linear pair)
⇒ ∠B + ∠CEB = 180°, [Using (ii)]
⇒ ∠B + ∠E = 180° ….(iii)
From (i) and (iii), we get
∠A + ∠E = ∠B + ∠E
⇒ ∠A = ∠B ……..(iv)
Hence proved
(ii) Since AB || CD and AD intersects them.
∠A + ∠D = 180°, ……..(v)
[∵ Sum of co-interior angles is 180°]
Again, AB || CD and BC intersects them.
∠B + ∠C = 180°, ……..(vi)
From (v) and (vi), we have
∠A + ∠D = ∠B + ∠C
⇒ ∠A + ∠D = ∠A + ∠C, [Using (iv)]
⇒ ∠D = ∠C. Hence proved
(iii) In ΔABC and ΔBAD, we have
AB = BA (Common)
∠B = ∠A,
[As proved above in (iv)]
and BC = AD (Given)
∴ ΔABC ≅ ΔBAD, (By SAS congruence rule)
proved ΔABC = ABAD
⇒ AC = BD. (CPCT)
Hence,diagonal AC = diagonal BD.
proved