Haryana State Board HBSE 9th Class Maths Solutions Chapter 6 Lines and Angles Ex 6.2 Textbook Exercise Questions and Answers.
Haryana Board 9th Class Maths Solutions Chapter 6 Lines and Angles Exercise 6.2
Question 1.
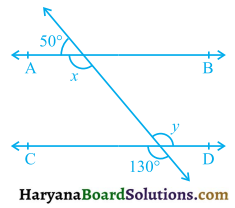
In the figure 6-49, find the values of x and y and then show that AB || CD.
Solution :
x + 50° = 180°,
(Linear pair axiom)
⇒ x = 180° – 50°
⇒ x = 130° …(i)
y = 130° …(ii)
(Vertically opposite angles)
From (i) and (ii), we get
x = y
Thus, a pair of alternate interior angles x and y are equal. Therefore, by theorem 6.3, we have
AB || CD. Hence proved.
![]()
Question 2.
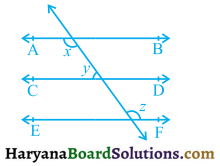
In figure 6.50, if AB || CD, CD || EF and y : z = 3 : 7, find x.
Solution:
Since, CD || EF and transversal line l intersects CD at Q and EF at R.
∠CQR = ∠QRF,
(Alternate interior angles)
⇒ ∠CQR = z
∠PQC + ∠CQR = 180°,
(Linear pair ax,iom)
⇒ y + z = 180°
Now, y : z = 3 : 7
Sum of ratios = 3 + 7 = 10
∴ y = \(\frac {3}{10}\) × 180°
⇒ y = 54°
and z = \(\frac {7}{10}\) × 180°
⇒ z = 126°
Since AB || CD and transversal line l intersects them at P and Q respectively. Therefore,
∠APQ + ∠PQC = 180°,
(Co-interior angles are supplementary)
⇒ x + y = 180°
⇒ x + 54° = 180° [∵ y = 54°]
⇒ x = 180° – 54°
⇒ x = 126°
Hence, x = 126°.
Question 3.
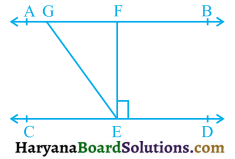
In figure 6.51, if AB || CD, EF ⊥ CD and ∠GED = 126°, find ∠AGE, ∠GEF and ∠FGE.
Solution :
Since, AB || CD and transversal GE cuts them at G and E respectively.
∠AGE = ∠GED,
(Alternate interior angles)
⇒ ∠AGE = 126°
[∵ ∠GED = 126° given]
∠GED = 126°
and ∠FED = 90° (EF ⊥ CD)
Now, ∠GEF = ∠GED – ∠FED
⇒ ∠GEF = 126° – 90°
⇒ ∠GEF = 36°
∠FGE + ∠GED = 180°
(Co-interior angles are supplementary)
⇒ ∠FGE + 126° = 180°
⇒ ∠FGE = 180° – 126°
⇒ ∠FGE = 54°
Hence, ∠AGE = 126°, ∠GEF = 36° and ∠FGE = 54°
![]()
Question 4.
In figure 6.52, if PQ || ST, ∠PQR = 110° and ∠RST = 130°, find ∠QRS.
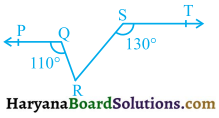
(Hint: Draw a line parallel to ST through point R.)
Solution :
Draw a line EF || ST through point R.
∠TSR + ∠FRS = 180°
(Co-interior angles are supplementary)
⇒ 130° + ∠FRS = 180°,(∵ ∠TSR = 130°)
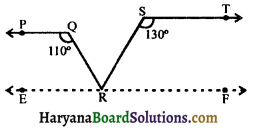
⇒ ∠FRS = 180° – 130°
⇒ ∠FRS = 50°
PQ || ST, (Given)
EF || ST, (By construction)
PQ || EF, (By theorem 6.6)
∠PQR + ∠ERQ = 180°
(Co-interior angles are supplementary)
⇒ 110° + ∠ERQ = 180° (∵ ∠PQR = 110°)
⇒ ∠ERQ = 180° – 110°
⇒ ∠ERQ = 70°
Now, ∠ERQ + ∠QRF = 180°,
(Linear pair axiom)
⇒ ∠ERQ + ∠QRS + ∠FRS = 180°
⇒ 70° + ∠QRS + 50° = 180°
(∵ ∠ERQ = 70° and ∠FRS = 50°)
⇒ 120° + ∠QRS = 180°
⇒ ∠QRS = 180° – 120°
⇒ ∠QRS = 60°
Question 5.
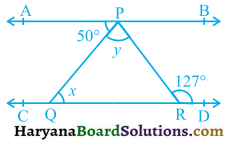
In figure 6.54, if AB || CD, ∠APQ = 50° and ∠PRD = 127°, find x and y.
Solution :
AB || CD and transversal PQ intersects them at P and Q respectively.
∴ ∠PQR = ∠APQ
(Alternate interior angles)
⇒ x = 50°, (∵ ∠APQ = 50°)
Also, AB || CD and transversal PR intersects them at P and R respectively.
∴ ∠APR = ∠PRD
⇒ ∠APQ + ∠QPR = 127°,(∵ ∠PRD = 127°)
⇒ 50° + y = 127°, (∵ ∠APQ = 50°, ∠QPR = y)
⇒ y = 127° – 50°
⇒ y = 77°
Hence, x = 50° and y = 77°.
![]()
Question 6.
In figure 6.55, PQ and RS are two mirrors placed parallel to each other. An incident ray AB strikes the mirror PQ at B, the reflected ray moves along the path BC and strikes the mirror RS at C and again reflects back along CD. Prove that AB || CD.

Solution:
Given: Two mirrors PQ and RS are placed such that PQ || RS. An incident ray AB strikes the mirror PQ at B, the reflected ray moves along the path BC and strikes the mirror RS at C and again reflects back along CD.
To prove: AB || CD.
Construction: Draw normals BM on PQ and CN on RS.
Proof: BM ⊥ PQ and CN ⊥ RS.
⇒ BM || CN
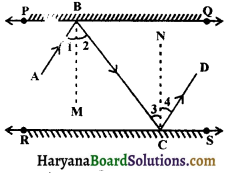
Now, BM || CN and BC is the transversal.
∴ ∠2 = ∠3, (Alternate interior angles) …(i)
∠1 = ∠2 and ∠3 = ∠4,
(By laws of reflection) …(ii)
From (i), we have
2∠2 = 2∠3
⇒ ∠2 + ∠2 = ∠3 + ∠3
⇒ ∠1 + ∠2 = ∠3 + ∠4,
[From (ii), ∠1 = ∠2, ∠3 = ∠4]
⇒ ∠ABC = ∠BCD
Thus, a pair of alternate interior angles ∠ABC and ∠BCD are equal. Therefore, by theorem 6.3, we have AB || CD. Hence proved