Haryana State Board HBSE 9th Class Maths Solutions Chapter 1 Number Systems Ex 1.3 Textbook Exercise Questions and Answers.
Haryana Board 9th Class Maths Solutions Chapter 1 Number Systems Exercise 1.3
Question 1.
Write the following in decimal form and say what kind of decimal expansion each has:
(i) \(\frac {36}{100}\)
(ii) \(\frac {1}{11}\)
(iii) 4\(\frac {1}{8}\)
(iv) \(\frac {3}{13}\)
(v) \(\frac {2}{11}\)
(vi) \(\frac {329}{400}\)
Solution:
(i) By long division, we have

Hence, \(\frac {36}{100}\) = 0.36
Hence, It has terminating decimal expansion.
(ii)
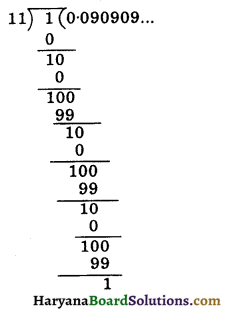
Hence, \(\frac {1}{11}\) = 0.090909 = \(0 . \overline{09}\).
It has non-terminating and repeating decimal expansion.
(iii)

Hence,
4\(\frac {1}{8}\) = 4.125.
It has terminating decimal expansion.
(iv)

Hence, \(\frac {3}{13}\) = 0.2307692307692…
= \(0 \cdot \overline{230769}\)
It has non-terminating repeating decimal expansion.
(v)

Hence,
\(\frac {2}{11}\) = 0.1818… = \(0 \cdot \overline{18}\)
It has non-terminating repeating decimal expansion.
(vi)

Hence, \(\frac {329}{400}\) = 0.8225.
It has terminating decimal expansion.
![]()
Question 2.
You know that \(\frac {1}{7}\) = \(0 \cdot \overline{142857}\). Can you predict what the decimal expansions of \(\frac{2}{7}, \frac{3}{7}, \frac{4}{7}, \frac{5}{7}, \frac{6}{7} \) are, without actually doing the long division ? If so, how ? [Hint : Study the remainders while finding the value of \(\frac {1}{7}\) carefully]
Solution :
Yes, we can predict what the decimal expansions of \(\frac{2}{7}, \frac{3}{7}, \frac{4}{7}, \frac{5}{7}, \frac{6}{7} \) are without actually doing the long division. Now 1 divide by 7

We observe that remainder repeat after six division. So, it has repeating block of six digits. The given rational numbers \(\frac{2}{7}, \frac{3}{7}, \frac{4}{7}, \frac{5}{7}\) and \(\frac {6}{7}\) and will also have repeating block of six digits in the decimal expansions. So, to obtain the decimal expansions of given rational numbers, multiplying \(0 . \overline{142857}\) successively by 2, 3, 4, 5 and 6 as follows:

Question 3.
Express the following in the form \(\frac {p}{q}\), where p and q are integers and q ≠ 0:
(i) \(0 \cdot \overline{6}\)
(ii) \(0.4 \overline{7}\)
(iii) \(0 \cdot \overline{001}\)
Solution:
(i) Let x = \(0 \cdot \overline{6}\) = 0.6666 …….(i)
Multiplying equation (i) by 10, we get
10x = \(6 \cdot \overline{6}\)
Subtracting equation (i) from (ii), we get
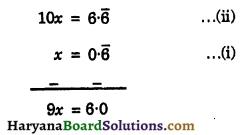
⇒ x = \(\frac{6}{9}=\frac{2}{3}\)
Hence,
\(0 \cdot \overline{6}\) = \(\frac{2}{3}\)
(ii) Let x = \(0.4 \overline{7}\) =0.47777 ……(i)
Since, there is one non-repeating digit after the decimal.
Therefore, multiplying equation (i) by 10. So that this digit shifted before the decimal, we get
10x = \(4 \cdot \overline{7}\) = 4.7777 ………(ii)
Multiplying the equation (ii) by 10, we get
100x = \(47 \cdot \overline{7}\) …….(ii)
Subtracting equation (ii) from (iii), we get
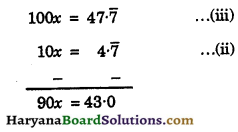
⇒ x = \(\frac {43}{90}\)
Hence,
\(0.4 \overline{7}\) = \(\frac {43}{90}\)
(iii) Let
x = \(0 . \overline{001}\)
= 0.001001001 ………(i)
Multiplying equation (i) by 1000, we get
1000x = \(1 . \overline{001}\) ………(ii)
Subtracting equation (i) from (ii), we get

⇒ x = \(\frac {1}{999}\)
Hence,
\(0 . \overline{001}\) = \(\frac {1}{999}\)
![]()
Question 4.
Express 0.99999….. in the form \(\frac {p}{q}\). Are you surprised by your answer ? With your teacher and classmates discuss why the answer makes sense.
Solution:
Let x = 0.99999… = \(0 . \overline{9}\) …….(i)
Mutiplying equation (i) by 10, we get
10x = 9.99999… = \(9 . \overline{9}\) ……(ii)
Subtracting equation (i) from (ii), we get
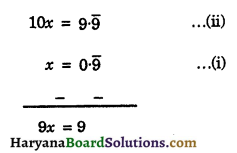
x = \(\frac {9}{9}\) = 1
Hence, 0.99999… = 1
We observe that there is no gap between 1 and 0.99999… and hence they are equal.
Question 5.
What can the maximum number of digits be in the repeating block of digits in the decimal expansion of \(\frac {1}{17}\)? Perform the division to check your answer.
Solution:
By long division method, we have


We observe that the remainder start repeating after 16 divisions.
Hence, \(\frac {1}{17}\) = \(0 . \overline{0588236294117647}\)
![]()
Question 6.
Look at several examples of rational numbers in the form \(\frac {p}{q}\) (q ≠ 0), where p and q are integers with no common factors other than 1 and having terminating decimal representations (expansions). Can you guess what property q must satisfy?
Solution :
Let us consider the various rational numbers be \(\frac{1}{2}, \frac{1}{4}, \frac{1}{8}, \frac{7}{20}, \frac{23}{25}, \frac{12}{125}\) etc.
In all these rational numbers multiplying the denominator by such numbers so that it becomes 10 or power of 10, we get
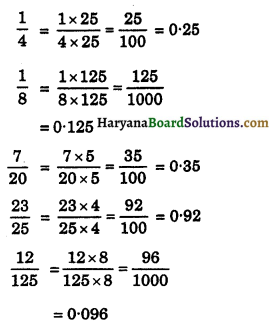
In these cases we observe that to obtain a terminating decimal form of given rational numbers we have to multiplied the denominator of these rational numbers by a suitable integer. But it is possible when denominator of given rational numbers be 2n5m as the prime factor, where m and n are non-negative integers. Thus we have the following property :
If the prime factorization of denominator of given rational numbers is of the form 2n5m, where m, n are non-negative integers, then it can be represented as a terminating decimal.
Question 7.
Write three numbers whose decimal expansions are non-terminating nonrecurring.
Solution :
Three numbers whose decimal expansions are non-terminating non recurring are .01001000100001…, 0.202002000200002… and 0.003000300003…
![]()
Question 8.
Find three different irrational numbers between rational numbers \(\frac {5}{7}\) and \(\frac {9}{11}\)
Solution:
We have,
\(\frac {5}{7}\) = 0.714285714…
\(\frac {9}{11}\) =0.81818181…
Three irrational numbers between
0.714285714… and 0.81818181… are: 0.75075007500075000075…, 0.767076700767000767…, and 0.808008000800008…
Hence, three irrational numbers between \(\frac {5}{7}\) and \(\frac {9}{11}\) are :
0.75075007500075000075…, 0.767076700767000767… and 0.808008000800008…
Question 9.
Classify the following numbers as rational or irrational:
(i) \(\sqrt{23}\)
(ii) \(\sqrt{225}\)
(ii) 0.3796
(iv) 7.478478…
(v) 1.101001000100001…
Solution:
(i) Since 23 is not a perfect square. So \(\sqrt{23}\) is an irrational number.
(ii) \(\sqrt{225}\) = \(\sqrt{3 \times 3 \times 5 \times 5}\)
= 3 × 5
= 15
which is a rational number.
Hence, \(\sqrt{225}\) is a rational number.
(iii) 0.3796 is a terminating decimal expansion, so it is a rational number.
(iv) 7.478478… is non-terminating repeating decimal expansion, so it is a rational number.
(v) 1.101001000100001… is non-terminating non-repeating decimal expansion, so it is an irrational number.