Haryana State Board HBSE 10th Class Maths Notes Chapter 4 Quadratic Equations Notes.
Haryana Board 10th Class Maths Notes Chapter 4 Quadratic Equations
Introduction
You are already familiar with linear equations in one and two variables and their solutions. In chapter 2, you have studied different types of polynomials. Recall that polynomials of degree 2 are called quadratic polynomials. The standard form of quadratic polynomial is ax2 + bx + c, where a, b, c are real numbers and a 1 0. When we equate this polynomial to zero, we get a quadratic equation. Quadratic equations come up when we deal with many real-life situations. In this chapter, we shall study about quadratic equations and solving them by various methods. We shall also discuss some applications of quadratic equations in daily life situations.
1. Equation : An equation is an algebraic expression obtained by equating a polynomial to zero. It has two parts separated by an equal (=) sign. Left part is called LHS (Left Hand Side) and right part is called RHS (Right Hand Side).
For example:
p(x) = x + 5 [Linear Polynomial]
put p(x) = 0
x + 5 = 0 [Linear Equation]
p(y) = 2y2 + 3y + 4 [Quadratic Polynomial]
put p(y) = 0
2y2 + 3y + 4 = 0 [Quadratic Equation]
2. Roots of an equation : A real number a is said to be a root of an equation p(x) = 0, if p(a) = 0.
3. The zeroes of a polynomial p(x) and roots of the respective equation p(x) = 0 are the same.
4. Imaginary roots : If an equation does not have real roots then it is said to be having imaginary roots.
5. \(\text { Speed }=\frac{\text { Distance }}{\text { Time }}\)
6. Distance = Speed × Time
7. \(\text { Time }=\frac{\text { Distance }}{\text { Speed }}\)
![]()
Quadratic Equation
If p(x) is a quadratic polynomial, then p(x) = 0 is called a quadratic equation.
The general form of a quadratic equation is ax2 + bx + c = 0, where a, b, c are real numbers and a ≠ 0. It is also known as the standard form of a quadratic equation.
For example: 2x2 + 5x + 7 = 0. -7x2 + 3x – 4 = 0, x2 + 5x + 6 = 0 are quadratic equations in variable x.
But x2 + \(\frac{3}{x}\) + 4 = 0, x2 + \(2 \sqrt{x}\) – 3 = 0, x2 + \(\frac{1}{x^2}\) + 1 = 0 are not quadratic equations.
Roots of a quadratic equation : Let p(x) = 0 be a quadratie equation, then the zeroes of the polynomial p(x) are called the roots of the equation p(x) = 0.
Thus, α is a root of quadratic equation p(x) = 0 if p(α) = 0, and x = α is the solution of the given equation.
In chapter 2, we studied that quadratic polynomial have at mont two zeroes. It follows from this that a quadratic equation has at most two real roots.
Let us consider some examples.
Solution of a Quadratic Equation by Factorisation Method
Let ax2 + bx + c = 0, a ≠ 0 be a quadratic equation and let quadratic polynomial ax2 + bx + c be expressible as a product of two linear factors, say (mx + n) and (qx + p), where m, n, q, p are real numbers such that m ≠ 0, q ≠ 0.
Then ax2 + bx + c = 0
⇒ (mx + n)(qx + p) = 0
⇒ mx + n = 0 or qx + p = 0
⇒ x = \(-\frac{n}{m}\) or x = \(-\frac{p}{q}\)
Hence, x = \(-\frac{n}{m}\) and x = \(-\frac{p}{q}\) are the possible roots of the quadrntic equation ax2 + bx + c = 0.
Solution of a Quadratic Equation by Completing the Square
It is possible to take a quadratic equation whose left hand side is not a perfect square and change it into one that is, then we solve the resulting equation by extracting root. The technique of solving quadratic equations in this manner is called completing the square. To solve the quadratic equation by the method of completing the square we may use the following steps:
Step I: Consider the quadratic, equation
ax2 + bx + c = 0, a ≠ 0, a b, c ∈R
Step II: Make the coefficient of x unity. If it is not, i.e., we get
\(x^2+\frac{b}{a}+\frac{c}{a}=0\)
Step III: Transposing the constant term \(\frac{c}{a}\) to R.H.S., we get
\(x^2+\frac{b}{a} x=-\frac{c}{a}\)
Step IV: Add(\(\frac{1}{2}\) × coefficient of x)2 on both sides, we get
\(x^2+\frac{b}{a} x+\left(\frac{b}{2 a}\right)^2=\left(\frac{b}{2 a}\right)^2-\frac{c}{a}\)
Step V: Express L.H.S. as the perfect square of a suitable binomial expression and simplify R.H.S. we get
\(\left(x+\frac{b}{2 a}\right)^2=\frac{b^2-4 a c}{4 a^2}\)
Step VI: Take square root of both sides, we get
\(x+\frac{b}{2 a}=\pm \sqrt{\frac{b^2-4 a c}{4 a^2}}\)
Step VII: Obtain the values of shifting the constant term \(\frac{b}{2 a}\) to R.H.S.
![]()
Solution of a Quadratic equation by Quadratic Formula
[Shreedhara Charya’s Rule]
Consider the quadratic equation ax2 + bx + c = 0, a ≠ 0, a, b, c ∈R.
ax2 + bx + c = 0
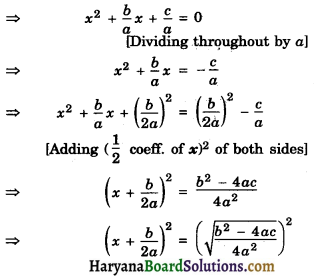
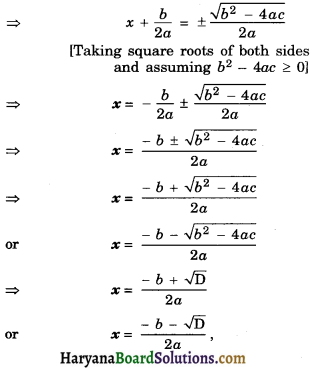
where D = b2 – 4ac and D is called discriminant.
Thus, If D = b2 – 4ac ≥ 0, then the quadratic equation ax2 + bx + 0 = 0 has two real roots α and β given by :

Remarks: This is a formula for the roots of a quadratic equation expressed in terms of the coefficienta a, b, c. This is given by an ancient Indian Mathematician Shreedhara Charya around 1025 A.D. and is known as Shreedhara Charya’s formula for determining the roots of the quadratic equation ax2 + bx + c = 0.
![]()
Nature of Roots
In previous section, you have seen that roots of the equation ax2 + bx + c = 0, a ≠ 0 are given by :
x = \(\frac{-b \pm \sqrt{b^2-4 a c}}{2 a}\)
There are three different cases:
Case I: If b2 – 4ac > 0
In this case, the roots are real and distinct.
These roots are given by
\(x=\frac{-b+\sqrt{b^2-4 a c}}{2 a}\)
and \(x=\frac{-b-\sqrt{b^2-4 a c}}{2 a}\)
Case II: If b2 – 4ac = 0
In this case, the roots are real and equal
Then \(x=\frac{-b \pm \sqrt{0}}{2 a}\)
\(x=-\frac{b}{2 a}\) and x = \(-\frac{b}{2 a}\)
Case III: If b2 – 4ac < 0
In this case there is no real number whose square is b2 – 4ac. Therefore, there are no real roots for the given quadratic equation. Since, b2 – 4ac determines whether the quadratic equation ax2 + bx + c has real roots or not, b2 – 4ac is called the discriminant of this quadratic equation. It is generally denoted by D.
So, a quadratic equation ax2 + bx + c = 0 has:
(1) Two distinct real roots, if b2 – 4ac > 0 i.e, D > 0.
(2) Two equal and real roots (ie., coincident roots) if b2 – 4ac = 0 i.e., D = 0.
(3) No real roots, if b2 – 4ac < 0 i.e, D < 0.