HBSE 10th Class Maths Solutions Chapter 6 Triangles Ex 6.5

Haryana State Board HBSE 10th Class Maths Solutions Chapter 6 Triangles Ex 6.5 Textbook Exercise Questions and Answers.

Haryana Board 10th Class Maths Solutions Chapter 6 Triangles Ex 6.5

Question 1.
Sides of triangles are given below. Determine which of them are right triangles. In case of a right triangle, write the length of its hypotenuse.
(i) 7 cm, 24 cm, 26 cm
(ii) 3 cm, 8 cm, 6 cm
(iii) 50 cm, 80 cm, 100 cm
(iv) 13 cm, 12 cm, 5 cm.
Solution:
(i) The given sides of the triangle are 7 cm, 24 cm, 25 cm,
The longest side = 25cm.
The triangle is right angled if
(the longest side)² = (sum of squares of other two sides) (by Pythagoras theorem)
(25)² = (7)² + (24)²
625 = 49 + 576
625 = 625
The given sides make a right triangle and length of hypotenuse = 25cm.

(ii) The given sides of the triangle are 3cm, 8 cm, 6 cm.
The longest side = 8 cm
(the longest side)² = (sum of the squares of other two sides) (by Pythagoras theorem)
(8)² = (3)² + (6)²
64 = 9 + 36
But 64 ≠ 45
The given sides do not make a right triangle.

(iii) The given sides of the triangle Eire 50 cm, 80 cm, 100 cm.
The longest side = 100 cm,
(the longest side)² = (sum of the squares of other two sides) (by Pythagoras theorem)
(100)² = (50)² + (80)²
10000 = 2500 + 6400
But 10000 ≠ 8900
∴ The given sides do not mEike a right triangle.

(iv) The given sides of the triangle are 13 cm, 12 cm, 5 cm.
The longest side = 13 cm.
(the longest side)² = (sum of the squares of other two sides) (by Pythagoras theorem)
(13)² = (12)² + (5)²
169 = 144 + 25
169 = 169
∴ The given sides make a right triangle and length of hypotenuse = 13 cm.

Question 2.
PQR is a triangle right angled at P and M is a point on QR such that PM ⊥ QR. Show that PM² = QM.MR.

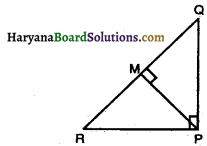
Solution:
Given : A right ∆PQR in which ∠P = 90° and PM ⊥ QR.
To Prove : PM² = QM.MR
Proof : In right ∆QPR, PM ⊥ QR.
∆RPM ~ ∆PQM [By theorem 6.7]
⇒ \(\frac{\mathrm{MR}}{\mathrm{PM}}=\frac{\mathrm{PM}}{\mathrm{QM}}\)
[Corresponding sides of similar triangles sere proportional]
⇒ PM² = QM × MR
Hence Proved.

Question 3.
In given figure, ABD is a triangle right angled at A and AC ⊥ BD. Show that
(i) AB² = BC.BD
(ii) AC² = BC.DC
(iii) AD² = BD.CD

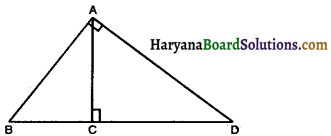
Solution:
Given : A right triangle in which ∠A = 90° sind AC ⊥ BD.
To Prove :
(i) AB² = BC.BD
(ii) AC² = BC.DC
(iii) AD² = BD.CD
Proof :
(i) In right ∆BAC, AC ⊥ BD.
∆BAC ~ ∆BDA [By theorem 6.7]
⇒ \(\frac{\mathrm{AB}}{\mathrm{BD}}=\frac{\mathrm{BC}}{\mathrm{AB}}\)
[Corresponding sides of similar triEingles are proportional]
⇒ AB² = BC.BD
Hence Proved.

(ii) Similsirly, ∆ABC~ ∆DAC [By theorem 6.7]
\(\frac{\mathrm{AC}}{\mathrm{DC}}=\frac{\mathrm{BC}}{\mathrm{AC}}\)
[Corresponding sides of similar triEingles Eire proportional]
⇒ AC² = BC.CD
Hence Proved.

(iii) Similarly, ∆DAC ~ ∆DBA [By theorem 6.7]
\(\frac{\mathrm{CD}}{\mathrm{AD}}=\frac{\mathrm{AD}}{\mathrm{BD}}\)
[Corresponding sides of similar triangles are proportional]
⇒ AD² = BD.CD
Hence Proved.

Question 4.
ABC is an isosceles triangle right angled at C. Prove that AB² = 2AC².
Solution:
Given : ∆ABC is an isosceles triangle right angled at C.
i.e., AC = BC
To Prove : AB² = 2AC²
Proof : In right ∆ACB

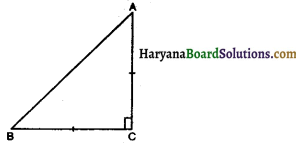
AB² = BC² + AC² [By Pythagoras theorem]
⇒ AB² = AC² + AC² [∵ BC = AC]
⇒ AB² = 2AC².
Hence Proved.

Question 5.
ABC is an isosceles triangle with AC = BC. If AB² = 2AC², prove that ABC is a right triangle.
Solution:
Given: ∆ABC is an isosceles triangle in which AC = BC and AB² = 2AC²
To Prove : ∆ABC is a right triangle.

Proof : In ∆ABC, AC = BC and AB² = 2AC²
⇒ AB² = AC² + AC²
⇒ AB² = BC² + AC² [v AC = BC]
⇒ ABC is right angle triangle.
[By converse of Pythagoras theorem]
Hence Proved.

Question 6.
ABC is an equilateral triangle of side 2a. Find each of its altitudes.
Solution :
Side of equilateral ∆ = 2a.
And AD is its altitude. We know that altitude bisects its corresponding side.
i.e., BD = CD = a

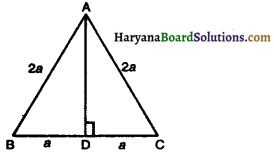
Now right triangle ∆ADC.
AC² = AD² + CD² [By Pythagoras theorem]
⇒ (2a)² = AD² + a²
⇒ 4a² = AD² + a²
⇒ AD² = 4a² – a² = 3a²
⇒ AD = √3a
Hence, Altitude = √3a.

Question 7.
Prove that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
Given : ABCD is a rhombus

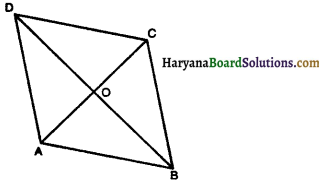
Proof: We know that diagonals of a rhombus bisect each other at right angle
∴ ∠AOB = ∠BOC = ∠COD = ∠AOD = 90° and AO = CO, OD = BO.
In right ∆AOB, AB² = OA² + OB² [By Pythagoras theorem]
AB² = (\(\frac{1}{2}\) AC)² + (\(\frac{1}{2}\) BD)²
[∵ OA = OC and OB = OD]
⇒ AB² = 7 AC² + 7BD²
⇒ 4AB² = AC² + BD² ……………(1)
Similarly, we have
4BC² = AC² + BD² ……………….(2)
4CD² = AC² + BD² ……………….(3)
4AD² = AC² + BD² ……………….(4)
Adding (1), (2), (3) and (4), we get
4(AB² + BC² + CD² + AD²) = 4(AC² + BD²)
⇒ AB² + BC² + CD² + AD² = AC² + BD²
Hence Proved.

Question 8.
In given figure, O is a point in the interior of a triangle ABC, OD ⊥ BC, OE ⊥ AC and OF ⊥ AB. Show that
(i) OA² + OB² + OC² – OD² – OE² – OF² = AF² + BD² + CE²
(ii) AF² + BD² + CE² = AE² + CD² + BF².

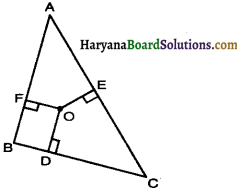
Solution: Given: A ∆ABC in which OD ⊥BC, OE ⊥ AC, OF ⊥ AB.
To Prove :
(i) OA² + OB² + OC² – OD² – OE² – OF² = AF² + BD² + CE²
(ii) AF² + BD² + CE² = AE² + CD² + BF²
Construction : Join OB, OC and OA.

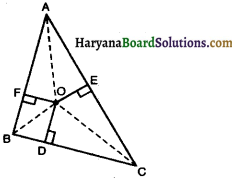
Proof :
(i) By Pythagoras theorem for each of the right triangles namely ∆OFA, ∆ODB, and ∆OEC, we get
OA² = OF² + AF² …………….(1)
OB² = OD² + BD² ……………(2)
OC² = OE² + CE² ……………..(3)
Adding (1), (2) and (3), we get
OA² + OB² + OC² = OF² + AF² + OD² + BD² + OE² + CE² = AF² + BD² + CE²
⇒ OA² + OB² + OC² – OD² – OE² – OF² = AF² + BD² + CE²
Hence Proved.

(ii) In right triangles ODB and ODC, we have
OB² = OD² + BD² …………….(4) [By Pythagoras theorem]
OC² = OD² + CD² ……………..(5)
Subtracting (5) from (4), we get
OB² – OC² = BD² – CD² ………….(6)
Similarly OC² – OA² = CE² – AE² ……………(7)
and OA² – OB² = AF² – BF² ……………(8)
Adding (6), (7) and (8), we get
OB² – OC² + OC² – OA² + OA² – OB² = BD² – CD² + CE² – AE² + AF² – BF²
⇒ 0 = BD² – CD² + CE² – AE² + AF² – BF²
⇒ AF² + BD² + CE² = AE² + CD² + BF²
Hence Proved.

Question 9.
A ladder 10 m long reaches a window 8m above the ground. Find the distance of the foot of the ladder from the base of the wall.
Solution :
Let AC be ladder and AB be wall and BC be the distance of the ladder from wall.

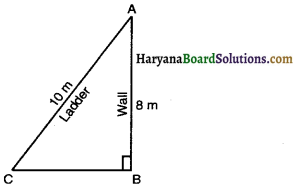
∴ AB = 8 m
AC = 10 m
In right ∆ABC, AC² = BC² + AB²
⇒ 10² = BC² + 8²
⇒ 100 = BC² + 64
⇒ BC² = 100 – 64
⇒ BC² = 36
⇒ BC = 6m.
Hence, distance of the foot of the ladder from base of the wall = 6m.

Question 10.
A guy wire attached to a vertical pole of height 18m is 24m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut ?
Solution :
Let AC be guy wire and AB be vertical pole and BC be distance of the stake from the base of the pole.
∴ AB = 18m, AC = 24m,
In right ∆ABC,
AC² = BC² + AB² [By Pythagoras theorem]
⇒ (24)² = BC² + (18)²
⇒ 576 = BC² + 324
⇒ BC² = 576 – 324

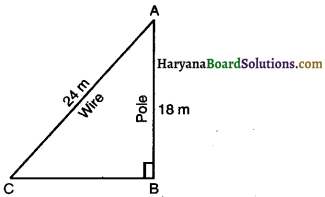
⇒ BC² = 252
⇒ BC = √252
⇒ BC = 6√7 m
Hence, distance of stake from the base of the pole = 6√7 m.

Question 11.
An aeroplane leaves an airport and flies due north at a speed of 1000 km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1\(\frac{1}{2}\)hours?
Solution:
Let point B represents the position of airport. Then distance covered by first plane in the north direction (AB) = Speed × Time
= 1000 × 1\(\frac{1}{2}\)
= 1000 × \(\frac{3}{2}\)
= 1500 km

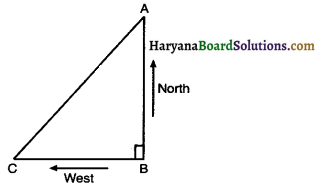
and distance covered by second plane in the west direction (BC) = Speed × Time
= 1200 × \(\frac{1}{2}\)
= 1200 × \(\frac{3}{2}\) = 1800 km.

Now, in right ∆ABC,
AC² = BC² + AB²
[By Pythagoras theorem]
⇒ AC² = (1800)² + (1500)²
⇒ AC² = 3240000 + 2250000
⇒ AC² = 5490000
⇒ AC = \(\sqrt{5490000}\)
⇒ AC = 300√61 km
Hence, distance between two planes = 300√61 km.

Question 12.
Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
Solution:
Let AB and CD be two poles, BD be distance between the feet of two poles and AC be distance between their tops.
∴ AB = 11 m, BD = 12 m, CD = 6 m
∠B = ∠D = 90°
CE || BD
∠AEC = 90°, CE = 12m, AE = 11 – 6 = 5 m
Now, in right ∆AEC,
∴ AC² = CE² + AE² [By Pythagoras theorem]

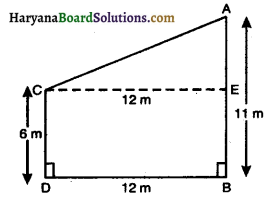
⇒ AC² = 12² + 5²
⇒ AC² = 144 + 25
⇒ AC² = 169
⇒ AC = √169
⇒ AC = 13 m.
Hence, distance between tops of two poles = 13 m.

Question 13.
D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE² + BD² = AB² + DE².
Solution :
Given : A triangle ABC in which ∠C = 90°, D and E are points on the sides CA and CB respectively.
To Prove : AE² + BD² = AB² + DE²
Proof: In right ∆ACE,
AE² = AC² + CE² ………………(1)
[By Pythagoras theorem]
In right ∆DCB,
BD² = BC² + DC² ………………(2)
In right ∆ABC,
AB² = BC² + AC² ……………….(3)
In right ∆DCE,
DE² = EC² + CD² ………………..(4)
Adding (1) and (2), we get

AE² + BD² = AC² + BC² + CE² + CD²
AE² + BD² = AB² + DE² [Using (3) and (4)]
Hence Proved.

Question 14.
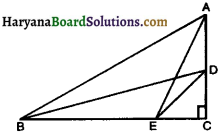
The perpendicular from A on side BC of a ∆ABC intersects BC at D such that DB = 3CD (see figure). Prove that 2AB² = 2AC² + BC².

Solution :
Given : A ∆ABC such that AD ⊥ BC and DB = 3CD
To Prove: 2AB² = 2AC² + BC²
Proof: DB = 3CD (given)
Now BC = DB + CD
⇒ BC = 3CD + CD [. DB = 3CD]
⇒ BC = 4CD
⇒ CD = \(\frac{1}{4}\)BC
⇒ and BD = 3CD = \(\frac{3}{4}\)BC
In right ∆ADB ,
AB² = BD² + AD² ………………..(1)
In right ∆ADC,
AC² = CD² + AD² …………………(2)
Subtracting (2) from (1), we get
AB² – AC² = BD² + AD² – CD² – AD²
⇒ AB² – AC² = (\(\frac{3}{4}\)BC)² – (\(\frac{1}{4}\)BC)²
[∵ BD = \(\frac{3}{4}\)BC and CD = \(\frac{1}{4}\)BC]
⇒ AB² – AC² = \(\frac{9}{16}\) BC² – \(\frac{9}{16}\) BC²
⇒ AB² – AC² = \(\frac{8}{16}\)BC²
⇒ AB² – AC² = \(\frac{1}{2}\)BC²
⇒ 2AB² – 2AC² = BC²
⇒ 2AB² = 2AC² + BC²
Hence Proved.

Question 15.
In an equilateral triangle ABC, D is a point on side BC such that BD = \(\frac{1}{3}\)BC. Prove that 9AD² = 7AB².
Solution :
Given : An equilateral ∆ABC such that BD = \(\frac{1}{3}\)BC.
To Prove: 9AD² = 7AB²
Construction : Draw AE ⊥ BC and Join AD.
Proof: Let each side of equilateral triangle be x units.

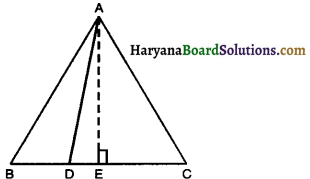
BD = \(\frac{1}{3}\)BC
⇒ BD = \(\frac{x}{3}\)
We know that in equi1ateral perpendicular bisects its corresponding opposite side
BE = EC = \(\frac{x}{2}\)
DE = BE – BD
DE = \(\frac{x}{2}\) – \(\frac{x}{3}\)
DE = \(\frac{x}{6}\)
In right ∆AED, AD² = AE² + DE² ……………..(1)
[By Pythagoras theorem]
In right ∆AEB, AB² = BE² + AE²
[By Pythagoras theorem]
AE² = AB² – BE²
Put AE² = AB² – BE² in (1), we get
AD² = 2 BE² + DE²
AD² = x² – (\(\frac{x}{2}\))² + (\(\frac{x}{6}\))²
⇒ AD² = x² – \(\frac{x^2}{4}+\frac{x^2}{36}\)
⇒ AD² = \(\frac{36 x^2-9 x^2+x^2}{36}\)
⇒ AD² = \(\frac{28 x^2}{36}\)
⇒ AD² = \(\frac{7}{9}\) x²
⇒ AD² = \(\frac{7}{9}\) AB²
⇒ 9AD² = 7AB²
Hence Proved.

Question 16.
In an equilateral triangle prove that three times the square of one side is equal to four times the square of one of its aftitudes.
Solution :
Given : A triangle ABC in which AB = BC = CA and AD ⊥ BC.
To Prove: 3AB² = 4AD²
Proof: In right ∆ADB and right ∆ADC.
AB = AC (Given)
∠ADB = ∠ADC (Each is 90°)

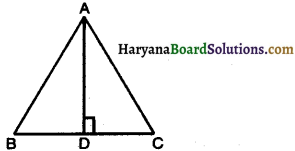
AD = AD (Common)
∴ ∆ADB ≅ ∆ADC (By RHS congruence criterion)
⇒ BD = CD (CPCT)
In right ∆ADB, AB² = AD² + BD² [By Pythagoras theorem]
⇒ AB² = AD² + (\(\frac{1}{2}\)AB)²
[∵ BD = CD
∴ BD = \(\frac{1}{2}\)AB]
⇒ AB² = 4AD² + \(\frac{1}{4}\)AB²
⇒ 4AB² = 4AD² + AB²
⇒ 3AB² = 4 AD²
Hence Proved.

Question 17.
Tick the correct answer and justify. In ∆ABC, AB = 6√3 cm, AC = 12 cm and BC = 6 cm. The angle B is :
(A) 120°
(B) 60°
(C) 90°
(D) 45°
Solution :
In ∆ABC,
AC² = 12²
⇒ AC² = 144
BC² + AB² = (6)² + (6√3)²
⇒ BC² + AB² = 36 + 108
⇒ BC² + AB² = 144
∴ AC² = BC² + AB²

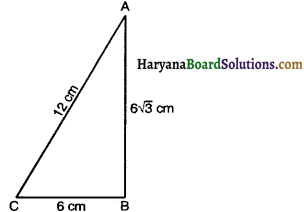
By converse of Pythagoras theorem,
∠B = 90°
Correct answer = (C)
Solution is also justified.